- Page 2:
This page intentionally left blank
- Page 8:
Computability and Logic Fifth Editi
- Page 12:
For SALLY and AIGLI and EDITH
- Page 18:
viii CONTENTS BASIC METALOGIC 9 APr
- Page 24:
Preface to the Fifth Edition The or
- Page 28:
PREFACE TO THE FIFTH EDITION xiii T
- Page 36:
1 Enumerability Our ultimate goal w
- Page 40:
1.1. ENUMERABILITY 5 we might have
- Page 44:
1.2. ENUMERABLE SETS 7 member of A,
- Page 48:
1.2. ENUMERABLE SETS 9 The pair (m,
- Page 52:
1.2. ENUMERABLE SETS 11 a pair G(n)
- Page 56:
1.2. ENUMERABLE SETS 13 on Example
- Page 60:
PROBLEMS 15 the set of all positive
- Page 64:
DIAGONALIZATION 17 supposition must
- Page 68:
DIAGONALIZATION 19 has that much ti
- Page 72:
PROBLEMS 21 2.4 Show that the set o
- Page 76:
3 Turing Computability A function i
- Page 80:
TURING COMPUTABILITY 25 carried out
- Page 84:
TURING COMPUTABILITY 27 in general
- Page 88:
TURING COMPUTABILITY 29 It will the
- Page 92:
TURING COMPUTABILITY 31 But if ther
- Page 96:
TURING COMPUTABILITY 33 A numerical
- Page 100:
4 Uncomputability In the preceding
- Page 104:
4.1. THE HALTING PROBLEM 37 machine
- Page 108:
4.1. THE HALTING PROBLEM 39 Turing
- Page 112:
4.2. THE PRODUCTIVITY FUNCTION 41 g
- Page 116:
4.2. THE PRODUCTIVITY FUNCTION 43 F
- Page 120:
5 Abacus Computability Showing that
- Page 124:
5.1. ABACUS MACHINES 47 be thought
- Page 128:
5.1. ABACUS MACHINES 49 Figure 5-4.
- Page 132:
5.2. SIMULATING ABACUS MACHINES BY
- Page 136:
5.2. SIMULATING ABACUS MACHINES BY
- Page 140:
5.2. SIMULATING ABACUS MACHINES BY
- Page 144:
5.3. THE SCOPE OF ABACUS COMPUTABIL
- Page 148:
5.3. THE SCOPE OF ABACUS COMPUTABIL
- Page 152:
PROBLEMS 61 Figure 5-17. Minimizati
- Page 156:
6 Recursive Functions The intuitive
- Page 160:
One might indicate this in shorthan
- Page 164:
6.1. PRIMITIVE RECURSIVE FUNCTIONS
- Page 168:
6.1. PRIMITIVE RECURSIVE FUNCTIONS
- Page 172:
PROBLEMS 71 The total function f is
- Page 176:
7 Recursive Sets and Relations In t
- Page 180:
7.1. RECURSIVE RELATIONS 75 Given a
- Page 184:
7.1. RECURSIVE RELATIONS 77 Proof:
- Page 188:
7.1. RECURSIVE RELATIONS 79 So the
- Page 192:
answer. Thus if S is a semidecidabl
- Page 196:
7.3. FURTHER EXAMPLES 83 Proof: Sup
- Page 200:
7.3. FURTHER EXAMPLES 85 after whic
- Page 204:
PROBLEMS 87 7.9 Let f (n) bethenth
- Page 208:
8.1. CODING TURING COMPUTATIONS 89
- Page 212:
8.1. CODING TURING COMPUTATIONS 91
- Page 216:
8.1. CODING TURING COMPUTATIONS 93
- Page 220:
8.2. UNIVERSAL TURING MACHINES 95 P
- Page 224:
PROBLEMS 97 relation of the restric
- Page 228:
Basic Metalogic
- Page 234:
102 APRÉCIS OF FIRST-ORDER LOGIC:
- Page 238:
104 APRÉCIS OF FIRST-ORDER LOGIC:
- Page 242:
106 APRÉCIS OF FIRST-ORDER LOGIC:
- Page 246:
108 APRÉCIS OF FIRST-ORDER LOGIC:
- Page 250:
110 APRÉCIS OF FIRST-ORDER LOGIC:
- Page 254:
112 APRÉCIS OF FIRST-ORDER LOGIC:
- Page 258:
10 APrécis of First-Order Logic: S
- Page 262:
116 APRÉCIS OF FIRST-ORDER LOGIC:
- Page 266:
118 APRÉCIS OF FIRST-ORDER LOGIC:
- Page 270:
120 APRÉCIS OF FIRST-ORDER LOGIC:
- Page 274:
122 APRÉCIS OF FIRST-ORDER LOGIC:
- Page 278:
124 APRÉCIS OF FIRST-ORDER LOGIC:
- Page 282:
11 The Undecidability of First-Orde
- Page 286:
128 THE UNDECIDABILITY OF FIRST-ORD
- Page 290:
130 THE UNDECIDABILITY OF FIRST-ORD
- Page 294:
132 THE UNDECIDABILITY OF FIRST-ORD
- Page 298:
134 THE UNDECIDABILITY OF FIRST-ORD
- Page 302:
136 THE UNDECIDABILITY OF FIRST-ORD
- Page 306:
138 MODELS There are actually sever
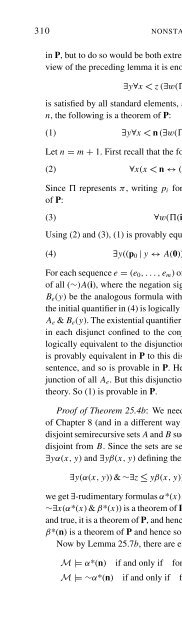
- Page 310:
140 MODELS If we also let 0 denote
- Page 314:
142 MODELS But this is simply the c
- Page 318:
144 MODELS the number of equivalenc
- Page 322:
146 MODELS elements are isolated, a
- Page 326:
148 MODELS (b) Any set of sentences
- Page 330:
150 MODELS vertices of a square, an
- Page 334:
152 MODELS Such a model A will cons
- Page 338:
154 THE EXISTENCE OF MODELS (S7) If
- Page 342:
156 THE EXISTENCE OF MODELS 13.6 Le
- Page 346:
158 THE EXISTENCE OF MODELS (E3) If
- Page 350:
160 THE EXISTENCE OF MODELS which i
- Page 354:
162 THE EXISTENCE OF MODELS And ind
- Page 358:
164 THE EXISTENCE OF MODELS Problem
- Page 362:
14 Proofs and Completeness Introduc
- Page 366:
168 PROOFS AND COMPLETENESS true.
- Page 370:
170 PROOFS AND COMPLETENESS Table 1
- Page 374:
172 PROOFS AND COMPLETENESS is beca
- Page 378:
174 PROOFS AND COMPLETENESS 14.13 E
- Page 382:
176 PROOFS AND COMPLETENESS new int
- Page 386:
178 PROOFS AND COMPLETENESS whether
- Page 390:
180 PROOFS AND COMPLETENESS possibl
- Page 394:
182 PROOFS AND COMPLETENESS Proofs:
- Page 398:
184 PROOFS AND COMPLETENESS In addi
- Page 402:
186 PROOFS AND COMPLETENESS Unless
- Page 406:
188 ARITHMETIZATION in which, as el
- Page 410:
190 ARITHMETIZATION 15.5 Corollary
- Page 414:
192 ARITHMETIZATION 15.7 Corollary.
- Page 418:
194 ARITHMETIZATION This means that
- Page 422:
196 ARITHMETIZATION For the first c
- Page 426:
198 ARITHMETIZATION 15.2 Let Ɣ be
- Page 430:
200 REPRESENTABILITY OF RECURSIVE F
- Page 434:
202 REPRESENTABILITY OF RECURSIVE F
- Page 438:
204 REPRESENTABILITY OF RECURSIVE F
- Page 442:
206 REPRESENTABILITY OF RECURSIVE F
- Page 446:
208 REPRESENTABILITY OF RECURSIVE F
- Page 450:
210 REPRESENTABILITY OF RECURSIVE F
- Page 454:
212 REPRESENTABILITY OF RECURSIVE F
- Page 458:
214 REPRESENTABILITY OF RECURSIVE F
- Page 462:
216 REPRESENTABILITY OF RECURSIVE F
- Page 466:
218 REPRESENTABILITY OF RECURSIVE F
- Page 470:
17 Indefinability, Undecidability,
- Page 474:
222 INDEFINABILITY, UNDECIDABILITY,
- Page 478:
224 INDEFINABILITY, UNDECIDABILITY,
- Page 482:
226 INDEFINABILITY, UNDECIDABILITY,
- Page 486:
228 INDEFINABILITY, UNDECIDABILITY,
- Page 490:
230 INDEFINABILITY, UNDECIDABILITY,
- Page 494:
18 The Unprovability of Consistency
- Page 498:
234 THE UNPROVABILITY OF CONSISTENC
- Page 502:
236 THE UNPROVABILITY OF CONSISTENC
- Page 506:
238 THE UNPROVABILITY OF CONSISTENC
- Page 512:
Further Topics
- Page 518:
244 NORMAL FORMS Proof: The proof i
- Page 522:
246 NORMAL FORMS 19.3 Theorem (Full
- Page 526:
248 NORMAL FORMS For (1) and (3.1)
- Page 530:
250 NORMAL FORMS can be the domains
- Page 534:
252 NORMAL FORMS as ‘set (of natu
- Page 538:
254 NORMAL FORMS Now if Ɣ is satis
- Page 542:
256 NORMAL FORMS in a sense to be m
- Page 546:
258 NORMAL FORMS if P A holds of a
- Page 550:
20 The Craig Interpolation Theorem
- Page 554:
262 THE CRAIG INTERPOLATION THEOREM
- Page 558:
264 THE CRAIG INTERPOLATION THEOREM
- Page 562:
266 THE CRAIG INTERPOLATION THEOREM
- Page 566:
268 THE CRAIG INTERPOLATION THEOREM
- Page 570:
21 Monadic and Dyadic Logic We have
- Page 574:
272 MONADIC AND DYADIC LOGIC associ
- Page 578:
274 MONADIC AND DYADIC LOGIC Our in
- Page 582:
276 MONADIC AND DYADIC LOGIC For th
- Page 586:
278 MONADIC AND DYADIC LOGIC Svu is
- Page 590:
280 SECOND-ORDER LOGIC just sets, o
- Page 594:
282 SECOND-ORDER LOGIC is true. But
- Page 598: 284 SECOND-ORDER LOGIC Proof: A fir
- Page 602: 23 Arithmetical Definability Tarski
- Page 606: 288 ARITHMETICAL DEFINABILITY Proof
- Page 610: 290 ARITHMETICAL DEFINABILITY 23.4
- Page 614: 292 ARITHMETICAL DEFINABILITY Case
- Page 618: 294 ARITHMETICAL DEFINABILITY A-cor
- Page 622: 296 DECIDABILITY OF ARITHMETIC WITH
- Page 626: 298 DECIDABILITY OF ARITHMETIC WITH
- Page 630: 300 DECIDABILITY OF ARITHMETIC WITH
- Page 634: 25 Nonstandard Models By a model of
- Page 638: 304 NONSTANDARD MODELS Note that a
- Page 642: 306 NONSTANDARD MODELS the proof of
- Page 646: 308 NONSTANDARD MODELS Before givin
- Page 652: 25.2. OPERATIONS IN NONSTANDARD MOD
- Page 656: 25.3. NONSTANDARD MODELS OF ANALYSI
- Page 660: 25.3. NONSTANDARD MODELS OF ANALYSI
- Page 664: PROBLEMS 317 (In general, there cou
- Page 668: 26 Ramsey’s Theorem Ramsey’s th
- Page 672: 26.1. RAMSEY’S THEOREM: FINITARY
- Page 676: 26.2. K ÖNIG’S LEMMA 323 called
- Page 680: 26.2. K ÖNIG’S LEMMA 325 The pre
- Page 684: 27 Modal Logic and Provability Moda
- Page 688: 27.1. MODAL LOGIC 329 There is a no
- Page 692: 27.1. MODAL LOGIC 331 deducible fro
- Page 696: 27.1. MODAL LOGIC 333 For Propositi
- Page 700:
27.2. THE LOGIC OF PROVABILITY 335
- Page 704:
27.3. THE FIXED POINT AND NORMAL FO
- Page 708:
27.3. THE FIXED POINT AND NORMAL FO
- Page 712:
Annotated Bibliography General Refe
- Page 718:
344 INDEX categorical theory, see d
- Page 722:
346 INDEX Gödel sentence, 225 Göd
- Page 726:
348 INDEX Presburger, Max, see arit
- Page 730:
350 INDEX valid sentence, 120, 327