The Computable Differential Equation Lecture ... - Bruce E. Shapiro

40 CHAPTER 2. SUCCESSIVE APPROXIMATIONS
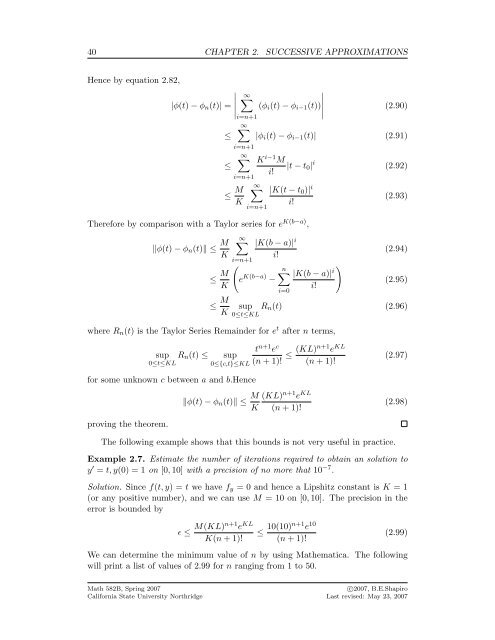
Hence by equation 2.82,
|φ(t) − φ n (t)| =
∣
≤
≤
≤ M K
∞∑
i=n+1
∞∑
i=n+1
∞∑
i=n+1
(φ i (t) − φ i−1 (t))
∣
(2.90)
|φ i (t) − φ i−1 (t)| (2.91)
K i−1 M
|t − t 0 | i (2.92)
i!
∞∑
i=n+1
|K(t − t 0 )| i
Therefore by comparison with a Taylor series for e K(b−a) ,
i!
(2.93)
‖φ(t) − φ n (t)‖ ≤ M K
≤ M K
∞∑ |K(b − a)| i
i!
i=n+1
(
)
n∑
e K(b−a) |K(b − a)| i
−
i!
i=0
(2.94)
(2.95)
≤ M K
sup R n (t) (2.96)
0≤t≤KL
where R n (t) is the Taylor Series Remainder for e t after n terms,
sup R n (t) ≤
0≤t≤KL
sup
0≤{c,t}≤KL
t n+1 e c
(n + 1)! ≤ (KL)n+1 e KL
(n + 1)!
(2.97)
for some unknown c between a and b.Hence
proving the theorem.
‖φ(t) − φ n (t)‖ ≤ M K
(KL) n+1 e KL
(n + 1)!
(2.98)
The following example shows that this bounds is not very useful in practice.
Example 2.7. Estimate the number of iterations required to obtain an solution to
y ′ = t, y(0) = 1 on [0, 10] with a precision of no more that 10 −7 .
Solution. Since f(t, y) = t we have f y = 0 and hence a Lipshitz constant is K = 1
(or any positive number), and we can use M = 10 on [0, 10]. The precision in the
error is bounded by
ɛ ≤ M(KL)n+1 e KL
K(n + 1)!
≤ 10(10)n+1 e 10
(n + 1)!
(2.99)
We can determine the minimum value of n by using Mathematica. The following
will print a list of values of 2.99 for n ranging from 1 to 50.
Math 582B, Spring 2007
California State University Northridge
c○2007, B.E.Shapiro
Last revised: May 23, 2007