Trigonometric Integrals - Bruce E. Shapiro
Trigonometric Integrals - Bruce E. Shapiro Trigonometric Integrals - Bruce E. Shapiro
Math 150A TOPIC 7. TRIGONOMETRIC INTEGRALS ∫ Example 7.5 sin 5 x cos 2 x dx ∫ ∫ sin 5 x cos 2 x dx = sin x(sin 2 x) 2 cos 2 x (7.17a) ∫ = sin x(1 − cos 2 x) 2 cos 2 x dx (7.17b) = − ∫ (1 − u 2 ) 2 u 2 du with u = cos x (7.17c) = − ∫ (u 2 − 2u 4 + u 6 ) du (7.17d) = − 1 3 u3 + 2 5 u5 − 1 7 u7 + C (7.17e) = − 1 3 cos3 x + 2 5 cos5 x − 1 7 cos7 x + C (7.17f) ∫ Example 7.6 sin 4 x cos 2 x dx ∫ ∫ sin 4 x cos 2 x dx = ⎛ ⎞ sin 2 x { }} { 1 ⎜ ⎝2 (1 − cos(2x)) ⎟ ⎠ 2 ( 1 2 (1 + cos(2x)) ) dx (7.18a) ∫ (1 − cos(2x) − cos 2 (2x) + cos 3 (2x) ) dx (7.18b) = 1 8 = x 8 − 1 16 sin(2x) − 1 8 ∫ cos 2 (2x) + 1 8 ∫ cos 3 (2x) dx (7.18c) From exercise 7.1 we have ∫ cos 2 xdx = x 2 + 1 4 sin(2x) (7.18d) and from example 7.2 ∫ cos 3 xdx = sin x − 1 3 sin3 x (7.18e) Page 24 « 2012. Last revised: February 26, 2013
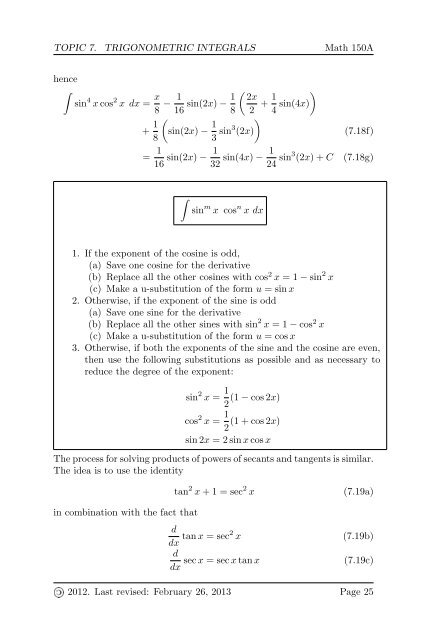
TOPIC 7. TRIGONOMETRIC INTEGRALS Math 150A hence ∫ sin 4 x cos 2 x dx = x 8 − 1 16 sin(2x) − 1 ( 2x 8 2 + 1 ) 4 sin(4x) + 1 ( sin(2x) − 1 ) 8 3 sin3 (2x) (7.18f) = 1 16 sin(2x) − 1 32 sin(4x) − 1 24 sin3 (2x) + C (7.18g) ∫ sin m x cos n x dx 1. If the exponent of the cosine is odd, (a) Save one cosine for the derivative (b) Replace all the other cosines with cos 2 x = 1 − sin 2 x (c) Make a u-substitution of the form u = sin x 2. Otherwise, if the exponent of the sine is odd (a) Save one sine for the derivative (b) Replace all the other sines with sin 2 x = 1 − cos 2 x (c) Make a u-substitution of the form u = cos x 3. Otherwise, if both the exponents of the sine and the cosine are even, then use the following substitutions as possible and as necessary to reduce the degree of the exponent: sin 2 x = 1 (1 − cos 2x) 2 cos 2 x = 1 (1 + cos 2x) 2 sin 2x = 2 sin x cos x The process for solving products of powers of secants and tangents is similar. The idea is to use the identity in combination with the fact that tan 2 x + 1 = sec 2 x d dx tan x = sec2 x d sec x = sec x tan x dx (7.19c) (7.19a) (7.19b) « 2012. Last revised: February 26, 2013 Page 25
- Page 1 and 2: Topic 7 Trigonometric Integrals In
- Page 3: TOPIC 7. TRIGONOMETRIC INTEGRALS Ma
- Page 7 and 8: TOPIC 7. TRIGONOMETRIC INTEGRALS Ma
- Page 9 and 10: TOPIC 7. TRIGONOMETRIC INTEGRALS Ma
TOPIC 7. TRIGONOMETRIC INTEGRALS Math 150A<br />
hence<br />
∫<br />
sin 4 x cos 2 x dx = x 8 − 1<br />
16 sin(2x) − 1 ( 2x<br />
8 2 + 1 )<br />
4 sin(4x)<br />
+ 1 (<br />
sin(2x) − 1 )<br />
8<br />
3 sin3 (2x)<br />
(7.18f)<br />
= 1 16 sin(2x) − 1<br />
32 sin(4x) − 1 24 sin3 (2x) + C (7.18g)<br />
∫<br />
sin m x cos n x dx<br />
1. If the exponent of the cosine is odd,<br />
(a) Save one cosine for the derivative<br />
(b) Replace all the other cosines with cos 2 x = 1 − sin 2 x<br />
(c) Make a u-substitution of the form u = sin x<br />
2. Otherwise, if the exponent of the sine is odd<br />
(a) Save one sine for the derivative<br />
(b) Replace all the other sines with sin 2 x = 1 − cos 2 x<br />
(c) Make a u-substitution of the form u = cos x<br />
3. Otherwise, if both the exponents of the sine and the cosine are even,<br />
then use the following substitutions as possible and as necessary to<br />
reduce the degree of the exponent:<br />
sin 2 x = 1 (1 − cos 2x)<br />
2<br />
cos 2 x = 1 (1 + cos 2x)<br />
2<br />
sin 2x = 2 sin x cos x<br />
The process for solving products of powers of secants and tangents is similar.<br />
The idea is to use the identity<br />
in combination with the fact that<br />
tan 2 x + 1 = sec 2 x<br />
d<br />
dx tan x = sec2 x<br />
d<br />
sec x = sec x tan x<br />
dx (7.19c)<br />
(7.19a)<br />
(7.19b)<br />
« 2012. Last revised: February 26, 2013 Page 25