Multivariate Calculus - Bruce E. Shapiro

LECTURE 11. GRADIENTS AND THE DIRECTIONAL DERIVATIVE 77
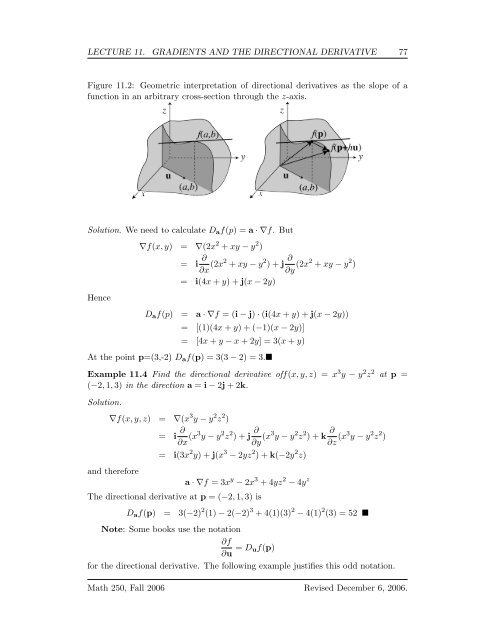
Figure 11.2: Geometric interpretation of directional derivatives as the slope of a
function in an arbitrary cross-section through the z-axis.
Solution. We need to calculate D a f(p) = a · ∇f. But
Hence
∇f(x, y) = ∇(2x 2 + xy − y 2 )
= i ∂
∂x (2x2 + xy − y 2 ) + j ∂ ∂y (2x2 + xy − y 2 )
= i(4x + y) + j(x − 2y)
D a f(p) = a · ∇f = (i − j) · (i(4x + y) + j(x − 2y))
= [(1)(4x + y) + (−1)(x − 2y)]
= [4x + y − x + 2y] = 3(x + y)
At the point p=(3,-2) D a f(p) = 3(3 − 2) = 3.
Example 11.4 Find the directional derivative off(x, y, z) = x 3 y − y 2 z 2 at p =
(−2, 1, 3) in the direction a = i − 2j + 2k.
Solution.
∇f(x, y, z) = ∇(x 3 y − y 2 z 2 )
= i ∂
∂x (x3 y − y 2 z 2 ) + j ∂ ∂y (x3 y − y 2 z 2 ) + k ∂ ∂z (x3 y − y 2 z 2 )
and therefore
= i(3x 2 y) + j(x 3 − 2yz 2 ) + k(−2y 2 z)
a · ∇f = 3x y − 2x 3 + 4yz 2 − 4y z
The directional derivative at p = (−2, 1, 3) is
D a f(p) = 3(−2) 2 (1) − 2(−2) 3 + 4(1)(3) 2 − 4(1) 2 (3) = 52
Note: Some books use the notation
∂f
∂u = D uf(p)
for the directional derivative. The following example justifies this odd notation.
Math 250, Fall 2006 Revised December 6, 2006.