Multivariate Calculus - Bruce E. Shapiro

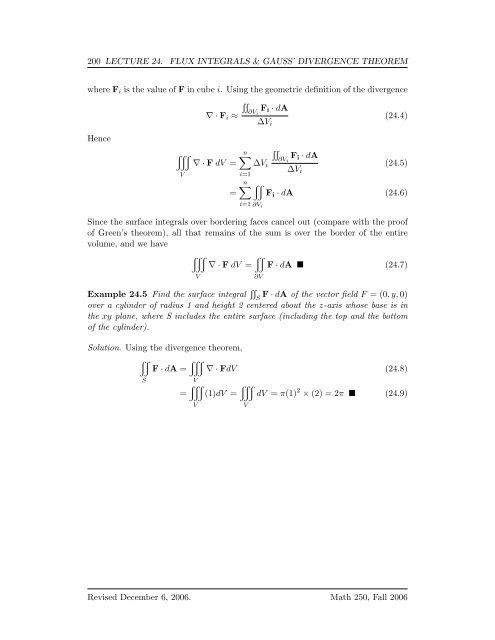
200 LECTURE 24. FLUX INTEGRALS & GAUSS’ DIVERGENCE THEOREM
where F i is the value of F in cube i. Using the geometric definition of the divergence
∬
∂V
∇ · F i ≈ i
F i · dA
(24.4)
∆V i
Hence
V
∇ · F dV =
=
n∑
i=1
n∑
i=1
∆V i
∬
∂V i
F i · dA
∆V i
(24.5)
∂V i
F i · dA (24.6)
Since the surface integrals over bordering faces cancel out (compare with the proof
of Green’s theorem), all that remains of the sum is over the border of the entire
volume, and we have
∇ · F dV = F · dA (24.7)
V
Example 24.5 Find the surface integral ∬ S
F · dA of the vector field F = (0, y, 0)
over a cylinder of radius 1 and height 2 centered about the z-axis whose base is in
the xy plane, where S includes the entire surface (including the top and the bottom
of the cylinder).
Solution. Using the divergence theorem,
F · dA = ∇ · FdV (24.8)
S
V
∂V
= (1)dV = dV = π(1) 2 × (2) = 2π (24.9)
V
V
Revised December 6, 2006. Math 250, Fall 2006