Multivariate Calculus - Bruce E. Shapiro

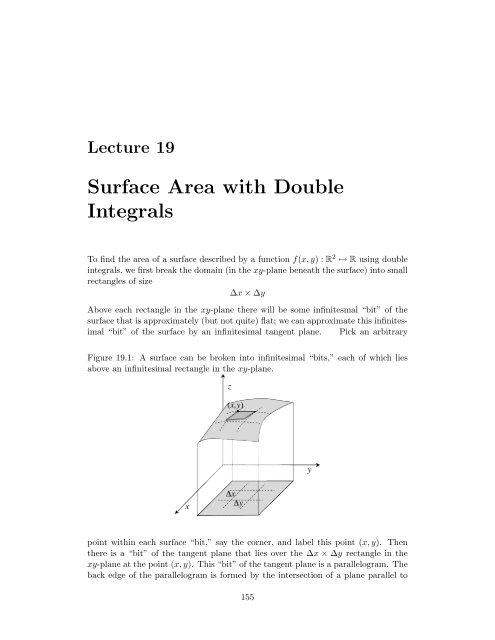
Lecture 19
Surface Area with Double
Integrals
To find the area of a surface described by a function f(x, y) : R 2 ↦→ R using double
integrals, we first break the domain (in the xy-plane beneath the surface) into small
rectangles of size
∆x × ∆y
Above each rectangle in the xy-plane there will be some infinitesmal “bit” of the
surface that is approximately (but not quite) flat; we can approximate this infinitesimal
“bit” of the surface by an infinitesimal tangent plane. Pick an arbitrary
Figure 19.1: A surface can be broken into infinitesimal “bits,” each of which lies
above an infinitesimal rectangle in the xy-plane.
point within each surface “bit,” say the corner, and label this point (x, y). Then
there is a “bit” of the tangent plane that lies over the ∆x × ∆y rectangle in the
xy-plane at the point (x, y). This “bit” of the tangent plane is a parallelogram. The
back edge of the parallelogram is formed by the intersection of a plane parallel to
155