Multivariate Calculus - Bruce E. Shapiro

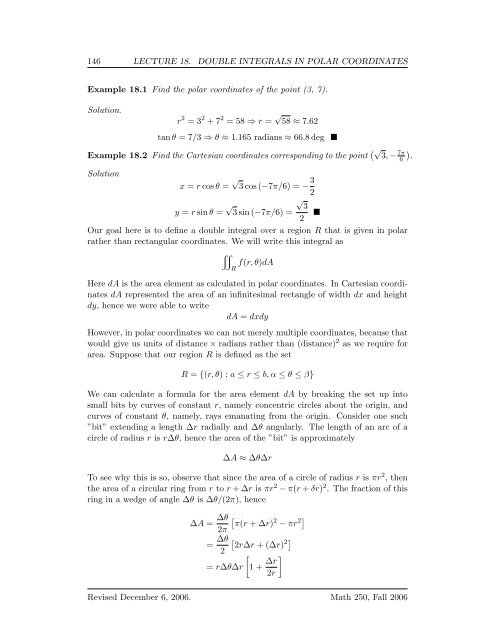
146 LECTURE 18. DOUBLE INTEGRALS IN POLAR COORDINATES
Example 18.1 Find the polar coordinates of the point (3, 7).
Solution.
r 2 = 3 2 + 7 2 = 58 ⇒ r = √ 58 ≈ 7.62
tan θ = 7/3 ⇒ θ ≈ 1.165 radians ≈ 66.8 deg
Example 18.2 Find the Cartesian coordinates corresponding to the point (√ 3, − 7π 6
Solution
x = r cos θ = √ 3 cos (−7π/6) = − 3 2
y = r sin θ = √ √
3
3 sin (−7π/6) =
2
Our goal here is to define a double integral over a region R that is given in polar
rather than rectangular coordinates. We will write this integral as
f(r, θ)dA
R
Here dA is the area element as calculated in polar coordinates. In Cartesian coordinates
dA represented the area of an infinitesimal rectangle of width dx and height
dy, hence we were able to write
dA = dxdy
However, in polar coordinates we can not merely multiple coordinates, because that
would give us units of distance × radians rather than (distance) 2 as we require for
area. Suppose that our region R is defined as the set
R = {(r, θ) : a ≤ r ≤ b, α ≤ θ ≤ β}
We can calculate a formula for the area element dA by breaking the set up into
small bits by curves of constant r, namely concentric circles about the origin, and
curves of constant θ, namely, rays emanating from the origin. Consider one such
”bit” extending a length ∆r radially and ∆θ angularly. The length of an arc of a
circle of radius r is r∆θ, hence the area of the ”bit” is approximately
∆A ≈ ∆θ∆r
To see why this is so, observe that since the area of a circle of radius r is πr 2 , then
the area of a circular ring from r to r + ∆r is πr 2 − π(r + δr) 2 . The fraction of this
ring in a wedge of angle ∆θ is ∆θ/(2π), hence
∆A = ∆θ
2π
= ∆θ
2
= r∆θ∆r
[
π(r + ∆r) 2 − πr 2]
[
2r∆r + (∆r)
2 ]
[
1 + ∆r ]
2r
Revised December 6, 2006. Math 250, Fall 2006
)
.