Multivariate Calculus - Bruce E. Shapiro

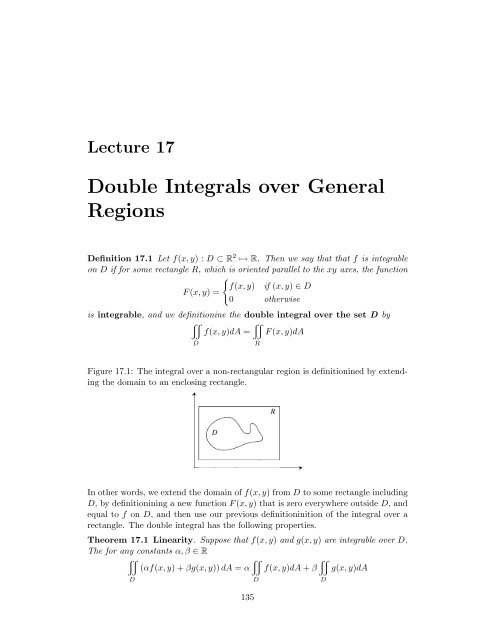
Lecture 17
Double Integrals over General
Regions
Definition 17.1 Let f(x, y) : D ⊂ R 2 ↦→ R. Then we say that that f is integrable
on D if for some rectangle R, which is oriented parallel to the xy axes, the function
{
f(x, y) if (x, y) ∈ D
F (x, y) =
0 otherwise
is integrable, and we definitionine the double integral over the set D by
f(x, y)dA = F (x, y)dA
D
R
Figure 17.1: The integral over a non-rectangular region is definitionined by extending
the domain to an enclosing rectangle.
In other words, we extend the domain of f(x, y) from D to some rectangle including
D, by definitionining a new function F (x, y) that is zero everywhere outside D, and
equal to f on D, and then use our previous definitioninition of the integral over a
rectangle. The double integral has the following properties.
Theorem 17.1 Linearity. Suppose that f(x, y) and g(x, y) are integrable over D.
The for any constants α, β ∈ R
(αf(x, y) + βg(x, y)) dA = α f(x, y)dA + β g(x, y)dA
D
135
D
D