Lecture Notes in Differential Equations - Bruce E. Shapiro

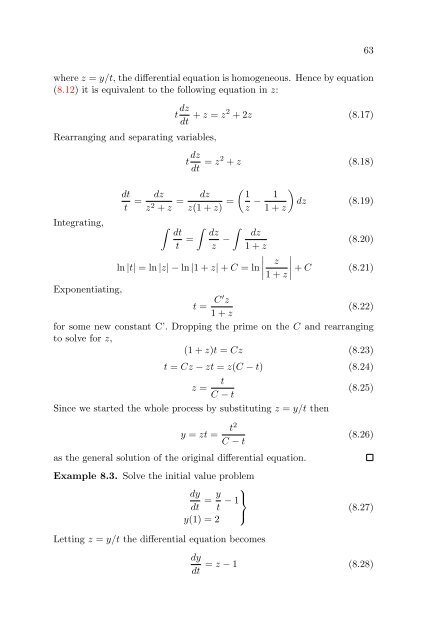
63
where z = y/t, the differential equation is homogeneous. Hence by equation
(8.12) it is equivalent to the following equation in z:
Rearranging and separating variables,
Integrating,
Exponentiating,
dt
t =
dz
z 2 + z =
t dz
dt + z = z2 + 2z (8.17)
t dz
dt = z2 + z (8.18)
(
dz 1
z(1 + z) = z − 1 )
dz (8.19)
1 + z
∫ ∫ ∫
dt dz
t = z − dz
(8.20)
1 + z
ln |t| = ln |z| − ln |1 + z| + C = ln
z
∣1 + z ∣ + C (8.21)
t = C′ z
(8.22)
1 + z
for some new constant C’. Dropping the prime on the C and rearranging
to solve for z,
(1 + z)t = Cz (8.23)
t = Cz − zt = z(C − t) (8.24)
z =
t
C − t
Since we started the whole process by substituting z = y/t then
(8.25)
y = zt =
t2
C − t
as the general solution of the original differential equation.
Example 8.3. Solve the initial value problem
dy
dt = y ⎫
t − 1 ⎬
⎭
y(1) = 2
(8.26)
(8.27)
Letting z = y/t the differential equation becomes
dy
dt = z − 1 (8.28)