Lecture Notes in Differential Equations - Bruce E. Shapiro
Lecture Notes in Differential Equations - Bruce E. Shapiro Lecture Notes in Differential Equations - Bruce E. Shapiro
248 LESSON 27. HIGHER ORDER EQUATIONS where min(m ij ) is the minor of the ij th element. Differentiating, dM dt n+1 ∑ n+1 ∑ = (−1) 1+i m ′ 1i min(m 1i ) + (−1) 1+i d m 1i dt min(m 1i) (27.170) i=1 i=1 The first sum is d(M,1). Since (27.166) is true for any n × n matrix, we can apply it to min(m 1i ) in the second sum. dM dt = d(M, 1) + ∑ n+1 i=1 (−1)1+i m 1i ∑ n j=1 d(min(m 1i), j) (27.171) which completes the inductive proof of the lemma. Proof. (Abel’s Formula) (n = 2). Suppose y 1 and y 2 are solutions of (27.165). Their Wronskian is Differentiating, W (t) = y 1 y ′ 2 − y 2 y ′ 1 (27.172) W ′ (x) = y 1 y ′′ 2 + y ′ 1y ′ 2 − y ′ 2y ′ 1 − y 2 y ′′ 1 = y 1 y ′′ 2 − y 2 y ′′ 1 (27.173) Since L 2 y 1 = L 2 y 2 = 0, Hence y ′′ 1 = −p(t)y ′ 1 − q(t)y 1 (27.174) y ′′ 2 = −p(t)y ′ 2 − q(t)y 2 (27.175) W ′ (t) = y 1 (−p(t)y 2 ′ − q(t)y 2 ) − y 2 (−p(t)y 1 ′ − q(t)y 1 ) = −p(t)(y 1 y 2 ′ − y 2 y 1) ′ = −p(t)W (t) Rearranging and integrating gives W (t) = C exp [ − ∫ p(t)dt ] . General Case. The Wronskian is W [y 1 , ..., y n ](t) = ∣ y 1 · · · y n . . y (n−1) 1 · · · y n (n−1) ∣ (27.176) (27.177)
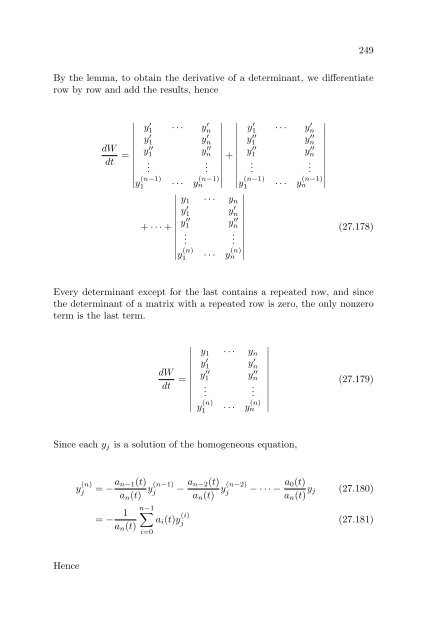
249 By the lemma, to obtain the derivative of a determinant, we differentiate row by row and add the results, hence dW dt = ∣ y 1 ′ · · · y n ′ y 1 ′ y n ′ y 1 ′ · · · y ′ n y 1 ′′ y n ′′ y 1 ′′ y ′′ n + y 1 ′′ y ′′ n . . . . 1 · · · y n (n−1) ∣ ∣y (n−1) 1 · · · y n (n−1) ∣ y 1 · · · y n y 1 ′ y ′ n + · · · + y 1 ′′ y n ′′ . . ∣y (n) 1 · · · y n (n) ∣ y (n−1) (27.178) Every determinant except for the last contains a repeated row, and since the determinant of a matrix with a repeated row is zero, the only nonzero term is the last term. dW dt = ∣ y 1 · · · y n y ′ 1 y ′ n y 1 ′′ y n ′′ . . y (n) 1 · · · y n (n) ∣ (27.179) Since each y j is a solution of the homogeneous equation, y (n) j = − a n−1(t) a n (t) y(n−1) j − a n−2(t) a n (t) y(n−2) j − · · · − a 0(t) a n (t) y j (27.180) = − 1 n−1 ∑ a i (t)y (i) j (27.181) a n (t) i=0 Hence
- Page 205 and 206: 197 The characteristic equation is
- Page 207 and 208: Lesson 23 Method of Annihilators In
- Page 209 and 210: 201 Theorem 23.5. (D 2 − 2aD + (a
- Page 211 and 212: 203 The method of annihilators is r
- Page 213 and 214: Lesson 24 Variation of Parameters T
- Page 215 and 216: 207 Substituting into equation (24.
- Page 217 and 218: 209 Example 24.3. Solve the initial
- Page 219 and 220: Lesson 25 Harmonic Oscillations If
- Page 221 and 222: 213 It is standard to define a new
- Page 223 and 224: 215 As with the unforced case, we c
- Page 225 and 226: Lesson 26 General Existence Theory*
- Page 227 and 228: 219 In the case just proven, there
- Page 229 and 230: 221 Theorem 26.5. Under the same co
- Page 231 and 232: 223 Since K n /(1 − K) → 0 as n
- Page 233 and 234: 225 for any φ ∈ V. Let g, h be f
- Page 235 and 236: Lesson 27 Higher Order Linear Equat
- Page 237 and 238: 229 L n+1 (e rt y) = e rt a n (D +
- Page 239 and 240: 231 Example 27.2. Find the general
- Page 241 and 242: 233 Differentiating, u ′ (t) = d
- Page 243 and 244: 235 Integrating, − 2K |t − t 0
- Page 245 and 246: 237 a closed form expression for a
- Page 247 and 248: 239 Example 27.6. Find the general
- Page 249 and 250: 241 The characteristic equation is
- Page 251 and 252: 243 The Wronskian In this section w
- Page 253 and 254: 245 Certainly every φ(t) given by
- Page 255: 247 the differential equation. Over
- Page 259 and 260: 251 Example 27.14. Find the general
- Page 261 and 262: 253 So that f(t) = a n (t)y[ (n) +
- Page 263 and 264: Lesson 28 Series Solutions In many
- Page 265 and 266: 257 Changing the index of the secon
- Page 267 and 268: 259 Since the first two terms (corr
- Page 269 and 270: 261 Hence ∞∑ ∞∑ ∞∑ 0 =
- Page 271 and 272: 263 has an analytic solution at t =
- Page 273 and 274: 265 By the triangle inequality, |(k
- Page 275 and 276: 267 Table 28.1: Table of Special Fu
- Page 277 and 278: 269 Thus y = a 0 ( 1 + 1 6 t3 + 1 +
- Page 279 and 280: 271 into (28.114) and collect terms
- Page 281 and 282: 273 Summary of Power series method.
- Page 283 and 284: Lesson 29 Regular Singularities The
- Page 285 and 286: 277 ∑ ∞ ∞∑ ∞∑ 0 = t 2 a
- Page 287 and 288: 279 Case 2: Two equal real roots. S
- Page 289 and 290: 281 Example 29.6. Solve t 2 y ′
- Page 291 and 292: Lesson 30 The Method of Frobenius I
- Page 293 and 294: 285 This is a homogeneous linear eq
- Page 295 and 296: 287 Example 30.4. Find a Frobenius
- Page 297 and 298: 289 Thus a Frobenius solution is y
- Page 299 and 300: 291 Example 30.6. Find the form of
- Page 301 and 302: 293 term by term to (30.97). Starti
- Page 303 and 304: 295 Let j = n − k. Then |n − 1
- Page 305 and 306: 297 is a solution of (t − t 0 ) 2
249<br />
By the lemma, to obta<strong>in</strong> the derivative of a determ<strong>in</strong>ant, we differentiate<br />
row by row and add the results, hence<br />
dW<br />
dt<br />
=<br />
∣<br />
y 1 ′ · · · y n<br />
′ y 1<br />
′ y n<br />
′ y 1 ′ · · · y ′ n<br />
y 1 ′′ y n<br />
′′<br />
y 1 ′′ y ′′<br />
n<br />
+<br />
y 1 ′′ y ′′<br />
n<br />
.<br />
.<br />
.<br />
.<br />
1 · · · y n<br />
(n−1) ∣ ∣y (n−1)<br />
1 · · · y n<br />
(n−1) ∣<br />
y 1 · · · y n<br />
y 1<br />
′ y ′ n<br />
+ · · · +<br />
y 1 ′′ y n<br />
′′<br />
. .<br />
∣y (n)<br />
1 · · · y n<br />
(n) ∣<br />
y (n−1)<br />
(27.178)<br />
Every determ<strong>in</strong>ant except for the last conta<strong>in</strong>s a repeated row, and s<strong>in</strong>ce<br />
the determ<strong>in</strong>ant of a matrix with a repeated row is zero, the only nonzero<br />
term is the last term.<br />
dW<br />
dt<br />
=<br />
∣<br />
y 1 · · · y n<br />
y ′ 1<br />
y ′ n<br />
y 1 ′′ y n<br />
′′<br />
. .<br />
y (n)<br />
1 · · · y n<br />
(n)<br />
∣<br />
(27.179)<br />
S<strong>in</strong>ce each y j is a solution of the homogeneous equation,<br />
y (n)<br />
j<br />
= − a n−1(t)<br />
a n (t) y(n−1) j − a n−2(t)<br />
a n (t) y(n−2) j − · · · − a 0(t)<br />
a n (t) y j (27.180)<br />
= − 1<br />
n−1<br />
∑<br />
a i (t)y (i)<br />
j (27.181)<br />
a n (t)<br />
i=0<br />
Hence