Lecture Notes in Differential Equations - Bruce E. Shapiro

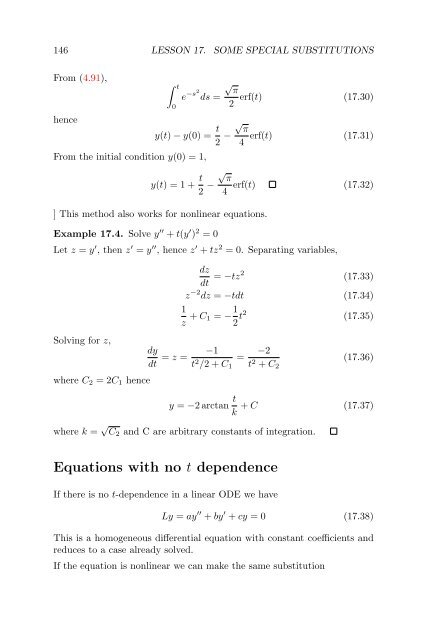
146 LESSON 17. SOME SPECIAL SUBSTITUTIONS
From (4.91),
hence
∫ t
From the initial condition y(0) = 1,
0
√ π
e −s2 ds = erf(t) (17.30)
2
y(t) − y(0) = t √ π
2 − erf(t) (17.31)
4
y(t) = 1 + t √ π
2 − erf(t) (17.32)
4
] This method also works for nonlinear equations.
Example 17.4. Solve y ′′ + t(y ′ ) 2 = 0
Let z = y ′ , then z ′ = y ′′ , hence z ′ + tz 2 = 0. Separating variables,
Solving for z,
where C 2 = 2C 1 hence
dy
dt = z =
dz
dt = −tz2 (17.33)
z −2 dz = −tdt (17.34)
1
z + C 1 = − 1 2 t2 (17.35)
−1
t 2 /2 + C 1
=
−2
t 2 + C 2
(17.36)
y = −2 arctan t k + C (17.37)
where k = √ C 2 and C are arbitrary constants of integration.
Equations with no t dependence
If there is no t-dependence in a linear ODE we have
Ly = ay ′′ + by ′ + cy = 0 (17.38)
This is a homogeneous differential equation with constant coefficients and
reduces to a case already solved.
If the equation is nonlinear we can make the same substitution