v2009.01.01 - Convex Optimization
v2009.01.01 - Convex Optimization v2009.01.01 - Convex Optimization
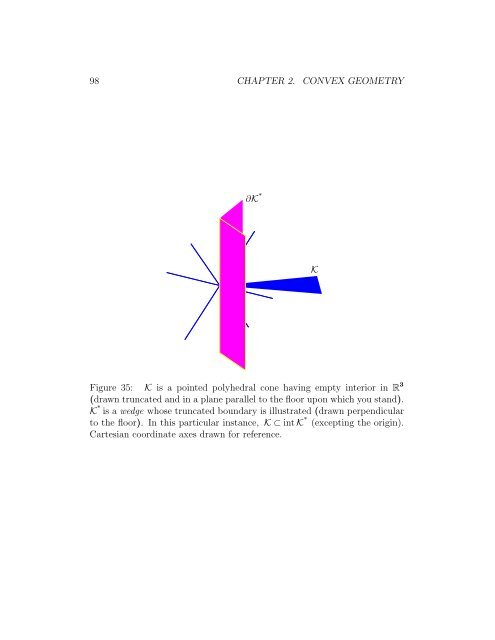
98 CHAPTER 2. CONVEX GEOMETRY ∂K ∗ K Figure 35: K is a pointed polyhedral cone having empty interior in R 3 (drawn truncated and in a plane parallel to the floor upon which you stand). K ∗ is a wedge whose truncated boundary is illustrated (drawn perpendicular to the floor). In this particular instance, K ⊂ int K ∗ (excepting the origin). Cartesian coordinate axes drawn for reference.
2.8. CONE BOUNDARY 99 2.8.1.1 extreme distinction, uniqueness An extreme direction is unique, but its vector representation Γ ε is not because any positive scaling of it produces another vector in the same (extreme) direction. Hence an extreme direction is unique to within a positive scaling. When we say extreme directions are distinct, we are referring to distinctness of rays containing them. Nonzero vectors of various length in the same extreme direction are therefore interpreted to be identical extreme directions. 2.34 The extreme directions of the polyhedral cone in Figure 20 (p.65), for example, correspond to its three edges. For any pointed polyhedral cone, there is a one-to-one correspondence of one-dimensional faces with extreme directions. The extreme directions of the positive semidefinite cone (2.9) comprise the infinite set of all symmetric rank-one matrices. [20,6] [170,III] It is sometimes prudent to instead consider the less infinite but complete normalized set, for M >0 (confer (206)) {zz T ∈ S M | ‖z‖= 1} (169) The positive semidefinite cone in one dimension M =1, S + the nonnegative real line, has one extreme direction belonging to its relative interior; an idiosyncrasy of dimension 1. Pointed closed convex cone K = {0} has no extreme direction because extreme directions are nonzero by definition. If closed convex cone K is not pointed, then it has no extreme directions and no vertex. [20,1] Conversely, pointed closed convex cone K is equivalent to the convex hull of its vertex and all its extreme directions. [266,18, p.167] That is the practical utility of extreme direction; to facilitate construction of polyhedral sets, apparent from the extremes theorem: 2.34 Like vectors, an extreme direction can be identified by the Cartesian point at the vector’s head with respect to the origin.
- Page 47 and 48: 2.2. VECTORIZED-MATRIX INNER PRODUC
- Page 49 and 50: 2.2. VECTORIZED-MATRIX INNER PRODUC
- Page 51 and 52: 2.2. VECTORIZED-MATRIX INNER PRODUC
- Page 53 and 54: 2.2. VECTORIZED-MATRIX INNER PRODUC
- Page 55 and 56: 2.3. HULLS 55 Figure 16: Convex hul
- Page 57 and 58: 2.3. HULLS 57 The affine hull of tw
- Page 59 and 60: 2.3. HULLS 59 2.3.2 Convex hull The
- Page 61 and 62: 2.3. HULLS 61 In case k = N , the F
- Page 63 and 64: 2.3. HULLS 63 2.3.2.0.3 Exercise. C
- Page 65 and 66: 2.3. HULLS 65 Figure 20: A simplici
- Page 67 and 68: 2.4. HALFSPACE, HYPERPLANE 67 H + a
- Page 69 and 70: 2.4. HALFSPACE, HYPERPLANE 69 1 1
- Page 71 and 72: 2.4. HALFSPACE, HYPERPLANE 71 Recal
- Page 73 and 74: 2.4. HALFSPACE, HYPERPLANE 73 C H
- Page 75 and 76: 2.4. HALFSPACE, HYPERPLANE 75 2.4.2
- Page 77 and 78: 2.4. HALFSPACE, HYPERPLANE 77 (conf
- Page 79 and 80: 2.5. SUBSPACE REPRESENTATIONS 79 Ra
- Page 81 and 82: 2.5. SUBSPACE REPRESENTATIONS 81 If
- Page 83 and 84: 2.6. EXTREME, EXPOSED 83 2.6 Extrem
- Page 85 and 86: 2.6. EXTREME, EXPOSED 85 A B C D Fi
- Page 87 and 88: 2.7. CONES 87 2.6.1.3.1 Definition.
- Page 89 and 90: 2.7. CONES 89 0 Figure 30: Boundary
- Page 91 and 92: 2.7. CONES 91 2.7.2 Convex cone We
- Page 93 and 94: 2.7. CONES 93 Then a pointed closed
- Page 95 and 96: 2.7. CONES 95 A pointed closed conv
- Page 97: 2.8. CONE BOUNDARY 97 So the ray th
- Page 101 and 102: 2.8. CONE BOUNDARY 101 2.8.2 Expose
- Page 103 and 104: 2.8. CONE BOUNDARY 103 From Theorem
- Page 105 and 106: 2.9. POSITIVE SEMIDEFINITE (PSD) CO
- Page 107 and 108: 2.9. POSITIVE SEMIDEFINITE (PSD) CO
- Page 109 and 110: 2.9. POSITIVE SEMIDEFINITE (PSD) CO
- Page 111 and 112: 2.9. POSITIVE SEMIDEFINITE (PSD) CO
- Page 113 and 114: 2.9. POSITIVE SEMIDEFINITE (PSD) CO
- Page 115 and 116: 2.9. POSITIVE SEMIDEFINITE (PSD) CO
- Page 117 and 118: 2.9. POSITIVE SEMIDEFINITE (PSD) CO
- Page 119 and 120: 2.9. POSITIVE SEMIDEFINITE (PSD) CO
- Page 121 and 122: 2.9. POSITIVE SEMIDEFINITE (PSD) CO
- Page 123 and 124: 2.9. POSITIVE SEMIDEFINITE (PSD) CO
- Page 125 and 126: 2.9. POSITIVE SEMIDEFINITE (PSD) CO
- Page 127 and 128: 2.10. CONIC INDEPENDENCE (C.I.) 127
- Page 129 and 130: 2.10. CONIC INDEPENDENCE (C.I.) 129
- Page 131 and 132: 2.10. CONIC INDEPENDENCE (C.I.) 131
- Page 133 and 134: 2.12. CONVEX POLYHEDRA 133 It follo
- Page 135 and 136: 2.12. CONVEX POLYHEDRA 135 Coeffici
- Page 137 and 138: 2.12. CONVEX POLYHEDRA 137 2.12.3 U
- Page 139 and 140: 2.12. CONVEX POLYHEDRA 139
- Page 141 and 142: 2.13. DUAL CONE & GENERALIZED INEQU
- Page 143 and 144: 2.13. DUAL CONE & GENERALIZED INEQU
- Page 145 and 146: 2.13. DUAL CONE & GENERALIZED INEQU
- Page 147 and 148: 2.13. DUAL CONE & GENERALIZED INEQU
98 CHAPTER 2. CONVEX GEOMETRY<br />
∂K ∗<br />
K<br />
Figure 35: K is a pointed polyhedral cone having empty interior in R 3<br />
(drawn truncated and in a plane parallel to the floor upon which you stand).<br />
K ∗ is a wedge whose truncated boundary is illustrated (drawn perpendicular<br />
to the floor). In this particular instance, K ⊂ int K ∗ (excepting the origin).<br />
Cartesian coordinate axes drawn for reference.