v2009.01.01 - Convex Optimization
Share Embed Flag
Download ePaper

v2009.01.01 - Convex Optimization

v2009.01.01 - Convex Optimization v2009.01.01 - Convex Optimization

convexoptimization.com
from convexoptimization.com More from this publisher
10.03.2015 Views

436 CHAPTER 5. EUCLIDEAN DISTANCE MATRIX theorem (A.3.1.0.4) it is sufficient to prove all d ij are nonnegative, all triangle inequalities are satisfied, and det(−VN TDV N) is nonnegative. When N = 4, in other words, that nonnegative determinant becomes the fifth and last Euclidean metric requirement for D ∈ EDM N . We now endeavor to ascribe geometric meaning to it. 5.14.2.1 Nonnegative determinant By (867) when D ∈EDM 4 , −VN TDV N is equal to inner product (862), ⎡ √ √ ⎤ d 12 d12 d 13 cos θ 213 d12 √d12 √ d 14 cos θ 214 Θ T Θ = ⎣ d 13 cos θ 213 d 13 d13 √d12 d 14 cos θ 314 ⎦ √ (1062) d 14 cos θ 214 d13 d 14 cos θ 314 d 14 Because Euclidean space is an inner-product space, the more concise inner-product form of the determinant is admitted; det(Θ T Θ) = −d 12 d 13 d 14 ( cos(θ213 ) 2 +cos(θ 214 ) 2 +cos(θ 314 ) 2 − 2 cos θ 213 cosθ 214 cosθ 314 − 1 ) The determinant is nonnegative if and only if (1063) cos θ 214 cos θ 314 − √ sin(θ 214 ) 2 sin(θ 314 ) 2 ≤ cos θ 213 ≤ cos θ 214 cos θ 314 + √ sin(θ 214 ) 2 sin(θ 314 ) 2 ⇔ cos θ 213 cos θ 314 − √ sin(θ 213 ) 2 sin(θ 314 ) 2 ≤ cos θ 214 ≤ cos θ 213 cos θ 314 + √ sin(θ 213 ) 2 sin(θ 314 ) 2 ⇔ cos θ 213 cos θ 214 − √ sin(θ 213 ) 2 sin(θ 214 ) 2 ≤ cos θ 314 ≤ cos θ 213 cos θ 214 + √ sin(θ 213 ) 2 sin(θ 214 ) 2 which simplifies, for 0 ≤ θ i1l ,θ l1j ,θ i1j ≤ π and all i≠j ≠l ∈{2, 3, 4} , to (1064) cos(θ i1l + θ l1j ) ≤ cos θ i1j ≤ cos(θ i1l − θ l1j ) (1065) Analogously to triangle inequality (978), the determinant is 0 upon equality on either side of (1065) which is tight. Inequality (1065) can be equivalently written linearly as a triangle inequality between relative angles [344,1.4]; |θ i1l − θ l1j | ≤ θ i1j ≤ θ i1l + θ l1j θ i1l + θ l1j + θ i1j ≤ 2π 0 ≤ θ i1l ,θ l1j ,θ i1j ≤ π (1066)

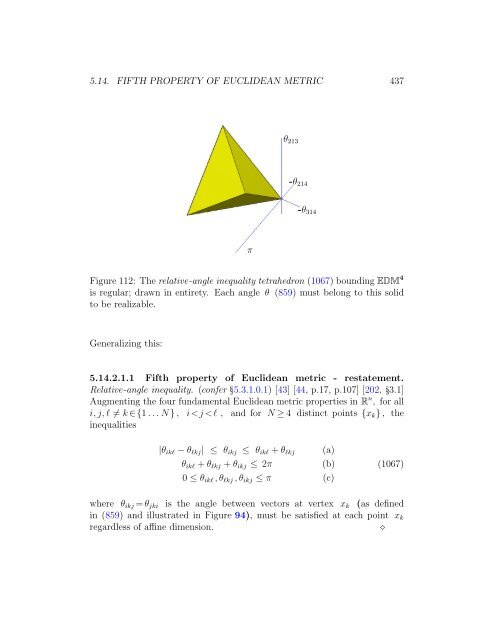
5.14. FIFTH PROPERTY OF EUCLIDEAN METRIC 437 θ 213 -θ 214 -θ 314 π Figure 112: The relative-angle inequality tetrahedron (1067) bounding EDM 4 is regular; drawn in entirety. Each angle θ (859) must belong to this solid to be realizable. Generalizing this: 5.14.2.1.1 Fifth property of Euclidean metric - restatement. Relative-angle inequality. (confer5.3.1.0.1) [43] [44, p.17, p.107] [202,3.1] Augmenting the four fundamental Euclidean metric properties in R n , for all i,j,l ≠ k ∈{1... N }, i

5.14. FIFTH PROPERTY OF EUCLIDEAN METRIC 437<br />

θ 213<br />

-θ 214<br />

-θ 314<br />

π<br />

Figure 112: The relative-angle inequality tetrahedron (1067) bounding EDM 4<br />

is regular; drawn in entirety. Each angle θ (859) must belong to this solid<br />

to be realizable.<br />

Generalizing this:<br />

5.14.2.1.1 Fifth property of Euclidean metric - restatement.<br />

Relative-angle inequality. (confer5.3.1.0.1) [43] [44, p.17, p.107] [202,3.1]<br />

Augmenting the four fundamental Euclidean metric properties in R n , for all<br />

i,j,l ≠ k ∈{1... N }, i

logo

products

Resources

Company

Terms of service
Privacy policy
Cookie policy
Cookie settings
Imprint
Change language
Made with love in Switzerland
© 2024 Yumpu.com all rights reserved

Hooray! Your file is uploaded and ready to be published.

Saved successfully!

Ooh no, something went wrong!