v2009.01.01 - Convex Optimization

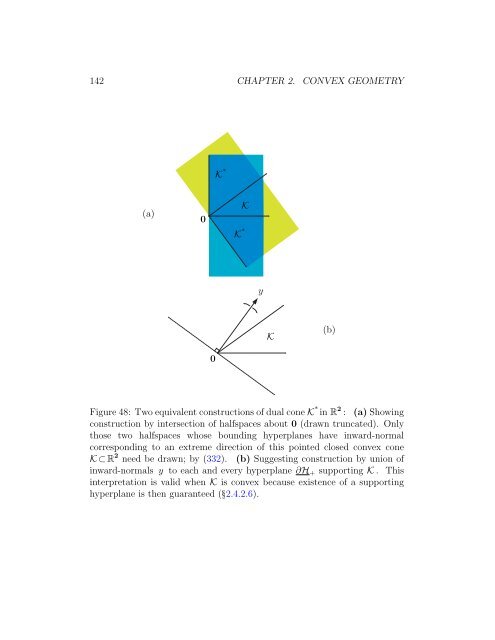
142 CHAPTER 2. CONVEX GEOMETRY
K ∗
(a)
0
K ∗
K
y
K
(b)
0
Figure 48: Two equivalent constructions of dual cone K ∗ in R 2 : (a) Showing
construction by intersection of halfspaces about 0 (drawn truncated). Only
those two halfspaces whose bounding hyperplanes have inward-normal
corresponding to an extreme direction of this pointed closed convex cone
K ⊂ R 2 need be drawn; by (332). (b) Suggesting construction by union of
inward-normals y to each and every hyperplane ∂H + supporting K . This
interpretation is valid when K is convex because existence of a supporting
hyperplane is then guaranteed (2.4.2.6).