Chapter 3 Geometry of convex functions - Meboo Publishing ...
Chapter 3 Geometry of convex functions - Meboo Publishing ...
Chapter 3 Geometry of convex functions - Meboo Publishing ...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
3.6. EPIGRAPH, SUBLEVEL SET 233<br />
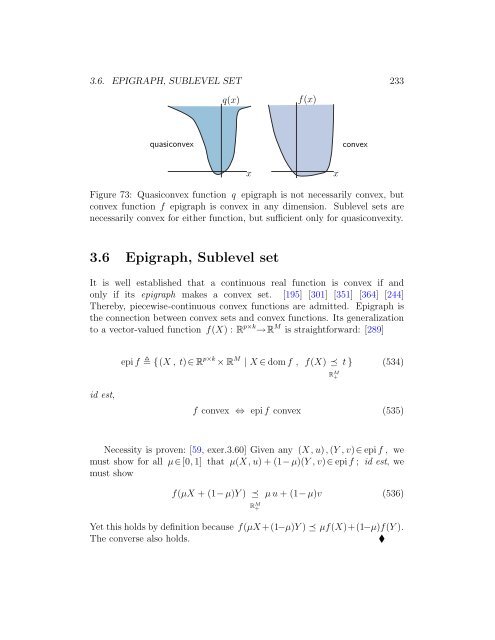
q(x)<br />
f(x)<br />
quasi<strong>convex</strong><br />
<strong>convex</strong><br />
x<br />
x<br />
Figure 73: Quasi<strong>convex</strong> function q epigraph is not necessarily <strong>convex</strong>, but<br />
<strong>convex</strong> function f epigraph is <strong>convex</strong> in any dimension. Sublevel sets are<br />
necessarily <strong>convex</strong> for either function, but sufficient only for quasi<strong>convex</strong>ity.<br />
3.6 Epigraph, Sublevel set<br />
It is well established that a continuous real function is <strong>convex</strong> if and<br />
only if its epigraph makes a <strong>convex</strong> set. [195] [301] [351] [364] [244]<br />
Thereby, piecewise-continuous <strong>convex</strong> <strong>functions</strong> are admitted. Epigraph is<br />
the connection between <strong>convex</strong> sets and <strong>convex</strong> <strong>functions</strong>. Its generalization<br />
to a vector-valued function f(X) : R p×k →R M is straightforward: [289]<br />
epi f {(X , t)∈ R p×k × R M | X ∈ domf , f(X) ≼<br />
t } (534)<br />
R M +<br />
id est,<br />
f <strong>convex</strong> ⇔ epif <strong>convex</strong> (535)<br />
Necessity is proven: [59, exer.3.60] Given any (X, u), (Y , v)∈ epif , we<br />
must show for all µ∈[0, 1] that µ(X, u) + (1−µ)(Y , v)∈ epif ; id est, we<br />
must show<br />
f(µX + (1−µ)Y ) ≼<br />
µu + (1−µ)v (536)<br />
R M +<br />
Yet this holds by definition because f(µX+(1−µ)Y ) ≼ µf(X)+(1−µ)f(Y ).<br />
The converse also holds.