(i) {α - Convex Optimization
(i) {α - Convex Optimization (i) {α - Convex Optimization
Characterizing Problem (Peq) min Ψ(α) s.t. y = HΦα. Proposition 1 (i) Existence: (P eq ) has at least one solution. (ii) Uniqueness: (P eq ) has a unique solution if ψ is strictly convex. (iii) If α solves (P eq ), then it solves (P σ=0 ). Stanford seminar 08-20
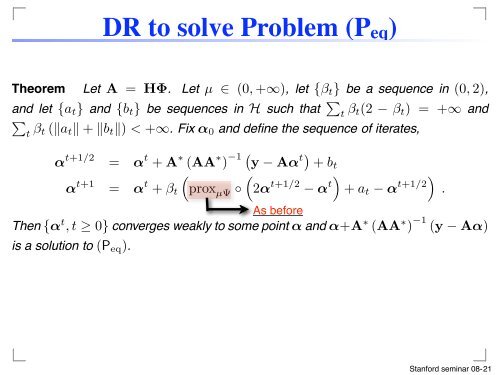
DR to solve Problem (Peq) Theorem 1 Let A = HΦ. Let µ ∈ (0, +∞), let {β t } be a sequence in (0, 2), and let {a t } and {b t } be sequences in H such that ∑ t β t(2 − β t ) = +∞ and ∑ t β t (‖a t ‖ + ‖b t ‖) < +∞. Fix α 0 and define the sequence of iterates, α t+1/2 = α t + A ∗ (AA ∗ ) −1 ( y − Aα t) + b t ( α t+1 = α t + β t (prox µΨ ◦ 2α t+1/2 − α t) + a t − α t+1/2) . Then {α t , t ≥ 0} converges weakly to some point α and α+A ∗ (AA ∗ ) −1 (y − Aα) is a solution to (P eq ). As before Stanford seminar 08-21
- Page 1 and 2: Optimization problems in compressed
- Page 3 and 4: Compressed/ive Sensing Stanford sem
- Page 5 and 6: Compressed/ive Sensing Common wisdo
- Page 7 and 8: Compressed/ive Sensing Common wisdo
- Page 9 and 10: Compressed/ive Sensing (cont’d) C
- Page 11 and 12: Compressed/ive Sensing (cont’d) C
- Page 13 and 14: Convex analysis and operator splitt
- Page 15 and 16: Class of problems in CS (cont’d)
- Page 17 and 18: Class of problems in CS (cont’d)
- Page 19 and 20: Characterization Theorem 1 (i) Exis
- Page 21 and 22: Operator splitting schemes Idea: re
- Page 23 and 24: Proximity operators Some properties
- Page 25 and 26: Example of proximity operator Stanf
- Page 27 and 28: Compressed sensing optimization pro
- Page 29 and 30: Characterizing Problem (P τ ) Stan
- Page 31 and 32: Proximity operators of Ψ Conclusio
- Page 33: DR to solve Problem (P σ ) Theorem
- Page 37 and 38: Pros and cons (P σ ) and (P eq ) h
- Page 39 and 40: CS reconstruction (1) H = Fourier,
- Page 41 and 42: Inpainting and CS H = Dirac, Φ = C
- Page 43 and 44: Inpainting and CS H = Dirac, Φ = C
- Page 45 and 46: Computation time CS H = Fourier, Φ
- Page 47 and 48: Ongoing and future work Beyond the
DR to solve Problem (Peq)<br />
Theorem 1 Let A = HΦ. Let µ ∈ (0, +∞), let {β t } be a sequence in (0, 2),<br />
and let {a t } and {b t } be sequences in H such that ∑ t β t(2 − β t ) = +∞ and<br />
∑<br />
t β t (‖a t ‖ + ‖b t ‖) < +∞. Fix α 0 and define the sequence of iterates,<br />
α t+1/2 = α t + A ∗ (AA ∗ ) −1 ( y − Aα t) + b t<br />
(<br />
α t+1 = α t + β t<br />
(prox µΨ ◦ 2α t+1/2 − α t) + a t − α t+1/2) .<br />
Then {α t , t ≥ 0} converges weakly to some point α and α+A ∗ (AA ∗ ) −1 (y − Aα)<br />
is a solution to (P eq ).<br />
As before<br />
Stanford seminar 08-21