ON GLOBAL RIEMANN-CARTAN GEOMETRY

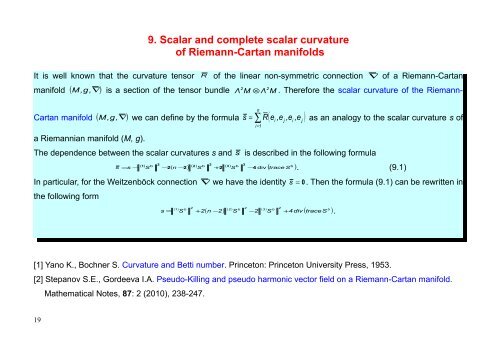
9. Scalar and complete scalar curvature
of Riemann-Cartan manifolds
It is well known that the curvature tensor R of the linear non-symmetric connection ∇ of a Riemann-Cartan
manifold ( ,g,∇)
2
2
M is a section of the tensor bundle Λ M ⊗ Λ M . Therefore the scalar curvature of the Riemann-
Cartan manifold ( ,g,∇)
a Riemannian manifold (M, g).
n
M we can define by the formula s ∑ R( e ,e ,e ,e )
_
= as an analogy to the scalar curvature s of
The dependence between the scalar curvatures s and s is described in the following formula
i = 1
( 1) b
2
( ) ( 2 ) b
2 ( 3 ) b
2
b
= s − S −2
n −2
S + 2 S − div ( trace S ). (9.1)
s 4
In particular, for the Weitzenböck connection ∇ we have the identity s = 0. Then the formula (9.1) can be rewritten in
the following form
( 1) b
2
( ) ( 2 ) b
2 ( 3 ) b
2
b
S + 2 n −2
S −2
S 4div ( traceS )
s = +
.
i
j
i
j
[1] Yano K., Bochner S. Curvature and Betti number. Princeton: Princeton University Press, 1953.
[2] Stepanov S.E., Gordeeva I.A. Pseudo-Killing and pseudo harmonic vector field on a Riemann-Cartan manifold.
Mathematical Notes, 87: 2 (2010), 238-247.
19