MCS 351 ENGINEERING MATHEMATICS SOLUTION OF ...
MCS 351 ENGINEERING MATHEMATICS SOLUTION OF ... MCS 351 ENGINEERING MATHEMATICS SOLUTION OF ...
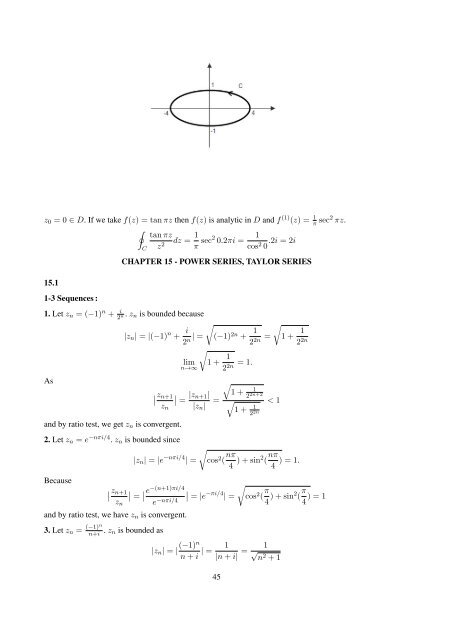
z 0 = 0 ∈ D. If we take f(z) = tan πz then f(z) is analytic in D and f (1) (z) = 1 π sec2 πz. ∮ tan πz z 2 dz = 1 π sec2 0.2πi = 1 cos 2 .2i = 2i 0 15.1 1-3 Sequences : C CHAPTER 15 - POWER SERIES, TAYLOR SERIES 1. Let z n = (−1) n + i 2 n . z n is bounded because As and by ratio test, we get z n is convergent. 2. Let z n = e −nπi/4 . z n is bounded since Because |z n | = |(−1) n + i √ 2 n | = (−1) 2n + 1 √ 2 2n = 1 + 1 √ lim n→∞ 1 + 1 = 1. 22n √ ∣ z n+1 ∣ |z n+1 | 1 + 1 2 = = √ 2n+2 < 1 z n |z n | 1 + 1 2 2n |z n | = |e −nπi/4 | = √ cos 2 ( nπ 4 ) + sin2 ( nπ 4 ) = 1. 2 2n ∣ z n+1 ∣ ∣ = e −(n+1)πi/4 √ ∣ = |e −πi/4 z n e −nπi/4 | = cos 2 ( π 4 ) + sin2 ( π 4 ) = 1 and by ratio test, we have z n is convergent. 3. Let z n = (−1)n n+i . z n is bounded as |z n | = | (−1)n n + i | = 1 |n + i| = 1 √ n 2 + 1 45
Because lim n→∞ 1 √ n 2 + 1 = 0. ∣ z √ n+1 ∣ ∣ = n + i ∣ |n + i| n = z n n + 1 + i |n + 1 + i| = 2 + 1 √ (n + 1) 2 + 1 < 1 and from ratio test, we have z n is convergent. 16-18 Series : 16. Let ∞∑ (10 − 15i) n n=0 n→∞ n! lim ∣ z n+1 ∣ = lim ∣ z n n→∞ and by ratio test we get this result. 17. Let and z n = (−1)n (1+2i) 2n+1 (2n+1)! . This serie is convergent from ratio test because 18. Let ∞∑ (−1) n (1 + 2i) 2n+1 n=0 ∞∑ n=0 n→∞ and we know that 15.2 (2n + 1)! and z n = (10−15i)n n! . This serie is convergent because (10−15i) n+1 (n+1)! (10−15i) n n! ∣ = lim n→∞ ∣ √ 10 − 15i ∣ = lim n + 1 n→∞ 325 (n + 1) 2 = 0 lim ∣ z n+1 ∣ ∣ (1 + 2i) = lim ∣(−1) 2 ∣ z n n→∞ (2n + 3)(2n + 2) i n n 2 − 2i and z n = ∞∑ n=0 3-5 Radius of Convergence : ∣ = lim n→∞ 5 (2n + 3)(2n + 2) = 0. in . This serie is convergent, we now explain this situation : n 2 −2i ∣ i n n 2 − 2i | = |in | |n 2 − 2i| = 1 |n 2 − 2i| ≤ 1 n 2 1 is convergent. Then via comparison test, we can say that this serie is convergent. n2 ∞∑ (z + i) n 3. Let . First, we determine the center. n=1 n 2 z + i = 0 ⇒ z = −i is the center point. On the other hand, z n = (z+i)n n 2 and because lim n→∞ radius of convergence is 1. ∣ 1 n 2 1 n→∞ (n+1) 2 | = lim ∣ (n + 1)2 ∣ n 2 + 2n + 1 = lim n 2 n→∞ n 2 = 1, 46
- Page 1 and 2: MCS 351 ENGINEERING MATHEMATICS SOL
- Page 3 and 4: a + b = 90 ◦ 3. z 1 = x 1 + iy 1
- Page 5 and 6: the fact that tan θ = π 2 , θ =
- Page 7 and 8: For this reason, tan α = 0 and so
- Page 9 and 10: k = 2 =⇒ z 2 = 3√ 5(cos(θ + 4
- Page 11 and 12: 4. Let’s now determine the region
- Page 13 and 14: 12-15 Function values: 12. For z =
- Page 15 and 16: 13.4 1-10 Cauchy-Riemann equations:
- Page 17 and 18: =⇒ f(z) = x 3 − 3xy 2 + i(3x 2
- Page 19 and 20: 13-17 Polar form: 13. z = √ i ⇒
- Page 21 and 22: then, cosh(z 1 + z 2 ) = cos(i(z 1
- Page 23 and 24: Then we obtain that e 2z + 1 = 0
- Page 25 and 26: ⇒ e x . cos y = − cos(1) and e
- Page 27 and 28: 4. z(t) = 1 + i + e −πit , 0 ≤
- Page 29 and 30: • t = 0 ⇒ z(0) = 0 • t = 2
- Page 31 and 32: • x = −1 ⇒ y = 4 − 4 = 0
- Page 33 and 34: f(z) = e 2z is analytic in C. So we
- Page 35 and 36: 27. z(t) = ( π 4 − t) + ti, (0
- Page 37 and 38: z 2 + 4 = 0 =⇒ z 2 = −4 =⇒ z
- Page 39 and 40: İf z = x + iy, dz = dx + idy = idy
- Page 41 and 42: Let D be the union of C and the int
- Page 43 and 44: Let g(z) = ln(z−1) z−5 . Singul
- Page 45: Hence we see that when k ∈ {0, 1,
- Page 49 and 50: 15.4 1-3 Taylor and Maclaurin serie
- Page 51: ∞∑ 11. Let n=1 (−1) n 2 n n z
z 0 = 0 ∈ D. If we take f(z) = tan πz then f(z) is analytic in D and f (1) (z) = 1 π sec2 πz.<br />
∮<br />
tan πz<br />
z 2 dz = 1 π sec2 0.2πi = 1<br />
cos 2 .2i = 2i<br />
0<br />
15.1<br />
1-3 Sequences :<br />
C<br />
CHAPTER 15 - POWER SERIES, TAYLOR SERIES<br />
1. Let z n = (−1) n + i<br />
2 n . z n is bounded because<br />
As<br />
and by ratio test, we get z n is convergent.<br />
2. Let z n = e −nπi/4 . z n is bounded since<br />
Because<br />
|z n | = |(−1) n + i<br />
√<br />
2 n | = (−1) 2n + 1<br />
√<br />
2 2n = 1 + 1<br />
√<br />
lim<br />
n→∞<br />
1 + 1 = 1.<br />
22n √<br />
∣ z n+1<br />
∣ |z n+1 | 1 + 1<br />
2<br />
= = √<br />
2n+2<br />
< 1<br />
z n |z n |<br />
1 + 1<br />
2 2n<br />
|z n | = |e −nπi/4 | =<br />
√<br />
cos 2 ( nπ 4 ) + sin2 ( nπ 4 ) = 1.<br />
2 2n<br />
∣ z n+1<br />
∣ ∣ = e −(n+1)πi/4<br />
√<br />
∣ = |e −πi/4<br />
z n e −nπi/4 | = cos 2 ( π 4 ) + sin2 ( π 4 ) = 1<br />
and by ratio test, we have z n is convergent.<br />
3. Let z n = (−1)n<br />
n+i . z n is bounded as<br />
|z n | = | (−1)n<br />
n + i | = 1<br />
|n + i| = 1<br />
√<br />
n 2 + 1<br />
45