MCS 351 ENGINEERING MATHEMATICS SOLUTION OF ...

30. For z 4 + 16 = 0, let z 2 = u. Then
√
i can be determined as following:
u 2 = −16 ⇒ u = ∓4i ⇒ z 2 = ∓4i ⇒ z = ∓2 √ i.
As a result, all roots are ∓(1 ∓ i). √ 2.
√
i = ∓[
√
1
2 + 1.i. √
1
2 ] = ∓ 1 √
2
(1 + i).
13.3
1-10 Find and graph followings in the complex plane:
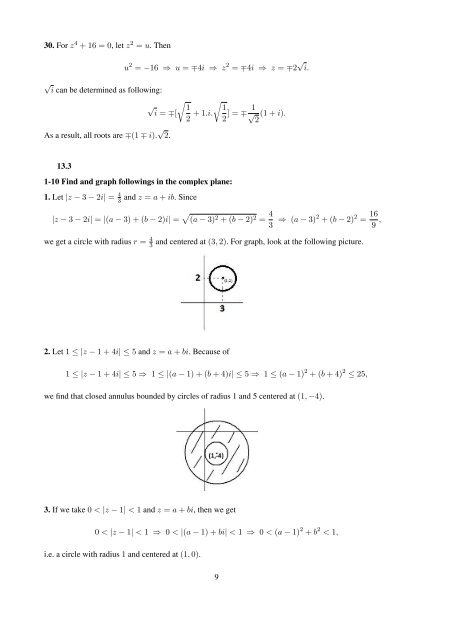
1. Let |z − 3 − 2i| = 4 3
and z = a + ib. Since
|z − 3 − 2i| = |(a − 3) + (b − 2)i| = √ (a − 3) 2 + (b − 2) 2 = 4 3 ⇒ (a − 3)2 + (b − 2) 2 = 16
9 ,
we get a circle with radius r = 4 3
and centered at (3, 2). For graph, look at the following picture.
2. Let 1 ≤ |z − 1 + 4i| ≤ 5 and z = a + bi. Because of
1 ≤ |z − 1 + 4i| ≤ 5 ⇒ 1 ≤ |(a − 1) + (b + 4)i| ≤ 5 ⇒ 1 ≤ (a − 1) 2 + (b + 4) 2 ≤ 25,
we find that closed annulus bounded by circles of radius 1 and 5 centered at (1, −4).
3. If we take 0 < |z − 1| < 1 and z = a + bi, then we get
0 < |z − 1| < 1 ⇒ 0 < |(a − 1) + bi| < 1 ⇒ 0 < (a − 1) 2 + b 2 < 1,
i.e. a circle with radius 1 and centered at (1, 0).
9