Stream Tube
Stream Tube
Stream Tube
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
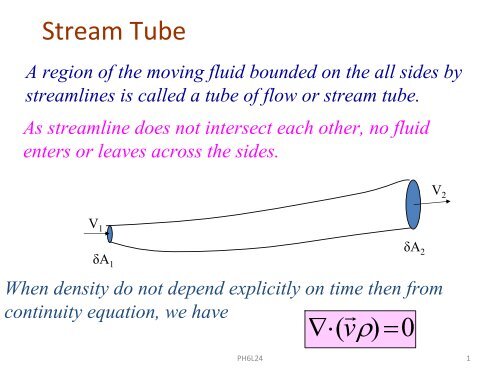
<strong>Stream</strong> <strong>Tube</strong><br />
A region of the moving fluid bounded on the all sides by<br />
streamlines is called a tube of flow or stream tube.<br />
As streamline does not intersect each other, no fluid<br />
enters or leaves across the sides.<br />
V 1<br />
V 2<br />
δA 1<br />
δA 2<br />
When density do not depend explicitly on time then from<br />
continuity equation, we have <br />
∇⋅ ( vρ) = 0<br />
PH6L24 1
∫ ∇⋅ <br />
=<br />
V<br />
∫<br />
s<br />
( vρ) 0<br />
<br />
( vρ ). dS=<br />
0<br />
Where V is the volume of the<br />
streamtube between faces δA 1 and<br />
δA 2<br />
From divergence theorem<br />
v <br />
ρ and vρ are the mean values of ρvover δA and δA<br />
1 2<br />
<br />
vρ δA= vρ δA<br />
1 1 2 2<br />
1 2<br />
For a homogeneous, incompressible, Non-viscous fluids<br />
<br />
v<br />
<br />
δA= v δA<br />
1 1 2 2<br />
Thus, whenever stream tube is constricted, i.e., wherever<br />
streamlines gets crowded, the speed of flow of liquid is larger<br />
PH6L24 2
For the entire collection of the stream tubes occupying the<br />
whole cross section of the passage through which the fluid<br />
flows<br />
∫ ρvdS<br />
A<br />
is constant along the passage<br />
In the case of constant density<br />
∫ vdS is constant<br />
or<br />
<br />
v<br />
<br />
A= v A<br />
1 1 2 2<br />
A<br />
PH6L24 3
Bernoulli’s Principle and Physical Significance of<br />
different terms<br />
In the steady flow of homogeneous, incompressible non-viscous fluid,<br />
P<br />
1<br />
v<br />
gz<br />
Constant where gravitional potential<br />
2<br />
ρ + 2<br />
+ = Ω<br />
=gz<br />
Along a very narrow stream tube<br />
In the case of the gases last term is negligible w.r.t. first two terms as the<br />
Density is very low for the gases<br />
Third term is gravitational potential energy of the unit mass<br />
Second term is kinetic energy of the unit mass<br />
First term also has units of energy per unit mass<br />
What energy this term represents<br />
PH6L24 4
δl 1<br />
δl 2<br />
P 1<br />
P 2<br />
A B<br />
1<br />
1<br />
A 2<br />
B 2<br />
Consider the volume of fluid element between A1 and A2 which is<br />
moved after some time to volume between B1 and B2<br />
δlδA = δl δA = δV<br />
1 1 2 2<br />
Work done during the motion by the pressure forces is given by<br />
δlPδA− δlPδA = ( P−P)<br />
δV<br />
1 1 1 2 2 2 1 2<br />
Work done during the motion by the pressure forces in moving the<br />
volume element from A1B1 to A2B2 per unit mass is given by<br />
P2<br />
( P1−P2)<br />
δV P1−P2<br />
dp<br />
= = ∫ −<br />
ρδV<br />
ρ ρ<br />
PH6L24 5<br />
P1
P2 0<br />
∫<br />
P1<br />
dp dp P<br />
− = ∫− =<br />
ρ ρ ρ<br />
P<br />
Therefore, P/ρ term represents the work that will be done on<br />
the unit mass of the fluid by the pressure forces, as the<br />
element flows from a point where pressure is P to a point<br />
where pressure is zero.<br />
At two different point in the stream tube<br />
P 1 P 1<br />
+ v + gz = + v + gz<br />
ρ 2 ρ 2<br />
1 2 2 2<br />
1 1 2 2<br />
P<br />
− P<br />
ρ<br />
1 1<br />
2 2<br />
1 2<br />
2 2<br />
⇒ + gz (<br />
1− z2)<br />
= v2 − v1<br />
In the steady flow of an incompressible, homogeneous, nonviscous<br />
fluid, moving from position 1 to 2, the increase in<br />
kinetic energy per unit mass is equal to the work done on unit<br />
mass of the fluid by pressure and gravity forces.<br />
PH6L24 6
The three terms is therefore called as pressure energy,<br />
kinetic energy and gravitational energy<br />
2<br />
P v<br />
, , z are known as pressure head, velocity head<br />
ρg 2g<br />
and gravity head and each having the dimention of length<br />
PH6L24 7
Application 1: Velocity of efflux from a reservoir<br />
P 1<br />
P 2<br />
Flow from a sharp<br />
edged orifice in a tank<br />
Vena Contracta<br />
Following assumptions are made in order to simplify the problem<br />
• steady state flow of Incompressible, homogeneous, nonviscous<br />
fluid (frictional effects can be neglected)<br />
•Cross sectional area of the reservoir is very large compared to<br />
that of orifice. Hence the velocity of the fluid at the surface is<br />
negligibly small. Practically hydrostatic equilibrium condition<br />
exist for points at the surface.<br />
PH6L24 8
From the Bernoulli’s principle, we can write<br />
P2<br />
≈ P atm<br />
v1 = 0<br />
1 atm<br />
1<br />
P 1 P 1<br />
+ v + z = + v + z<br />
ρg 2g ρg 2g<br />
1 2 2<br />
2<br />
1 1 2 2<br />
z<br />
2<br />
= 0 when centre of the orifice is chosen<br />
as the horizontal plane for the gravitaional head<br />
P − P = ρg( h−z<br />
) from the hydrostatic condition<br />
Putting these all values<br />
v<br />
2<br />
= 2<br />
gh<br />
PH6L24 9
Identical relation for velocity of efflux is obtained for a<br />
discharge from one reservoir to another reservoir where h<br />
now stand for difference between the gravitational head<br />
across the orifice<br />
The rate of discharge Q, i.e., the volume of the fluid flowing<br />
out of the reservoir per unit time is given by<br />
Q Av A gh<br />
actual<br />
= αα<br />
c v 2<br />
= αd<br />
2<br />
Q= Av = A gh<br />
2<br />
2<br />
Actual Velocity<br />
where αv<br />
= ; αc<br />
= Cofficient of contraction<br />
Ideal Velocity<br />
and αα = α is called coefficient of discharge<br />
c v d<br />
PH6L24 10
Application 2: Flow meters<br />
From the Bernoulli’s<br />
principle for the<br />
streamline OA<br />
1 2 1 2<br />
P0 + ρv0 + ρgz0<br />
= PA + ρvA + ρgzA<br />
2 2<br />
For steady state, and OA taken as horizontal and velocity at<br />
A is zero from the definition of stagnation point<br />
1 2 1 2<br />
PA<br />
= P0 + ρv0 or PA<br />
− P0 = ρv0<br />
2 2<br />
Thus pressure at the stagnation point exceed the pressure<br />
at any other point on the streamline OA exceeds by 1 2<br />
2 ρv<br />
Dynamic pressure<br />
PH6L24 11
Vortex Line<br />
In the steady flow of homogeneous, incompressible,<br />
non-viscous fluid the Eulers equation is given by<br />
<br />
∂v<br />
1 ⎛1<br />
⎞<br />
− v× ( ∇× v)<br />
=− ∇p−∇ v<br />
2<br />
⎜ +Ω⎟<br />
∂t<br />
ρ ⎝2<br />
⎠<br />
Instead of taking a dot product with velocity vector as we<br />
have taken earlier, we take dot product with vorticity vector<br />
⎛ ⎞ <br />
χ χ φ<br />
ρ<br />
P 1<br />
. v<br />
2<br />
. 0<br />
2<br />
∇ ⎜ + +Ω ⎟= ∇ =<br />
⎝ ⎠<br />
Therefore, the potential φ is constant along the vortex line<br />
PH6L24 12
P v<br />
2<br />
+<br />
ρ 2<br />
+ Ω =<br />
C<br />
May be satisfied by more than one stream line and more than one vortex line<br />
Circular flow with straight streamline<br />
Non-zero curl or circulation does not necessary mean<br />
that the streamlines are circular or curved Ex.<br />
<br />
v<br />
<br />
∇ × v <br />
=<br />
v<br />
( y)<br />
ˆ<br />
x<br />
e x<br />
⎡dvx<br />
( y)<br />
⎤<br />
= −⎢<br />
⎥eˆ<br />
⎣ dy ⎦<br />
z<br />
≠ 0<br />
Flow is rotational even though the stream lines are parallel straight lines<br />
PH6L24 13