ECE 316, Fall 2005 Solutions to Problem Set 1 - for tutorials week of ...

ECE 316, Fall 2005
Solutions to Problem Set 1 - for tutorials week of Sept. 19
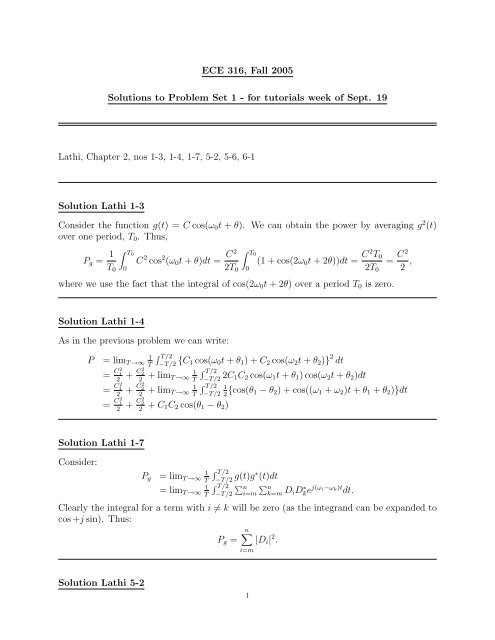
Lathi, Chapter 2, nos 1-3, 1-4, 1-7, 5-2, 5-6, 6-1
Solution Lathi 1-3
Consider the function g(t) = C cos(ω 0 t + θ). We can obtain the power by averaging g 2 (t)
over one period, T 0 . Thus,
P g = 1 ∫ T0
C 2 cos 2 (ω 0 t + θ)dt = C2 ∫ T0
(1 + cos(2ω 0 t + 2θ))dt = C2 T 0
T 0 0
2T 0 0
2T 0
where we use the fact that the integral of cos(2ω 0 t + 2θ) over a period T 0 is zero.
= C2
2 ,
Solution Lathi 1-4
As in the previous problem we can write:
1 ∫ T/2
P = lim T →∞ T
= C2 1
2
+ C2 2
1
2
+ lim T →∞ T
= C2 1
2
+ C2 2
1
2
+ lim T →∞ T
−T/2 {C 1 cos(ω 0 t + θ 1 ) + C 2 cos(ω 2 t + θ 2 )} 2 dt
∫ T/2
−T/2 2C 1C 2 cos(ω 1 t + θ 1 ) cos(ω 2 t + θ 2 )dt
1
{cos(θ 2 1 − θ 2 ) + cos((ω 1 + ω 2 )t + θ 1 + θ 2 )}dt
∫ T/2
−T/2
= C2 1
2
+ C2 2
2
+ C 1 C 2 cos(θ 1 − θ 2 )
Solution Lathi 1-7
Consider:
1 ∫ T/2
P g = lim T →∞ T
= lim T →∞
1
T
−T/2 g(t)g∗ (t)dt
∫ T/2 ∑ ni=m ∑ nk=m
−T/2
D i Dke ∗ j(ω i−ω k )t dt.
Clearly the integral for a term with i ≠ k will be zero (as the integrand can be expanded to
cos +j sin). Thus:
n∑
P g = |D i | 2 .
i=m
Solution Lathi 5-2
1

From equation 2.31 the constant c is
c = 1 ∫ 1
g(t)x(t)dt =
E x
The energy in the error signal is then
E ɛ =
∫ 1
0
0
∫ 1
0
t · 1dt = 1/2.
(g(t) − 1 2 x(t))2 dt = 1 3 − 1 4 = 1 12 .
Solution Lathi 5-6
(a)
(i) g 1 (t) ∼ (2, −1) (ii) g 2 (t) ∼ (−1, 2) (iii) g 3 (t) ∼ (0, −1)
(iv) g 4 (t) ∼ (1, 2) (v) g 5 (t) ∼ (2, 1) (vi) g 6 (t) ∼ (3, 0).
(b) We can determine if a pair of vectors is orthogonal by checking if the dot product of
the two vectors is equal to zero. It is easy to verify that the following pairs each have a dot
product equal to zero: (i) and (iv); (ii) and (v); (iii) and (vi).
Solution Lathi 6-1
The correlation coefficient is given by c = √ 1 ∫ 1
ExE 0 x(t)g(t)dt. Thus
g
c 1 =< x(t), g 1 (t) >= ∫ 1
0 sin(2πt) sin(4πt)dt/√ E x E g1 = 0
c 2 = − ∫ 1
0 sin(2πt) sin(2πt)dt/√ E x E g2 = −1
c 3 = 0
c 4 = 2 ∫ 1/2
0 sin(2πt)/ √ √
2dt/ 1/2 · 1/2 = 2√ 2
. π
1. Determine which of the following signals are energy or power signals. For those that
are energy signals, determine their energy. For those that are power signals determine their
power.
Solution
(a) g 1 (t) = e −a|t| , −∞ < t < ∞, a > 0 (b) g 2 (t) = e a|t| , −∞ < t < ∞, a > 0
(c) g 3 (t) = ∑ N
i=1 a i e jnω 0t , −∞ < t < ∞
(a) An energy signal since E g = ∫ ∞
−∞ e−2a|t| dt = 2 ∫ ∞
0 e −2a|t| dt = 1/a.
(b) Neither an energy nor a power signal since the time average of the square of the signal
goes to infinity.
(c) As in a problem above this is a power signal with P g = ∑ N
i=1 |a i | 2 .

2. A collection of (complex) functions {φ n (t), n = 0, ±1, ±2 · · · } is called an orthonormal
set on the interval (0, T ) if
∫ T
(a) Show that the set of functions
{
√
1/T 0 ,
√
2/T 0 cos(ω 0 t),
0
{
1 m = n
φ n (t)φ ∗ m(t)dt = δ mn
0 otherwise.
√
2/T 0 sin(ω 0 t),
√
√
2/T 0 cos(2ω 0 t), 2/T 0 sin(2ω 0 t), · · · }
are orthonormal over any interval of the real line of
√
length T 0 = 2π/ω 0 .
(b) Show that the set of complex exponentials { 1/T 0 e jnω0t , n = 0, ±1, ±2, · · · } are orthonormal
over any interval of the real line of length T 0 = 2π/ω 0 .
Solution
(a) This derives from the fact that
∫ T0
sin 2 (nω 0 t)dt =
0
∫ T0
0
cos 2 (nω 0 t)dt = T 0 /2
and
∫
2 T0
sin(nω 0 t) sin(mω 0 t)dt = 1 ∫ T0
{cos((n − m)t − cos((n + m)t)}dt = 0 n ≠ m
T 0 0
T 0 0
and similarly for other combinations of sin’s and cosines.
(b) This follows directly from the previous part since the exponentials can be written in
terms of sines and cosines by Euler’s relation.
3. Compute the following complex quantities in polar form:
Solution
(a) (1 + j) 3 (b) ( √ 3 + j 3 )(1 − j) (c) 2−j(6/√ 3)
2+j(6/ √ 3)
(d) j(1 + j)e jπ/6 (e) ejπ/3 −1
1+j √ 3
(a) (1 + j) = √ 2e jπ/4 and so (1 + j) 3 = 2 3/2 e j3π/4 .
(b) j 3 = −j and so ( √ 3+j 3 )(1−j) = 2e −jθ√ 2e −jπ/4 = 2 3/2 e −j(θ+π/4) where θ = arctan −1 1/ √ 3 =
π/6.
(c) 2 − j(6/ √ 3) = 2(1 − j √ 3) = 2e −jπ/3 . Thus, we have (2e −jπ/3 )/(2e jπ/3 ) = e −j2π/3
(d) √ 2e j11π/12
(e) 1 2 ejπ/3
4. Use Euler’s relation (e jθ = cos(θ) + j sin(θ)) to show that:
(a) sin(θ) sin(φ) = 1 cos(θ − φ) − 1 cos(θ + φ)
2 2
(b) sin(θ + φ) = sin(θ) cos(φ) + cos(θ) sin(φ)
Solution

(a) Since e jθ e jφ = e j(θ+φ) = cos(θ + φ) + j sin(θ + φ) = (cos(θ) + j sin(θ)) · (cos(φ) + j sin(φ))
the result is obtained by first using θ + φ and then θ − φ and equating real parts.
(b) Similar to part (a) except equating complex parts.