On the Generalized Gergonne Point and Beyond - Forum ...

More documents

Recommendations

Info

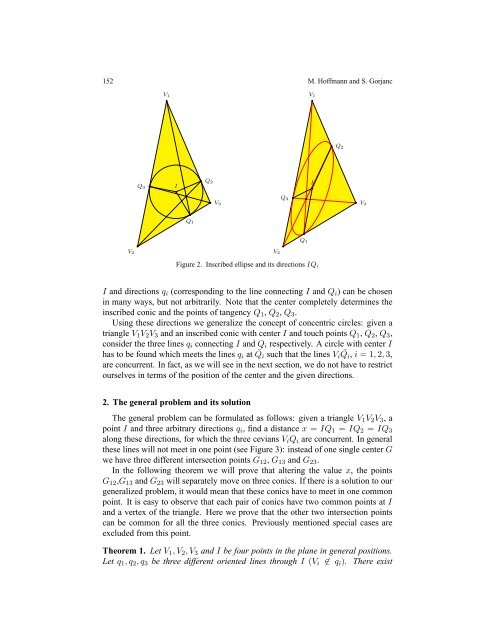
152 M. Hoffmann and S. Gorjanc V 1 V 1 Q 2 Q 2 I Q 3 I Q 3 V 3 Q 1 Q 1 V 2 V 2 V 3 Figure 2. Inscribed ellipse and its directions IQ i I and directions q i (corresponding to the line connecting I and Q i ) can be chosen in many ways, but not arbitrarily. Note that the center completely determines the inscribed conic and the points of tangency Q 1 , Q 2 , Q 3 . Using these directions we generalize the concept of concentric circles: given a triangle V 1 V 2 V 3 and an inscribed conic with center I and touch points Q 1 , Q 2 , Q 3 , consider the three lines q i connecting I and Q i respectively. A circle with center I has to be found which meets the lines q i at ¯Q i such that the lines V i ¯Qi , i = 1,2,3, are concurrent. In fact, as we will see in the next section, we do not have to restrict ourselves in terms of the position of the center and the given directions. 2. The general problem and its solution The general problem can be formulated as follows: given a triangle V 1 V 2 V 3 , a point I and three arbitrary directions q i , find a distance x = IQ 1 = IQ 2 = IQ 3 along these directions, for which the three cevians V i Q i are concurrent. In general these lines will not meet in one point (see Figure 3): instead of one single center G we have three different intersection points G 12 , G 13 and G 23 . In the following theorem we will prove that altering the value x, the points G 12 ,G 13 and G 23 will separately move on three conics. If there is a solution to our generalized problem, it would mean that these conics have to meet in one common point. It is easy to observe that each pair of conics have two common points at I and a vertex of the triangle. Here we prove that the other two intersection points can be common for all the three conics. Previously mentioned special cases are excluded from this point. Theorem 1. Let V 1 ,V 2 ,V 3 and I be four points in the plane in general positions. Let q 1 ,q 2 ,q 3 be three different oriented lines through I (V i ∉ q i ). There exist
On the generalized Gergonne point and beyond 153 V 1 Q 3 G 13 I G 12 G 23 V 2 V 3 Q 1 Q 2 Figure 3. For arbitrary directions and distance, cevians V iQ i are generally not concurrent, but meet at three different points at most two values x ∈ R\{0} such that for points Q i along the lines q i with IQ 1 = IQ 2 = IQ 3 = x, the lines V i Q i are concurrent. Proof. For a real number x and i = 1,2,3, let Q i (x) be a point on q i for which IQ i (x) = x. The correspondences Q i (x) ↔ Q j (x) define perspectivities (q i ) (q j ), (i ≠ j). Now let l i (x) be the line connecting V i and Q i (x). The correspondences l i (x) ↔ l j (x) define projectivities (V i ) ⊼ (V j ), (i ≠ j). The intersection points of corresponding lines of these projectivities lie on three conics: (V 1 ) ⊼ (V 2 ) ⇒ c 3 (V 1 ) ⊼ (V 3 ) ⇒ c 2 (V 2 ) ⊼ (V 3 ) ⇒ c 1 . We find the intersection points of these conics. Since Q i (0) = I, then I ∈ c i ,(i = 1,2,3). V 3 ∈ c 1 ∩ c 2 , V 2 ∈ c 1 ∩ c 3 and V 1 ∈ c 2 ∩ c 3 also hold. Denote the other two intersection points of c 1 and c 2 by S 1 and S 2 , i.e., c 2 ∩ c 3 = {I,V 1 ,S 1 ,S 2 }. The points S 1 and S 2 can be real and distinct, real and identical, or imaginary in pair. (i) If they are real and distinct, then for some x 1 and x 2 , S 1 = l 1 (x 1 ) ∩ l 2 (x 1 ) = l 1 (x 1 ) ∩ l 3 (x 1 ) ⇒ S 1 = l 2 (x 1 ) ∩ l 3 (x 1 ) S 2 = l 1 (x 2 ) ∩ l 2 (x 2 ) = l 1 (x 2 ) ∩ l 3 (x 2 ) ⇒ S 2 = l 2 (x 2 ) ∩ l 3 (x 2 ) which immediately yields S 1 ,S 2 ∈ c 1 as well. (ii) If they are identical, then for the unique x, S = l 1 (x) ∩ l 2 (x) = l 1 (x) ∩ l 3 (x) ⇒ S = l 2 (x) ∩ l 3 (x) which yields S ∈ c 1 .
Page 1: • • Forum Geometricorum Volume
Page 5: On the generalized Gergonne point a

On the Generalized Gergonne Point and Beyond - Forum ...

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?