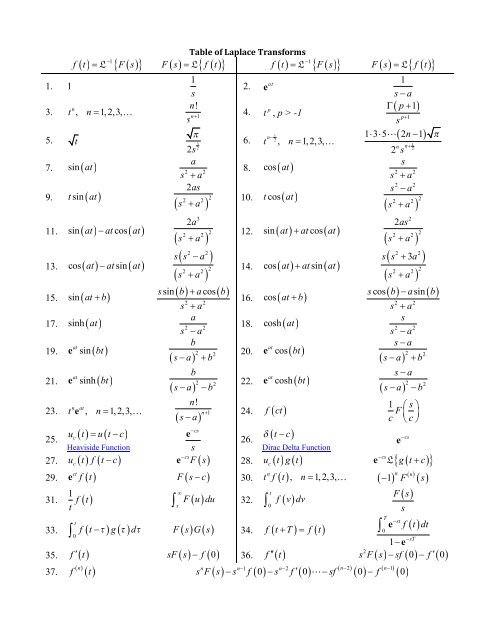
Table of Laplace Transforms

Table of Laplace Transforms
-
f t
f t
-
( ) = L F( s)
F( s) = L ( )
( ) = L F( s)
F( s) = L f ( t)
f t
1 { }
{ }
1. 1
1
s
2.
at
e
3.
n
t , n= 1,2,3, K
n!
n 1
s + 4. p
t , p > -1
5. t 3
2s 2
a
7. sin ( at )
2 2
s + a
2as
9. tsin
( at )
2 2
s + a
11. sin( at) - atcos( at)
13. cos( at) - atsin
( at)
p
( ) 2
2a
3
2 2
2
( s + a )
2 2
s( s -a
)
2 2
2
( s + a )
ssin
15. sin ( at+ b)
( b) + acos( b)
2 2
s + a
a
sinh at
2 2
s - a
b
at
e sin bt
s- a + b
17. ( )
19. ( )
at
21. e sinh ( bt)
23. n at
t e , n= 1,2,3, K
25. u ( t c ) = u ( t - c )
Heaviside Function
( ) 2 2
b
( ) 2 2
s-a -b
n!
n
( s-
a ) + 1
-cs
e
s
-cs F s
6.
t
n-
8. ( )
1 { }
1
2
, n= 1,2,3, K
1
s-
a
G p + 1
( )
p 1
s +
{ }
( n )
135 ⋅ ⋅ L 2 -1
1
2 n n +
s 2
s
cos at
2 2
s + a
2 2
s - a
tcos
at
2 2
s + a
10. ( )
12. sin( at) + atcos( at)
14. cos( at) + atsin
( at)
( )
2as
2
2
2 2
2
( s + a )
2 2
( + 3a
)
2 2
2
( s + a )
s s
scos
16. cos( at+ b)
( b) - asin
( b)
2 2
s + a
s
cosh at
2 2
s - a
s-
a
at
e cos bt
s- a + b
18. ( )
20. ( )
at
22. e cosh ( bt)
24. f ( ct )
d
26.
( t-
c)
Dirac Delta Function
( ) 2 2
s-
a
( ) 2 2
s-a -b
1 Ês
ˆ
F Á ˜
c Ëc¯
-cs
27. uc
( t) f ( t- c)
e ( ) 28. uc
( t) g( t )
e L g( t+
c)
29. ct n
(
e f ( t)
F( s- c)
30. t f ( t), n= 1,2,3, K ( 1)
n n
- F )
( s)
•
t
F
Ú F( u)
du 32. Ú f ( v)
dv
( s)
1
31. f () t
t
s
t
33. Ú f ( t-t) g( t)
dt
F( s) G( s ) 34. f ( t T) f ( t)
0
0
-cs
e
{ }
s
+ =
T
-st
Ú e f () t dt
0
-sT
1-
e
t
sF 2 s -sf 0 - f¢ 0
35. f¢ ( t)
sF( s) - f ( 0)
36. f¢¢ ( )
( ) ( ) ( )
( n
37. ) n n-1 n- 2
( n 2
f ( t )
( ) ( ) ( )
) ( n 1
sF s -s f 0 -s f¢
0 L -sf - ( 0)
- f
- )
( 0)
p

Table Notes
1. This list is not a complete listing of Laplace transforms and only contains some of
the more commonly used Laplace transforms and formulas.
2. Recall the definition of hyperbolic functions.
e + e e -e
cosh() t = sinh () t =
2 2
t -t t -t
3. Be careful when using “normal” trig function vs. hyperbolic functions. The only
difference in the formulas is the “+ a 2 ” for the “normal” trig functions becomes a
“- a 2 ” for the hyperbolic functions!
4. Formula #4 uses the Gamma function which is defined as
If n is a positive integer then,
x t 1
() • - -
G t = Ú e x dx
0
( n )
G + 1 = n!
The Gamma function is an extension of the normal factorial function. Here are a
couple of quick facts for the Gamma function
( p 1) p ( p)
G + = G
Ê1ˆ
G Á ˜=
Ë2¯
( p n)
( p)
G +
p( p+ 1)( p+ 2) L ( p+ n- 1)
= G
p