Lecture notes for Introduction to Representation Theory

More documents

Recommendations

Info

is the rotation by 180 0 around an axis that is perpendicular to two opposite faces, (123) is the rotation around a main diagonal by 120 0 , and (1234) is the rotation by 90 0 around an axis that is perpendicular to two opposite faces; this allows us to compute the traces easily, using the fact that the trace of a rotation by the angle θ in R 3 is 1 + 2 cos θ. Now the character of C 3 − is found by multiplying the character of C 3 by the character of the sign representation. + Finally, we explain how to obtain the character table of A 5 (even permutations of 5 items). The group A 5 is the group of rotations of the regular icosahedron. Thus it has a 3-dimensional “rotation representation” C 3 + , in which (12)(34) is the rotation by 180 0 around an axis perpendicular to two opposite edges, (123) is the rotation by 120 0 around an axis perpendicular to two opposite faces, and (12345), (13254) are the rotations by 72 0 , respectively 144 0 , around axes going through two opposite vertices. The character of this representation is computed from this description in a straightforward way. Another representation of A 5 , which is also 3-dimensional, is C 3 + twisted by the automorphism of A This representation is denoted by C 3 5 given by conjugation by (12) inside S 5 . −. It has the same character as C 3 , except that the conjugacy classes (12345) and (13245) are interchanged. + There are two remaining irreducible representations, and by the sum of squares formula their dimensions are 4 and 5. So we call them C 4 and C 5 . The representation C 4 is realized on the space of functions on the set {1, 2, 3, 4, 5} with zero sum of values, where A 5 acts by permutations (check that it is irreducible!). The character of this representation is equal to the character of the 5-dimensional permutation representation minus the character of the 1-dimensional trivial representation (constant functions). The former at an element g equals to the number of items among 1,2,3,4,5 which are fixed by g. The representation C 5 is realized on the space of functions on pairs of opposite vertices of the icosahedron which has zero sum of values (check that it is irreducible!). The character of this representation is computed similarly to the character of C 4 , or from the orthogonality formula. 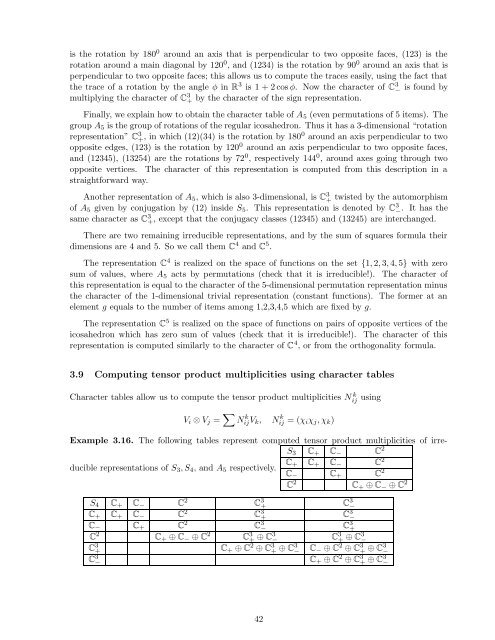3.9 Computing tensor product multiplicities using character tables Character tables allow us to compute the tensor product multiplicities N ij k using V i V j = N ij k V k , N ij k = (ν i ν j , ν k ) Example 3.16. The following tables represent computed tensor product multiplicities of irre S C 2 3 C + C − C − C 2 + C + C ducible representations of S 3 , S 4 , and A 5 respectively. C− C + C 2 C 2 C + C − C 2 S C 2 C 3 C3 4 C + C − + − C 2 C 3 C + C 3 + C + C − − C C + C 2 3 C 3 − C − + C 2 C C2 C3 C3 − + − C C 3 C C3 + + 3 + 3 C 3 + C − C 3 − C C C− 3 + 3 + C 2 C− C 2 − C + C− 3 + C 2 C 3 42
⇒ C C C + 3 C 3 − C 3 − C C− 3 4 C 3 + C C 5 C 3 + C 4 C 5 A 5 C C + 3 C 4 C 5 C 5 C C 5 C 3 + C 4 C 5 C 3 C 4 C 5 C 3 + C 3 + C 4 C 5 + C 3 − C 4 C 5 C 3 − C 4 C 5 − C C4 C 5 C+ 3 C3 − 2C 5 C 4 C C 3 C3 2C 4 2C 5 − C 3 C 3 + C3 + − 3.10 Problems Problem 3.17. Let G be the group of symmetries of a regular N-gon (it has 2N elements). (a) Describe all irreducible complex representations of this group (consider the cases of odd and even N) (b) Let V be the 2-dimensional complex representation of G obtained by complexification of the standard representation on the real plane (the plane of the polygon). Find the decomposition of V V in a direct sum of irreducible representations. Problem 3.18. Let G be the group of 3 by 3 matrices over F p which are upper triangular and have ones on the diagonal, under multiplication (its order is p 3 ). It is called the Heisenberg group. For any complex number z such that z p = 1 we define a representation of G on the space V of complex functions on F p , by ⎧ 1 1 0 (δ0 1 0⎝f)(x) = f(x − 1), 0 0 1 ⎧ 1 0 0 (δ0 1 1⎝f)(x) = z x f(x). 0 0 1 (note that z x makes sense since z p = 1). (a) Show that such a representation exists and is unique, and compute δ(g) for all g G. (b) Denote this representation by R z . Show that R z is irreducible if and only if z ⇒= 1. (c) Classify all 1-dimensional representations of G. Show that R 1 decomposes into a direct sum of 1-dimensional representations, where each of them occurs exactly once. (d) Use (a)-(c) and the “sum of squares” formula to classify all irreducible representations of G. Problem 3.19. Let V be a finite dimensional complex vector space, and GL(V ) be the group of invertible linear transformations of V . Then S n V and Γ m V (m ∗ dim(V )) are representations of GL(V ) in a natural way. Show that they are irreducible representations. Hint: Choose a basis {e i } in V . Find a diagonal element H of GL(V ) such that δ(H) has distinct eigenvalues. (where δ is one of the above representations). This shows that if W is a subrepresentation, then it is spanned by a subset S of a basis of eigenvectors of δ(H). Use the invariance of W under the operators δ(1 + E ij ) (where E ij is defined by E ij e k = ζ jk e i ) for all i = j to show that if the subset S is nonempty, it is necessarily the entire basis. Problem 3.20. Recall that the adjacency matrix of a graph (without multiple edges) is the matrix in which the ij-th entry is 1 if the vertices i and j are connected with an edge, and zero otherwise. Let be a finite graph whose automorphism group is nonabelian. Show that the adjacency matrix of must have repeated eigenvalues. 43
Page 1 and 2: Introduction to representation theo
Page 3 and 4: 4.15 The Frobenius character formul
Page 5 and 6: 1 Basic notions of representation t
Page 7 and 8: 1.2 Algebras Let us now begin a sys
Page 9 and 10: (ii) If V 2 is irreducible, θ is s
Page 11 and 12: 1.5 Quotients Let A be an algebra a
Page 13 and 14: Hint. Show that x p and y p are cen
Page 15 and 16: (b) A = k < x 1 , ..., x m > (the g
Page 17 and 18: 1.10 Tensor products In this subsec
Page 19 and 20: Similarly, if C is another k-algebr
Page 21 and 22: (e) Let N v be the smallest N satis
Page 23 and 24: ⇒ ⇒ 2 General results of repres
Page 25 and 26: y θ(a 1 , . . . , a n ) = a 1 y 1
Page 27 and 28: Example 2.14. 1. Let A = k[x]/(x n
Page 29 and 30: V . This number is called the lengt
Page 31 and 32: Problem 2.24. Let A be an algebra,
Page 33 and 34: 3 Representations of finite groups:
Page 35 and 36: Exercise. Show that if | G | = 0 in
Page 37 and 38: If V, W are representations of G, t
Page 39 and 40: Theorem 3.11. If G is finite, then
Page 41: A 4 Id (123) (132) (12)(34) # 1 4 4
Page 45 and 46: (a) Derive this classification. Hin
Page 47 and 48: 4 Representations of finite groups:
Page 49 and 50: Proof. Let V = C[G] be the regular
Page 51 and 52: Note that any algebraic conjugate o
Page 53 and 54: The proof will be based on the foll
Page 55 and 56: and the action g(f)(x) = f(xg) ⊕g
Page 57 and 58: 1.48). In particular, this understa
Page 59 and 60: For the partition ∂ = (1, ..., 1)
Page 61 and 62: Proof. The proof is obtained easily
Page 63 and 64: Corollary 4.49 easily implies that
Page 65 and 66: Lemma 4.56. Let k be a field of cha
Page 67 and 68: Theorem 4.63. (Weyl character formu
Page 69 and 70: ⇒ ⇒ The goal of this section is
Page 71 and 72: ⇒ 4.24.3 Principal series represe
Page 73 and 74: If ∂ 1 = ⇒ ∂ 2 , let z = xy
Page 75 and 76: Because λ is also a root of unity,
Page 77 and 78: Proof. (i) Let us decompose V = V (
Page 79 and 80: • E 7 : −−−−−−−−
Page 81 and 82: (d) Which groups from Problem 3.24
Page 83 and 84: By identifying V and Y as subspaces
Page 85 and 86: So - considering the first summand
Page 87 and 88: 5.4 Roots From now on, let be a fi
Page 89 and 90: on Z N restricts to the inner produ
Page 91 and 92: 1. Let i Q be a sink. Then either
Page 93 and 94:
Proposition 5.31. Let Q be a quiver
Page 95 and 96:
Theorem 5.34. There is m N, such t
Page 97 and 98:
3) H n : V = C n with basis v i , W
Page 99 and 100:
We also mention that in many exampl
Page 101 and 102:
For this reason in category theory,
Page 103 and 104:
Dictionary between category theory
Page 105 and 106:
Definition 6.21. An abelian categor
Page 107 and 108:
Now, in the general case, we prove
Page 109:
MIT OpenCourseWare http://ocw.mit.e
show all

Lecture notes for Introduction to Representation Theory

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?