The rational Khovanov homology of 3-strand pretzel links

More documents

Recommendations

Info

$MATH 201 - FINAL EXAM Friday January 24 2003, 8:30am Problem 1$

28 ANDREW MANION the orientation chosen). Hence ǫ = l + n − 1. The oriented resolution is a diagram for P(−l,l,n − 1) RL . The skein sequence for a positive crossing is f q 3l+3n−1 t l+n Kh(U 2 ) Kh(P(−l,l,n)) qKh(P(−l,l,n − 1)) Denote the left, middle, and right terms by W, X, and V respectively. W has two exceptional pairs in t = l + n. If n is odd, V has four exceptional pairs (one in t = 0, one in t = 2l, and two in t = l + n − 1), and X has two exceptional pairs (one in t = 0 and one in t = 2l). On the other hand, if n is even, then V has two exceptional pairs (in t = 0 and t = 2l), and X has four exceptional pairs (one in t = 0, one in t = 2l, and two in t = l + n). The map f preserves the q-grading and increases the t-grading by one. 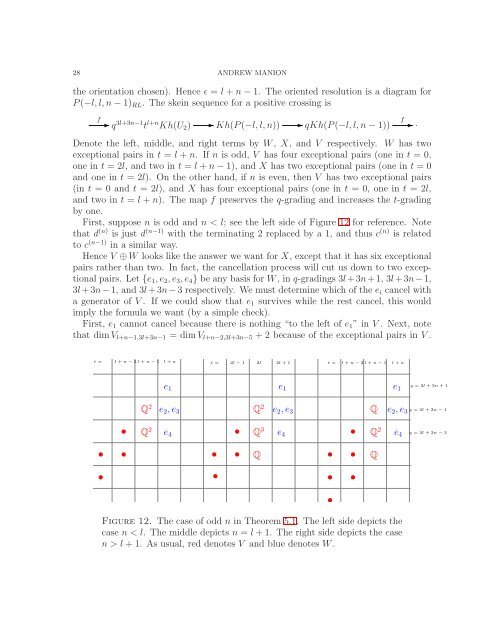First, suppose n is odd and n < l; see the left side of Figure 12 for reference. Note that d (n) is just d (n−1) with the terminating 2 replaced by a 1, and thus c (n) is related to c (n−1) in a similar way. Hence V ⊕ W looks like the answer we want for X, except that it has six exceptional pairs rather than two. In fact, the cancellation process will cut us down to two exceptional pairs. Let {e 1 ,e 2 ,e 3 ,e 4 } be any basis for W, in q-gradings 3l+3n+1, 3l+3n−1, 3l+3n −1, and 3l+3n −3 respectively. We must determine which of the e i cancel with a generator of V . If we could show that e 1 survives while the rest cancel, this would imply the formula we want (by a simple check). First, e 1 cannot cancel because there is nothing “to the left of e 1 ” in V . Next, note that dimV l+n−1,3l+3n−1 = dimV l+n−2,3l+3n−5 + 2 because of the exceptional pairs in V . f . t = l + n−2 l + n−1 l + n t = 2l−1 2l 2l + 1 t = l + n−2 l + n−1 l + n e 1 e 1 e 1 q = 3l + 3n + 1 Q 2 e 2 ,e 3 Q 2 e 2 ,e 3 Q e 2 ,e 3 q = 3l + 3n−1 Q 2 e 4 Q 3 e 4 Q 2 e 4 q = 3l + 3n−3 Q Q Figure 12. The case of odd n in Theorem 5.1. The left side depicts the case n < l. The middle depicts n = l + 1. The right side depicts the case n > l + 1. As usual, red denotes V and blue denotes W.
THE RATIONAL KHOVANOV HOMOLOGY OF 3-STRAND PRETZEL LINKS 29 But we must have dimX l+n−1,3l+3n−1 = dimX l+n−2,3l+3n−5 since X has no exceptional pair in t = l + n − 1 or t = l + n − 2. So both e 2 and e 3 must cancel. Finally, if e 4 did not cancel, then we would have dimX l+n−1,3l+3n−3 = dimX l+n,3l+3n+1 + 1. But we need dim X l+n−1,3l+3n−3 = dimX l+n,3l+3n+1 , again because the only exceptional pairs of X are in t = 0 and t = 2l. Hence e 4 must cancel, and we have proved our formula when n is odd and less than l. The next case for odd n is n = l + 1. The middle of Figure 12 is a reference here. A similar argument implies that e 2 , e 3 , and e 4 all cancel, and a quick check verifies that our results for the lower and upper summands of X agree with Definition 2.5(4) and Definition 2.6(7). If n is odd and n > l + 1, then the right side of Figure 12 depicts the situation. The generator e 1 still cannot cancel. But logic very similar to before implies that e 4 and a one-dimensional subspace of (e 2 ,e 3 ) must cancel, since X has no exceptional pairs in t = l + n. Thus, the lower summand loses an exceptional pair (as predicted by Definition 2.5), and the upper summand turns an exceptional pair into a knight’s move. Finally, suppose n is even; luckily, this case is easier. First, if n < l, we want to show that only e 4 cancels (using the above notation). Again, e 1 cannot cancel. If some combination of e 2 and e 3 cancelled, then we would have dimX l+n−1,3l+3n−1 < dimX l+n−2,3l+3n−5 , an impossibility since X has no exceptional pairs in t = l + n − 2. So both e 2 and e 3 survive. Since n < l, P(−l,l,n) is quasi-alternating by [4], so X l+n,3l+3n−3 = 0 for δ-grading reasons, and e 4 has nowhere to live. The cancellation is responsible for the next (−1) in the sequence d (n) , and e 2 and e 3 are responsible for the (2) following it. On the other hand, if n > l, then X has two exceptional pairs in t = l + n but V l+n = 0, so none of the e i can cancel. If n = l, the same argument holds: X must have three exceptional pairs in t = l + n but dimV l+n is only two, so none of the e i can cancel. Thus e 1 and (say) e 2 add an exceptional pair to the lower summand, and e 3 and e 4 add an exceptional pair to the upper summand. These additions are precisely what we were expecting, completing the inductive argument. □ 5.2. P(−l,l + 1,n) for even l. Now we will make a similar computation for P(−l,l + 1,n) which we will use as the base case in the induction to follow. As with odd l, we do not need to start with n = 0. We can use n = l instead; since P(−l,l + 1,l) = P(−l,l,l + 1), the previous section tells us the formula for Kh(P(−l,l + 1,l)). Lemma 5.2. For n ≥ l+1, Kh(P(−l,l+1,n)) is given by the formula in Theorem 2.9. Proof. Resolve the top crossing on the rightmost strand, a positive crossing. Our diagram for P(−l,l + 1,n) has no negative crossings, while the unoriented resolution (a diagram for the unknot) has l + n − 1 negative crossings. The oriented resolution is a diagram for P(−l,l + 1,n − 1). Hence the skein sequence is f q 3l+3n−1 t l+n Kh(U) Kh(P(−l,l + 1,n)) qKh(P(−l,l + 1,n − 1)) f ,
Page 1 and 2: THE RATIONAL KHOVANOV HOMOLOGY OF 3
Page 25 and 26: skein sequence is f THE RATIONAL KH
Page 27: THE RATIONAL KHOVANOV HOMOLOGY OF 3
Page 33: THE RATIONAL KHOVANOV HOMOLOGY OF 3

The rational Khovanov homology of 3-strand pretzel links

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?