MAS107 Control Theory Exam Solutions

MAS107 CONTROL THEORY 1. HOVLAND: EXAM SOLUTION 2008 1
MAS107 Control Theory
Exam Solutions 2008
Geir Hovland, Mechatronics Group, Grimstad, Norway
June 30, 2008
C. Repeat question 1B, but plot the phase curve instead of the
gain.
I. BODE PLOT
A. Write down the zeroes and poles of the following transfer
function
0.1s
G(s) =
(1 + s
10
)(1 + s
2 10
) 3
This transfer function consists of four parts
• The gain constant 0.1
• The derivator s, which is equal to a zero at location s = 0
• The pole (1 + s
10
), which is equal to a pole at s =
2
−10 2 = −100
• The pole (1 + s
10
), which is equal to a pole at s =
3
−10 3 = −1000
B. Draw the asymptotic Bode plots (only gain) for each
component of the transfer function above. Use frequencies
from 10 0 to 10 5 rad/sec. Add all the components together to get
an asymptotic approximation for the Bode plot of G(s). Finally,
draw a more accurate Bode-plot based on the asymptotic
approximation.
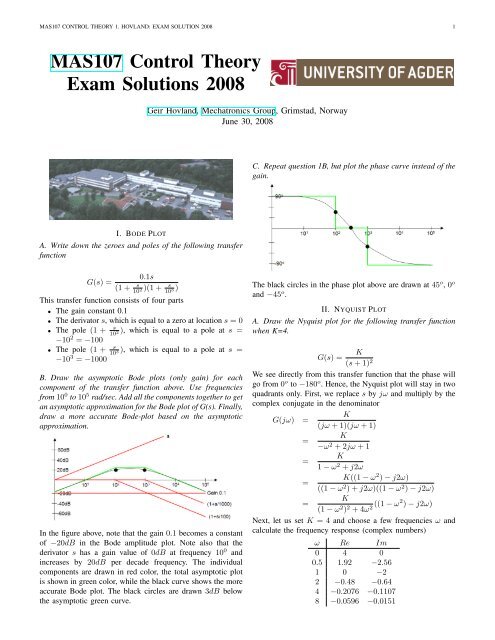
In the figure above, note that the gain 0.1 becomes a constant
of −20dB in the Bode amplitude plot. Note also that the
derivator s has a gain value of 0dB at frequency 10 0 and
increases by 20dB per decade frequency. The individual
components are drawn in red color, the total asymptotic plot
is shown in green color, while the black curve shows the more
accurate Bode plot. The black circles are drawn 3dB below
the asymptotic green curve.
The black circles in the phase plot above are drawn at 45 o , 0 o
and −45 o .
II. NYQUIST PLOT
A. Draw the Nyquist plot for the following transfer function
when K=4.
K
G(s) =
(s + 1) 2
We see directly from this transfer function that the phase will
go from 0 o to −180 o . Hence, the Nyquist plot will stay in two
quadrants only. First, we replace s by jω and multiply by the
complex conjugate in the denominator
G(jω) =
K
(jω + 1)(jω + 1)
=
K
−ω 2 + 2jω + 1
=
K
1 − ω 2 + j2ω
=
K((1 − ω 2 ) − j2ω)
((1 − ω 2 ) + j2ω)((1 − ω 2 ) − j2ω)
=
K
(1 − ω 2 ) 2 + 4ω 2 ((1 − ω2 ) − j2ω)
Next, let us set K = 4 and choose a few frequencies ω and
calculate the frequency response (complex numbers)
ω Re Im
0 4 0
0.5 1.92 −2.56
1 0 −2
2 −0.48 −0.64
4 −0.2076 −0.1107
8 −0.0596 −0.0151

MAS107 CONTROL THEORY 1. HOVLAND: EXAM SOLUTION 2008 2
This gives us approximately the following Nyquist plot, where
the red curve is from the table above, while the green curve
is the mirror of the red curve about the real axis.
D. Based on the Nyquist plot from question 2A, estimate the
phase margin graphically (no calculations required).
B. Calculate amplitude and phase for the system in question
2A at the following frequencies: 0.5, 1, 2 and 4 rad/sec.
The amplitude A in decibel and phase P are given by the
following formulas.
(√ )
A = 20log 10 Re(G(jω))2 + Im(G(jω)) 2
( )
Im(G(jω))
P = atan
Re(G(jω))
We then get the following results
ω Re Im A P
0.5 1.92 −2.56 10.1 −53.1 o
1 0 −2 6.02 −90 o
2 −0.48 −0.64 −1.94 −126.9 o
4 −0.2076 −0.1107 −12.6 −151.9 o
C. Is the system in question 2A stable for a unity-feedback
controller ?
Yes, the system is stable because N = 0 (the number of
encirclements of -1) and P = 0 (the number of open-loop
unstable poles). The Nyquist criterion says Z = P − N = 0,
which is stable when Z = 0.
A unit circle is drawn in the Nyquist plot, see the figure
above. The phase margin is given by the angle between the
negative real axis and the cross-point between the unit circle
and the Nyquist curve. From the figure this seems to occur
approximately at the black dot representing the frequency
ω = 2. However, the table in question 2B says that the
amplitude A = −1.92dB at ω = 2. Hence, the Nyquist curve
will cross the unit circle slightly before ω = 2 (remember that
an amplitude of 0dB represents the unit circle). The phase
margin at ω = 2 equals 180 o − 126.9 o = 53.1 o . Since the
cross-point occurs slightly before ω = 2, the phase margin is
estimated to be approximately 60 o .
III. TIME AND FREQUENCY RESPONSE
Given the control system in the figure below with the
following plant model:
K
G(s) =
s(s + √ 2K)
A. Describe a method to analytically calculate percentage
overshoot and settling time when a unit step is applied to
the reference input R(s). Hint: Open/closed transfer functions.
First, the closed-loop transfer function is calculated.
G(s)
1 + G(s)
=
=
=
K
s(s+ √ 2K)
K
1 +
s(s+ √ 2K)
K
s 2 + √ 2Ks + K
ω 2
s 2 + 2ζωs + ω 2

MAS107 CONTROL THEORY 1. HOVLAND: EXAM SOLUTION 2008 3
From the equations above we see that the natural frequency ω
and the damping factor ζ equal
ω = √ √
K
√
2K 2
ζ =
2ω = 2 = 0.707
From the exam formula sheet, we then have the following
relationships between percentage overshoot and settling time.
√
ζ 2 π 2
%OS = 100/exp
T s = 4
ζω = 5.66 √
K
1−ζ 2 = 4.32
B. When a settling time less than 1.0 second is required,
calculate the range of values for the parameter K.
T s = 4
ζω = 5.66 √
K
( ) 2 5.66
K =
= 32
T s
Hence, K must be equal to 32 or larger to achieve a settling
time of 1.0 seconds or less.
C. When a peak time less than 0.2 second is required, calculate
the range of values for the parameter K.
The formula for peak time was specified as follows.
T p =
K =
π
ω √ 1 − ζ = 4.44 √ 2 K
( ) 2 4.44
= 492.8 (1)
T p
Hence, K must be equal to 492.8 or larger to achieve a peak
time of 0.2 seconds or less.
as there is a steady-state control error. Hence, this type of
controller is typically used to achieve zero steady-state error
for Type 0 systems when a step is applied as the reference
input. The integral action appears at low frequencies in the
Bode plot, and hence the PI-controller can be tuned such that
it does not worsen bandwidth performance or stability margins.
PID-Controller: The PID-controller is the same as the PIcontroller
with an added parameter T d which is the derivative
time constant. The derivative action reacts on changes in the
control error. Hence, the derivative action prevents the system
response to change too rapidly. A mechanical equivalent of the
derivative action is a damper mechanism. In the Bode plot, the
derivative action increases both the amplitude and the phase.
Hence, the derivative action can be used to improve system
bandwidth as well as stability margins.
Lag-Compensator: The lag compensator is very similar to
the PI-controller. The main difference is the low frequency
characteristics. While the PI-controller goes to infinity gain
at low frequencies, the lag compensator has a limited gain
at low frequencies. Hence, the lag compensator can reduce
steady-state errors, but not completely remove them.
Lead-Compensator: The lead compensator is very similar
to a PD controller. The main difference is the high frequency
characteristics. While the PD controller goes to infinity gain
at high frequencies, the lead compensator has a limited gain
at high frequencies. This limited gain is a benefit in systems
which contain high frequency measurement noise.
B. The Nyquist curve for a system crosses the real axis at -2,
circles the point -1 once and the system is stable in closed
loop. How many poles in the right half plane does the system
have? Explain your answer.
IV. SYSTEM UNDERSTANDING
A. Explain the properties of the following controllers: P, PI,
PID, lag and lead compensator. Write maximum one page.
P-Controller: The P-controller is the simplest controller
and contains a single parameter usually denoted K p . This
parameter affects only the gain of the system, while the total
phase is unaffected. Hence, a typical method to tune this
controller is to find the frequency ω c where the desired phase
margin in the phase plot occurs, and then adjust the gain
parameter K p such that the amplitude curve crosses the 0dB
line at the same frequency ω c . Systems which are open-loop
stable (no poles in the right half-plane) have an upper limit on
the gain parameter K p for stability, while systems which are
open-loop unstable have a lower limit on the gain parameter.
The P-controller can typically improve system properties such
as rise time, settling time, peak time and steady-state error.
These improvements usually result in a worse performance in
terms of percentage overshoot (stability margin).
PI-Controller: The PI-controller is the same as the P-
controller with an added parameter T i which is the integral
time constant. The integral action will never settle as long
Let us use the Nyquist curve above as a concrete example for
this problem. The Nyquist curve above is a perfect circle with
a radius of 2 and is generated by the following system
G(s) =
2(s + 1)
s − 1

MAS107 CONTROL THEORY 1. HOVLAND: EXAM SOLUTION 2008 4
The Nyquist curve crosses the real axis at -2, the curve circles C. Design a lag compensator for the system such that the
the point -1 once. The problem text states that the system is steady-state error for a ramp is reduced by a factor of 10
stable in closed-loop. Hence, Z = P − N = 0. Since N = 1, without influencing the system’s bandwidth to a large degree.
we need also that P = 1. In other words, the open-loop system The Bode plot of the open-loop transfer function is given
has one pole in the right half-plane. We can also see that the below.
example transfer function above also has a single pole in the
right half-plane (at s = 1).
C. For the system in question 4B two different P-controllers
are applied. The first P-controller has a gain of 2.0, while the
other controller has a gain of 0.4. Are the two new control
systems stable or unstable. Explain your answers.
The first P-controller will double the radius of the example
circle to 4. The Nyquist curve will still encircle the point
−1 once. Hence, the control system will be stable. The P-
controller with gain 0.4 will change the radius of the example
circle to 0.8. The Nyquist curve will no longer encircle the
point −1. Hence, the P-controller with a gain of 0.4 will be
unstable for this system. Note, that this system behavior is the
opposite of what we see for an open-loop stable system. For
an open-loop unstable system, a large P-controller gain will
First, we multiply the system with the gain of 10. From the
stabilize the system, while small P-controller gains will reduce
Bode plot we see that the cross-over frequency w c = 10 −1 =
the stability margins.
0.1. Hence, to make sure that we do not influence the system
V. STEADY-STATE ERRORS AND COMPENSATOR bandwidth to a large degree, we choose the zero of the lag
compensator equal to 0.01, ie. 1 T
= 0.01 or T = 100. The high
frequency gain of the lag compensator needs to compensate for
1
the initial multiplication of 10. Hence, α = 10, or
αT = 0.001.
The lag compensator and the initial gain of 10 are summarized
as follows and illustrated in the figure below. Note from the
figure that the net gain of the lag compensator and the gain
G p (s) = 0.2
of 10 equals 0dB at high frequencies. This is an important
characteristic too avoid affecting the overall system bandwidth
s(s + 2)
as specified in the problem definition.
The controller equals G c = 10.
A. Calculate the steady-state error when applying a unity step
G c (s) = 10 s + 1 T
α s + 1
αT
at the input R(s).
The system above is a Type 1 system (because of the
= s + 0.01
s + 0.001
integrator). Hence, the steady-state error for a unity step is
equal to 0.
B. Calculate the steady-state error when applying a ramp at
the input R(s).
The control error equals
1
e(s) =
1 + G c (s)G p (s) = 1
1 + 2
s(s+2)
s(s + 2)
=
s(s + 2) + 2
Now we can use the final value theorem to determine the D. Keep the lag compensator from question 5C and add a
steady-state error (knowing that the Laplace transform of a lead compensator such that the bandwidth is increased by a
ramp equals 1 s
). 2 factor 3.
[ ]
1
lim
s→0 s 2 · s · s(s + 2)
We want to increase the cross-over frequency from ω
s(s + 2) + 2
c = 0.1
[
]
s + 2
lim
= 2 to ω c = 0.3. From the Bode plot in Question 5C, we see
s→0 s(s + 2) + 2 2 = 1 that the amplitude at ω c = 0.3 equals approximately −10dB.
Hence, the lead compensator must contribute with a gain of

MAS107 CONTROL THEORY 1. HOVLAND: EXAM SOLUTION 2008 5
10dB (or 3.16) at ω c . From the formula sheet, we have
|G c (jω c )| =
β =
1
√ β
= 3.16
( ) 2 1
= 0.1
3.16
The formula sheet gives us the final parameter T of the lead
compensator.
ω c =
T =
G c (s) = 1 β
1
T √ β
1 1
√ =
ω c β 0.3 · 0.01 = 10.54
s + 1 T
s + 1
βT
=
10(s + 0.095)
s + 0.95
From the Bode plots below, we see that the lead compensator
raises the gain with 10dB at ω c = 0.3 and that the phase has
its maximum phase lift at the same frequency.
Taking the Laplace transform and inserting m = 1, d = 1, we
can find the transfer function from f(t) to x(t).
x(s 2 + s) = f(s)
x
f (s) = 1
s 2 + 2
B. Calculate the transfer function from the battery voltage to
the current in the figure below.
V (t) = Ri(t) + 1 C
∫
i(t)dt
VI. MODELLING
A. Calculate the transfer function from the force f(t) to the
position x(t) for the mechanical system in the figure below.
Taking Laplace, we get the following transfer function
The spring has no effect, since the force f(t) is the same on
both sides of the spring (Newton’s 1st law). The differential
equation which describes the motion of the mass are given
below.
mẍ(t) = f(t) − dẋ(t)
(2)
V (s) = Ri(s) + 1
Cs i(s)
(
= i(s) R + 1 )
Cs
= i(s) RCs + 1
Cs
i
V (s) = Cs
RCs + 1 = 0.5s
0.5s + 1

MAS107 CONTROL THEORY 1. HOVLAND: EXAM SOLUTION 2008 6
C. The figure below shows two watertanks. The height in tank
1 is h 1 (metre) while the height in tank 2 is h 2 . The area of
tank 1 and 2 are A 1 and A 2 (m 2 ), respectively. The inflow to
tank 1 is u (m 3 /sec) while the outflow is k 1 h 1 . The inflow to
tank 2 is k 1 h 1 , while the outflow is k 2 h 2 . Write down the two
differential equations which describe the system. Based on the
differential equations, write down the transfer function from
u(s) to h2(s).
A 1
h 1
dt
h 2
A 2
dt
Taking Laplace, we get
= u − k 1 h 1
= k 1 h 1 − k 2 h 2
A 1 sh 1 = u − k 1 h 1
h 1 (A 1 s + k 1 ) = u
h 1 =
1
u
A 1 s + k 1
A 2 sh 2 = k 1 h 1 − k 2 h 2
k 1
h 2 (A 2 s + k 2 ) = k 1 h 1 = u
(
A 1 s
)
+
(
k 1
)
h 2
u (s) = 1
k 1
A 2 s + k 2 A 1 s + k 1