THESE de DOCTORAT - cerfacs

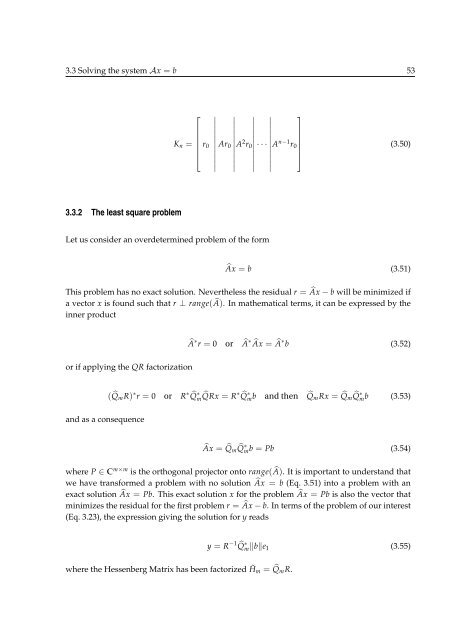
3.3 Solving the system Ax = b 53
⎡ ∣ ∣ ∣ ⎤
∣∣∣∣∣∣∣∣∣∣ ∣∣∣∣∣∣∣∣∣∣ ∣∣∣∣∣∣∣∣∣∣ K n =
r
⎢ 0 Ar 0 A 2 r 0 · · ·
A n−1 r 0 ⎥
⎣
⎦
∣
(3.50)
3.3.2 The least square problem
Let us consider an overdetermined problem of the form
Âx = b (3.51)
This problem has no exact solution. Nevertheless the residual r = Âx − b will be minimized if
a vector x is found such that r ⊥ range(Â). In mathematical terms, it can be expressed by the
inner product
 ∗ r = 0 or  ∗ Âx =  ∗ b (3.52)
or if applying the QR factorization
( ̂Q m R) ∗ r = 0 or R ∗ ̂Q ∗ m ̂QRx = R ∗ ̂Q ∗ mb and then ̂Q m Rx = ̂Q m ̂Q ∗ mb (3.53)
and as a consequence
Âx = ̂Q m ̂Q ∗ mb = Pb (3.54)
where P ∈ C m×m is the orthogonal projector onto range(Â). It is important to understand that
we have transformed a problem with no solution Âx = b (Eq. 3.51) into a problem with an
exact solution Âx = Pb. This exact solution x for the problem Âx = Pb is also the vector that
minimizes the residual for the first problem r = Âx − b. In terms of the problem of our interest
(Eq. 3.23), the expression giving the solution for y reads
y = R −1 ̂Q ∗ m‖b‖e 1 (3.55)
where the Hessenberg Matrix has been factorized ˜H m = ̂Q m R.