THESE de DOCTORAT - cerfacs

108 Chapter 6: Boundary conditions for low Mach number acoustic codes
evaluate the acoustic response of a system due to changes in the mean flow caused by section
variations, but to study the influence of an entropy variation on the acoustics of a given system.
Hence, a thin one-dimensional flame will be considered. This flame, which is characterized by
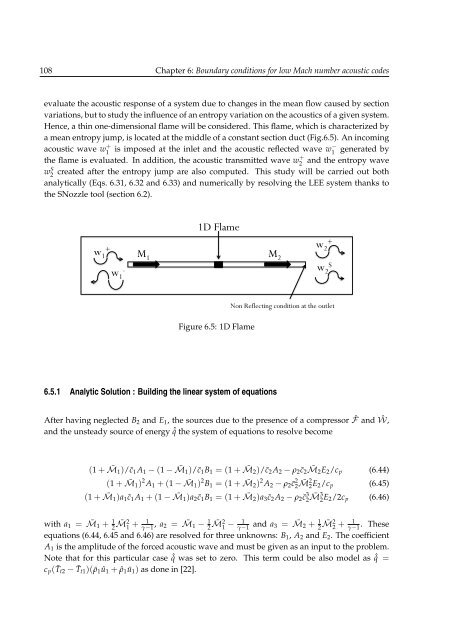
a mean entropy jump, is located at the middle of a constant section duct (Fig.6.5). An incoming
acoustic wave w + 1 is imposed at the inlet and the acoustic reflected wave w− 1
generated by
the flame is evaluated. In addition, the acoustic transmitted wave w + 2
and the entropy wave
w2 S created after the entropy jump are also computed. This study will be carried out both
analytically (Eqs. 6.31, 6.32 and 6.33) and numerically by resolving the LEE system thanks to
the SNozzle tool (section 6.2).
1D Flame
w 1
+
w 1
-
M 1
M 2
w 2
+
w 2
S
Figure 6.5: 1D Flame
Non Reflecting condition at the outlet
6.5.1 Analytic Solution : Building the linear system of equations
After having neglected B 2 and E 1 , the sources due to the presence of a compressor ˆ F and Ŵ,
and the unsteady source of energy ˆ˙q the system of equations to resolve become
(1 + ¯M 1 )/ ¯c 1 A 1 − (1 − ¯M 1 )/ ¯c 1 B 1 = (1 + ¯M 2 )/ ¯c 2 A 2 − ρ 2 ¯c 2 ¯M 2 E 2 /c p (6.44)
(1 + ¯M 1 ) 2 A 1 + (1 − ¯M 1 ) 2 B 1 = (1 + ¯M 2 ) 2 A 2 − ρ 2 ¯c 2 2 ¯M 2 2 E 2/c p (6.45)
(1 + ¯M 1 )a 1 ¯c 1 A 1 + (1 − ¯M 1 )a 2 ¯c 1 B 1 = (1 + ¯M 2 )a 3 ¯c 2 A 2 − ρ 2 ¯c 3 2 ¯M 3 2 E 2/2c p (6.46)
with a 1 = ¯M 1 + 1 2 ¯M 2 1 + 1
γ−1 , a 2 = ¯M 1 − 1 2 ¯M 2 1 − 1
γ−1 and a 3 = ¯M 2 + 1 2 ¯M 2 2 + 1
γ−1 . These
equations (6.44, 6.45 and 6.46) are resolved for three unknowns: B 1 , A 2 and E 2 . The coefficient
A 1 is the amplitude of the forced acoustic wave and must be given as an input to the problem.
Note that for this particular case ˆ˙q was set to zero. This term could be also model as ˆ˙q =
c p ( ¯T t2 − ¯T t1 )( ¯ρ 1 û 1 + ˆρ 1 ū 1 ) as done in [22].