THESE de DOCTORAT - cerfacs

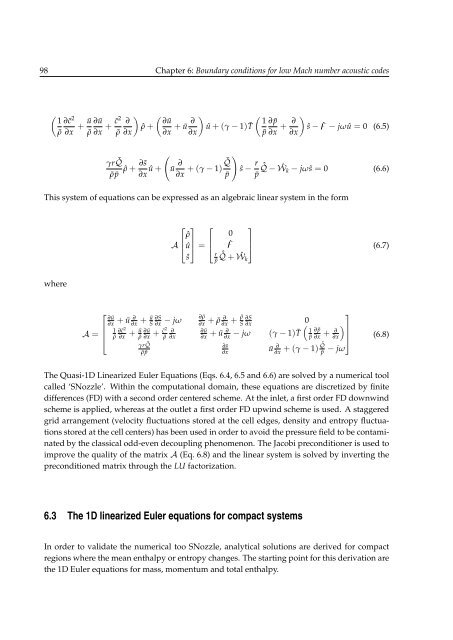
98 Chapter 6: Boundary conditions for low Mach number acoustic codes
( 1¯ρ
∂ ¯c 2
∂x + ū ∂ū
¯ρ ∂x + ¯c2¯ρ
γr ¯˙Q
¯ρ ¯p
) (
∂ ∂ū
ˆρ +
∂x ∂x + ū ∂ )
(
∂ ¯p
û + (γ − 1) ¯T
∂x
1¯p ∂x + ∂ )
ŝ − ˆϜ − jωû = 0 (6.5)
∂x
(
)
∂¯s ˆρ +
∂x û + ū ∂
¯˙Q
∂x + (γ − 1) ŝ − r¯p ¯p
ˆ˙Q − ˆ W k − jωŝ = 0 (6.6)
This system of equations can be expressed as an algebraic linear system in the form
⎡ ⎤ ⎡ ⎤
ˆρ 0
⎢ ⎥ ⎢
A ⎣û⎦ = ⎣ ˆϜ
⎥
⎦ (6.7)
ŝ
r¯p
ˆ˙Q + Wˆ
k
where
⎡
∂ū
∂x + ū ∂
A = ⎢
⎣
1¯ρ
∂ ¯c 2
∂x + ū¯ρ
∂x + ū ∂S
S
∂ū
∂x + ¯c2¯ρ
γr ¯˙Q
¯ρ ¯p
∂x − jω
∂
∂x
∂ ¯ρ
∂x + ¯ρ ∂
∂x + ¯ρ S
∂ū
∂x + ū ∂
∂x − jω
∂¯s
∂x
∂S
∂x
0
(γ − 1) ¯T
(
1¯p
∂ ¯p
∂x + ∂
∂x
ū ∂
∂x + (γ − 1) ¯˙Q¯p − jω
⎤
)
⎥
⎦ (6.8)
The Quasi-1D Linearized Euler Equations (Eqs. 6.4, 6.5 and 6.6) are solved by a numerical tool
called ‘SNozzle’. Within the computational domain, these equations are discretized by finite
differences (FD) with a second order centered scheme. At the inlet, a first order FD downwind
scheme is applied, whereas at the outlet a first order FD upwind scheme is used. A staggered
grid arrangement (velocity fluctuations stored at the cell edges, density and entropy fluctuations
stored at the cell centers) has been used in order to avoid the pressure field to be contaminated
by the classical odd-even decoupling phenomenon. The Jacobi preconditioner is used to
improve the quality of the matrix A (Eq. 6.8) and the linear system is solved by inverting the
preconditioned matrix through the LU factorization.
6.3 The 1D linearized Euler equations for compact systems
In order to validate the numerical too SNozzle, analytical solutions are derived for compact
regions where the mean enthalpy or entropy changes. The starting point for this derivation are
the 1D Euler equations for mass, momentum and total enthalpy.