Notes on Relativity and Cosmology - Physics Department, UCSB
Share Embed Flag
Download ePaper

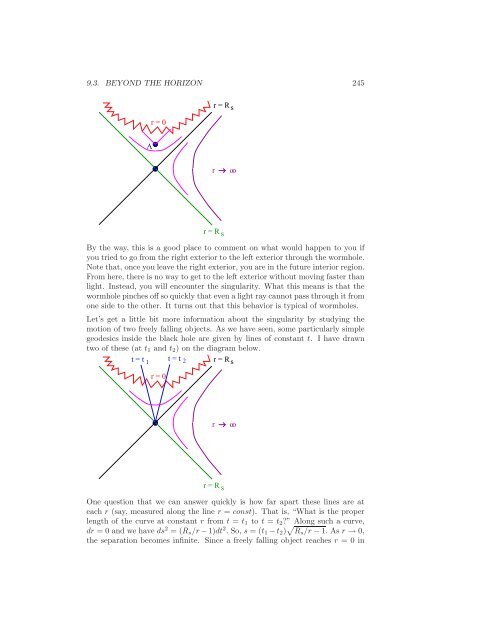
Notes on Relativity and Cosmology - Physics Department, UCSB

Notes on Relativity and Cosmology - Physics Department, UCSB Notes on Relativity and Cosmology - Physics Department, UCSB

web.physics.ucsb.edu
from web.physics.ucsb.edu More from this publisher
27.09.2014 Views

244 CHAPTER 9. BLACK HOLES Well, our diagram is exactly the same on the right as on the left, so there seems to be a symmetry. In fact, you can check that the Schwarzschild metric is unchanged if we replace t by −t. So, both directions must behave identically. If any calculation found that the worldline bends to the left, then there would be an equally valid calculation showing that the worldline bends to the right. As a result, the freely falling worldline will not bend in either direction and will remain at a constant value of t. Now, how long does it take to reach r = 0? We can compute the proper time by using the freely falling worldline with dt = 0. For such a worldline the metric yields: dτ 2 = −ds 2 = dr 2 R s /r − 1 = r R s − r dr2 . (9.18) Integrating, we have: τ = √ r dr R s R s − r . (9.19) ∫ 0 It is not important to compute this answer exactly. What is important is to notice that the answer is finite. We can see this from the fact that, near r ≈ R s the integral is much like √ dx x near x = 0. This latter integral integrates to √ x and is finite at x = 0. Also, near r = 0 the integral is much like x R s dx, which clearly gives a finite result. Thus, our observer measures a finite proper time between r = R s and r = 0 and the throat does collapse to zero size in finite time. 9.3.2 The Singularity This means that we should draw the line r = 0 as one of the hyperbolae on our digram. It is clearly going to be a ‘rather singular line’ (to paraphrase Sherlock Holmes again), and we will mark it as special by using a jagged line. As you can see, this line is spacelike and so represents a certain time. We call this line the singularity. Note that this means that the singularity of a black hole is not a place at all! The singularity is most properly thought of as being a very special time, at which the entire interior of the black hole squashes itself (and everything in it) to zero size. Note that, since it cuts all of the way across the future light cone of any events in the interior (such as event A below), there is no way for any object in the interior to avoid the singularity.

9.3. BEYOND THE HORIZON 245 r = 0 r = R s A r oo r = R s By the way, this is a good place to comment on what would happen to you if you tried to go from the right exterior to the left exterior through the wormhole. Note that, once you leave the right exterior, you are in the future interior region. From here, there is no way to get to the left exterior without moving faster than light. Instead, you will encounter the singularity. What this means is that the wormhole pinches off so quickly that even a light ray cannot pass through it from one side to the other. It turns out that this behavior is typical of wormholes. Let’s get a little bit more information about the singularity by studying the motion of two freely falling objects. As we have seen, some particularly simple geodesics inside the black hole are given by lines of constant t. I have drawn two of these (at t 1 and t 2 ) on the diagram below. t = t 1 t = t 2 r = R s r = 0 r oo r = R s One question that we can answer quickly is how far apart these lines are at each r (say, measured along the line r = const). That is, “What is the proper length of the curve at constant r from t = t 1 to t = t 2 ?” Along such a curve, dr = 0 and we have ds 2 = (R s /r −1)dt 2 . So, s = (t 1 −t 2 ) √ R s /r − 1. As r → 0, the separation becomes infinite. Since a freely falling object reaches r = 0 in

9.3. BEYOND THE HORIZON 245<br />

r = 0<br />

r = R s<br />

A<br />

r<br />

oo<br />

r = R s<br />

By the way, this is a good place to comment <strong>on</strong> what would happen to you if<br />

you tried to go from the right exterior to the left exterior through the wormhole.<br />

Note that, <strong>on</strong>ce you leave the right exterior, you are in the future interior regi<strong>on</strong>.<br />

From here, there is no way to get to the left exterior without moving faster than<br />

light. Instead, you will encounter the singularity. What this means is that the<br />

wormhole pinches off so quickly that even a light ray cannot pass through it from<br />

<strong>on</strong>e side to the other. It turns out that this behavior is typical of wormholes.<br />

Let’s get a little bit more informati<strong>on</strong> about the singularity by studying the<br />

moti<strong>on</strong> of two freely falling objects. As we have seen, some particularly simple<br />

geodesics inside the black hole are given by lines of c<strong>on</strong>stant t. I have drawn<br />

two of these (at t 1 <strong>and</strong> t 2 ) <strong>on</strong> the diagram below.<br />

t = t 1<br />

t = t 2 r = R s<br />

r = 0<br />

r<br />

oo<br />

r = R s<br />

One questi<strong>on</strong> that we can answer quickly is how far apart these lines are at<br />

each r (say, measured al<strong>on</strong>g the line r = c<strong>on</strong>st). That is, “What is the proper<br />

length of the curve at c<strong>on</strong>stant r from t = t 1 to t = t 2 ?” Al<strong>on</strong>g such a curve,<br />

dr = 0 <strong>and</strong> we have ds 2 = (R s /r −1)dt 2 . So, s = (t 1 −t 2 ) √ R s /r − 1. As r → 0,<br />

the separati<strong>on</strong> becomes infinite. Since a freely falling object reaches r = 0 in

logo

products

Resources

Company

Terms of service
Privacy policy
Cookie policy
Cookie settings
Imprint
Change language
Made with love in Switzerland
© 2024 Yumpu.com all rights reserved

Hooray! Your file is uploaded and ready to be published.

Saved successfully!

Ooh no, something went wrong!