Notes on Relativity and Cosmology - Physics Department, UCSB

224 CHAPTER 8. GENERAL RELATIVITY AND CURVED SPACETIME
a geodesic. For this part, consider only triangles which can be shrunk
down to zero size without taking the triangle out of the surface. (For
example, the triangle should not loop all the way around the hole in
the cylinder, cone, or double funnel below.)
c) Do initially parallel ‘geodesics’ bend toward each other, bend away from
each other, or remain parallel? Important note: The phrase initially
parallel is important here. Certainly, two geodesics that start out pointing
toward each other will move toward each other, and similarly for
geodesics that initially point apart. What exactly do I mean by initially
parallel, you may ask? Suppose that two geodesics are both orthogonal
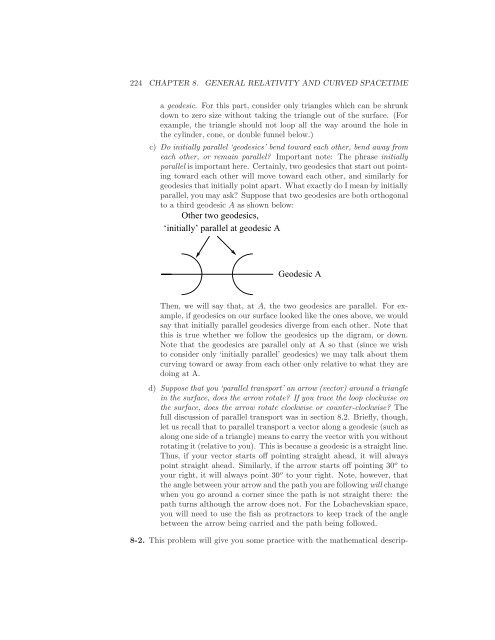
to a third geodesic A as shown below:
Other two geodesics,
‘initially’ parallel at geodesic A
Geodesic A
Then, we will say that, at A, the two geodesics are parallel. For example,
if geodesics on our surface looked like the ones above, we would
say that initially parallel geodesics diverge from each other. Note that
this is true whether we follow the geodesics up the digram, or down.
Note that the geodesics are parallel only at A so that (since we wish
to consider only ‘initially parallel’ geodesics) we may talk about them
curving toward or away from each other only relative to what they are
doing at A.
d) Suppose that you ‘parallel transport’ an arrow (vector) around a triangle
in the surface, does the arrow rotate? If you trace the loop clockwise on
the surface, does the arrow rotate clockwise or counter-clockwise? The
full discussion of parallel transport was in section 8.2. Briefly, though,
let us recall that to parallel transport a vector along a geodesic (such as
along one side of a triangle) means to carry the vector with you without
rotating it (relative to you). This is because a geodesic is a straight line.
Thus, if your vector starts off pointing straight ahead, it will always
point straight ahead. Similarly, if the arrow starts off pointing 30 o to
your right, it will always point 30 o to your right. Note, however, that
the angle between your arrow and the path you are following will change
when you go around a corner since the path is not straight there: the
path turns although the arrow does not. For the Lobachevskian space,
you will need to use the fish as protractors to keep track of the angle
between the arrow being carried and the path being followed.
8-2. This problem will give you some practice with the mathematical descrip-