Notes on Relativity and Cosmology - Physics Department, UCSB

206 CHAPTER 8. GENERAL RELATIVITY AND CURVED SPACETIME
Now, there are several ways to discuss curvature. We are used to looking at
curved spaces inside of some larger (flat) space. Einstein’s idea was that the
only relevant things are those that can be measured in terms of the curved
surface itself and which have nothing to do with it (perhaps) being part of some
larger flat space. As a result, one would gain nothing by assuming that there is
such a larger flat space. In Einstein’s theory, there is no reason to suppose that
one exists.
Our task for this section is to learn how to describe this in a useful way. For
example, we noticed above that this new understanding of gravity means that
the gravitational field contains more information than just giving an acceleration
at various points in spacetime. The acceleration is related to curvature in
spacetime associated with a time direction (say, in the xt plane), but there are
also parts of the gravitational field associated with the (purely spatial) xy, xz,
and yz planes.
Let’s begin by thinking back to the flat spacetime case (special relativity). What
was the object which encoded the flat Minkowskian geometry? It was the interval:
(interval) 2 = −c 2 ∆t 2 + ∆x 2 . This is a special case of something known
as a ‘metric,’ which we will explore further in the rest of this section.
8.3.1 Building Intuition in flat space
To understand fully what information is contained in the interval, it is perhaps
even better to think first about flat space, for which the analogous quantity is
the distance ∆s between two points: ∆s 2 = ∆x 2 + ∆y 2 .
Much of the important information in geometry is not the distance between two
points per se, but the closely related concept of length. For example, one of
the properties of flat space is that the length of the circumference of a circle is
equal to 2π times the length of its radius. Now, in flat space, distance is most
directly related to length for straight lines: the distance between two points is
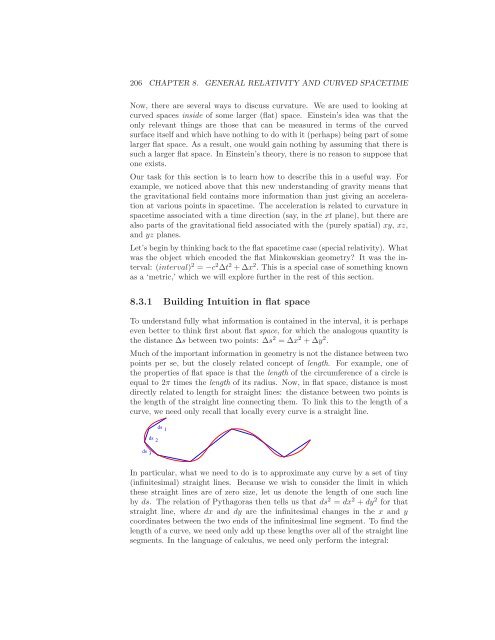
the length of the straight line connecting them. To link this to the length of a
curve, we need only recall that locally every curve is a straight line.
ds 3
ds
2
ds
1
In particular, what we need to do is to approximate any curve by a set of tiny
(infinitesimal) straight lines. Because we wish to consider the limit in which
these straight lines are of zero size, let us denote the length of one such line
by ds. The relation of Pythagoras then tells us that ds 2 = dx 2 + dy 2 for that
straight line, where dx and dy are the infinitesimal changes in the x and y
coordinates between the two ends of the infinitesimal line segment. To find the
length of a curve, we need only add up these lengths over all of the straight line
segments. In the language of calculus, we need only perform the integral: