Notes on Relativity and Cosmology - Physics Department, UCSB

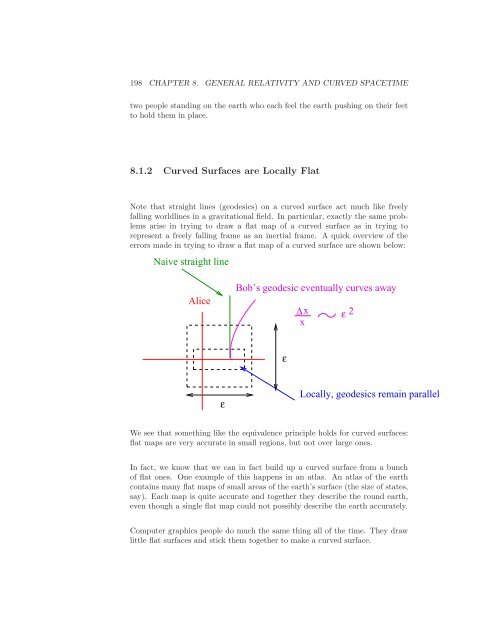
198 CHAPTER 8. GENERAL RELATIVITY AND CURVED SPACETIME
two people standing on the earth who each feel the earth pushing on their feet
to hold them in place.
8.1.2 Curved Surfaces are Locally Flat
Note that straight lines (geodesics) on a curved surface act much like freely
falling worldlines in a gravitational field. In particular, exactly the same problems
arise in trying to draw a flat map of a curved surface as in trying to
represent a freely falling frame as an inertial frame. A quick overview of the
errors made in trying to draw a flat map of a curved surface are shown below:
Naive straight line
Alice
Bob’s geodesic eventually curves away
∆x
x
ε 2
ε
ε
Locally, geodesics remain parallel
We see that something like the equivalence principle holds for curved surfaces:
flat maps are very accurate in small regions, but not over large ones.
In fact, we know that we can in fact build up a curved surface from a bunch
of flat ones. One example of this happens in an atlas. An atlas of the earth
contains many flat maps of small areas of the earth’s surface (the size of states,
say). Each map is quite accurate and together they describe the round earth,
even though a single flat map could not possibly describe the earth accurately.
Computer graphics people do much the same thing all of the time. They draw
little flat surfaces and stick them together to make a curved surface.