CASINO manual - Theory of Condensed Matter

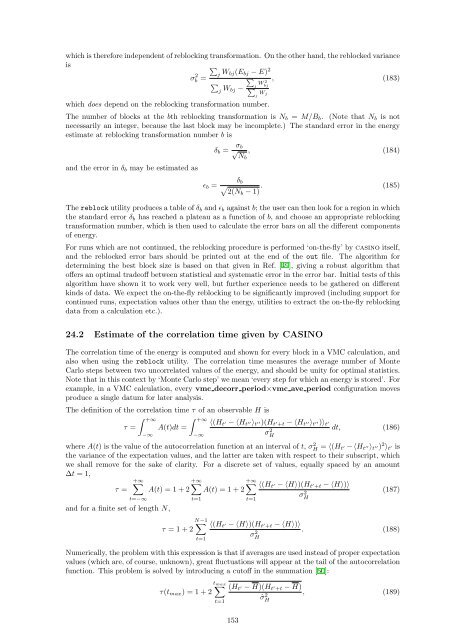
which is therefore independent of reblocking transformation. On the other hand, the reblocked variance
is
∑j
σb 2 =
W bj(E bj − E) 2
∑ , (183)
∑
j W bj − ∑ W 2 j bj
j Wj
which does depend on the reblocking transformation number.
The number of blocks at the bth reblocking transformation is N b = M/B b . (Note that N b is not
necessarily an integer, because the last block may be incomplete.) The standard error in the energy
estimate at reblocking transformation number b is
δ b =
σ b
√ , (184)
Nb
and the error in δ b may be estimated as
ɛ b =
δ b
√
2(Nb − 1) . (185)
The reblock utility produces a table of δ b and ɛ b against b; the user can then look for a region in which
the standard error δ b has reached a plateau as a function of b, and choose an appropriate reblocking
transformation number, which is then used to calculate the error bars on all the different components
of energy.
For runs which are not continued, the reblocking procedure is performed ‘on-the-fly’ by casino itself,
and the reblocked error bars should be printed out at the end of the out file. The algorithm for
determining the best block size is based on that given in Ref. [49], giving a robust algorithm that
offers an optimal tradeoff between statistical and systematic error in the error bar. Initial tests of this
algorithm have shown it to work very well, but further experience needs to be gathered on different
kinds of data. We expect the on-the-fly reblocking to be significantly improved (including support for
continued runs, expectation values other than the energy, utilities to extract the on-the-fly reblocking
data from a calculation etc.).
24.2 Estimate of the correlation time given by CASINO
The correlation time of the energy is computed and shown for every block in a VMC calculation, and
also when using the reblock utility. The correlation time measures the average number of Monte
Carlo steps between two uncorrelated values of the energy, and should be unity for optimal statistics.
Note that in this context by ‘Monte Carlo step’ we mean ‘every step for which an energy is stored’. For
example, in a VMC calculation, every vmc decorr period×vmc ave period configuration moves
produce a single datum for later analysis.
The definition of the correlation time τ of an observable H is
τ =
∫ +∞
t=−∞
−∞
A(t)dt =
∫ +∞
−∞
〈(H t ′ − 〈H t ′′〉 t ′′)(H t ′ +t − 〈H t ′′〉 t ′′)〉 t ′
σ 2 H
dt, (186)
where A(t) is the value of the autocorrelation function at an interval of t, σH 2 = 〈(H t ′ − 〈H t ′′〉 t ′′)2 〉 t ′ is
the variance of the expectation values, and the latter are taken with respect to their subscript, which
we shall remove for the sake of clarity. For a discrete set of values, equally spaced by an amount
∆t = 1,
+∞∑
+∞∑
+∞∑ 〈(H t ′ − 〈H〉)(H t
τ = A(t) = 1 + 2 A(t) = 1 + 2
′ +t − 〈H〉)〉
σH
2 (187)
and for a finite set of length N,
t=1
N−1
∑
τ = 1 + 2
t=1
t=1
〈(H t ′ − 〈H〉)(H t′ +t − 〈H〉)〉
σH
2 . (188)
Numerically, the problem with this expression is that if averages are used instead of proper expectation
values (which are, of course, unknown), great fluctuations will appear at the tail of the autocorrelation
function. This problem is solved by introducing a cutoff in the summation [50]:
t∑
max
τ(t max ) = 1 + 2
t=1
(H t ′ − H)(H t′ +t − H)
ˆσ
H
2 , (189)
153