CASINO manual - Theory of Condensed Matter

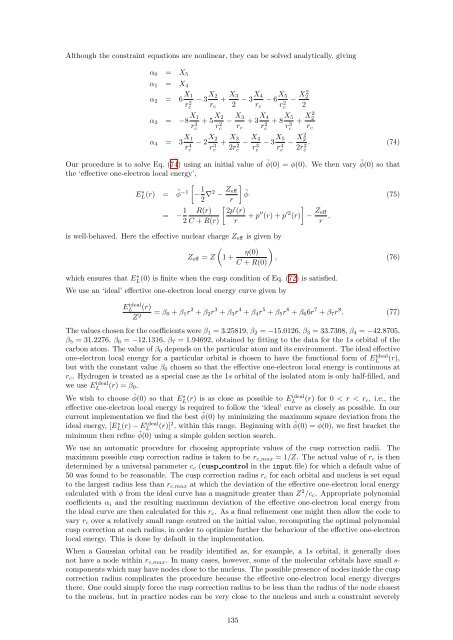
Although the constraint equations are nonlinear, they can be solved analytically, giving
α 0 = X 5
α 1 = X 4
α 2 = 6 X 1
r 2 c
α 3 = −8 X 1
r 3 c
α 4 = 3 X 1
r 4 c
− 3 X 2
+ X 3
r c 2 − 3X 4
− 6 X 5
r c rc
2 − X2 2
2
+ 5 X 2
r 2 c
− 2 X 2
r 3 c
− X 3
+ 3 X 4
r c rc
2 + 8 X 5
rc
3 + X2 2
r c
+ X 3
2r 2 c
− X 4
r 3 c
− 3 X 5
r 4 c
− X2 2
2rc
2 . (74)
Our procedure is to solve Eq. (74) using an initial value of ˜φ(0) = φ(0). We then vary ˜φ(0) so that
the ‘effective one-electron local energy’,
[
EL(r) s = ˜φ −1 − 1 2 ∇2 − Z ]
eff ˜φ (75)
r
= − 1 [
R(r) 2p ′ ]
(r)
+ p ′′ (r) + p ′2 (r) − Z eff
2 C + R(r) r
r ,
is well-behaved. Here the effective nuclear charge Z eff is given by
(
Z eff = Z 1 + η(0) )
, (76)
C + R(0)
which ensures that EL s (0) is finite when the cusp condition of Eq. (72) is satisfied.
We use an ‘ideal’ effective one-electron local energy curve given by
EL
ideal (r)
Z 2 = β 0 + β 1 r 2 + β 2 r 3 + β 3 r 4 + β 4 r 5 + β 5 r 6 + β 6 6r 7 + β 7 r 8 . (77)
The values chosen for the coefficients were β 1 = 3.25819, β 2 = −15.0126, β 3 = 33.7308, β 4 = −42.8705,
β 5 = 31.2276, β 6 = −12.1316, β 7 = 1.94692, obtained by fitting to the data for the 1s orbital of the
carbon atom. The value of β 0 depends on the particular atom and its environment. The ideal effective
one-electron local energy for a particular orbital is chosen to have the functional form of EL
ideal (r),
but with the constant value β 0 chosen so that the effective one-electron local energy is continuous at
r c . Hydrogen is treated as a special case as the 1s orbital of the isolated atom is only half-filled, and
we use EL ideal (r) = β 0 .
We wish to choose ˜φ(0) so that EL s (r) is as close as possible to Eideal L (r) for 0 < r < r c , i.e., the
effective one-electron local energy is required to follow the ‘ideal’ curve as closely as possible. In our
current implementation we find the best ˜φ(0) by minimizing the maximum square deviation from the
ideal energy, [EL s (r) − Eideal L (r)] 2 , within this range. Beginning with ˜φ(0) = φ(0), we first bracket the
minimum then refine ˜φ(0) using a simple golden section search.
We use an automatic procedure for choosing appropriate values of the cusp correction radii. The
maximum possible cusp correction radius is taken to be r c,max = 1/Z. The actual value of r c is then
determined by a universal parameter c c (cusp control in the input file) for which a default value of
50 was found to be reasonable. The cusp correction radius r c for each orbital and nucleus is set equal
to the largest radius less than r c,max at which the deviation of the effective one-electron local energy
calculated with φ from the ideal curve has a magnitude greater than Z 2 /c c . Appropriate polynomial
coefficients α i and the resulting maximum deviation of the effective one-electron local energy from
the ideal curve are then calculated for this r c . As a final refinement one might then allow the code to
vary r c over a relatively small range centred on the initial value, recomputing the optimal polynomial
cusp correction at each radius, in order to optimize further the behaviour of the effective one-electron
local energy. This is done by default in the implementation.
When a Gaussian orbital can be readily identified as, for example, a 1s orbital, it generally does
not have a node within r c,max . In many cases, however, some of the molecular orbitals have small s-
components which may have nodes close to the nucleus. The possible presence of nodes inside the cusp
correction radius complicates the procedure because the effective one-electron local energy diverges
there. One could simply force the cusp correction radius to be less than the radius of the node closest
to the nucleus, but in practice nodes can be very close to the nucleus and such a constraint severely
135