On the Analysis of Optical Mapping Data - University of Wisconsin ...

78
−2 0 2
Observed Quantiles
4
2
0
−2
−4
Raw errors
Average errors
−2 0 2
Quantiles of Standard Normal
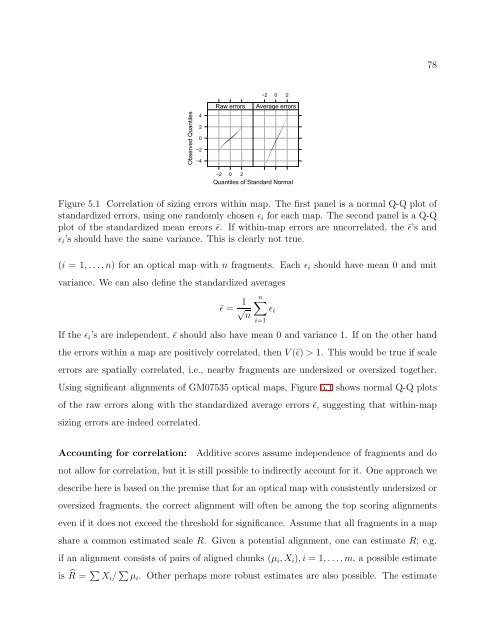
Figure 5.1 Correlation of sizing errors within map. The first panel is a normal Q-Q plot of
standardized errors, using one randomly chosen ǫ i for each map. The second panel is a Q-Q
plot of the standardized mean errors ¯ǫ. If within-map errors are uncorrelated, the ¯ǫ’s and
ǫ i ’s should have the same variance. This is clearly not true.
(i = 1, . . .,n) for an optical map with n fragments. Each ǫ i should have mean 0 and unit
variance. We can also define the standardized averages
¯ǫ = √ 1 n∑
n
If the ǫ i ’s are independent, ¯ǫ should also have mean 0 and variance 1. If on the other hand
the errors within a map are positively correlated, then V (¯ǫ) > 1. This would be true if scale
errors are spatially correlated, i.e., nearby fragments are undersized or oversized together.
Using significant alignments of GM07535 optical maps, Figure 5.1 shows normal Q-Q plots
of the raw errors along with the standardized average errors ¯ǫ, suggesting that within-map
sizing errors are indeed correlated.
i=1
ǫ i
Accounting for correlation: Additive scores assume independence of fragments and do
not allow for correlation, but it is still possible to indirectly account for it. One approach we
describe here is based on the premise that for an optical map with consistently undersized or
oversized fragments, the correct alignment will often be among the top scoring alignments
even if it does not exceed the threshold for significance. Assume that all fragments in a map
share a common estimated scale R. Given a potential alignment, one can estimate R; e.g.
if an alignment consists of pairs of aligned chunks (µ i , X i ), i = 1, . . ., m, a possible estimate
is ̂R = ∑ X i / ∑ µ i . Other perhaps more robust estimates are also possible. The estimate