Complex numbers 3

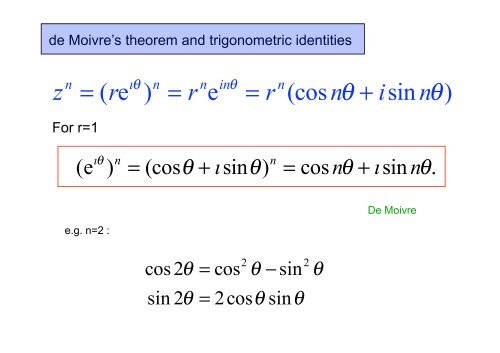
de Moivre’s theorem and trigonometric identities
z n = (re ıθ ) n = r n e inθ = r n (cos nθ + isin nθ)
For r=1
(e ıθ ) n = (cosθ + ısinθ) n = cos nθ + ısin nθ.
De Moivre
e.g. n=2 :
cos 2 cos sin
2 2
θ = θ − θ
sin 2θ = 2cosθ sinθ

Uses of de Moivre and complex exponentials
Ex. 1 Find (1+ ı) 8
. Taking powers is much simpler in polar form so we write
. Hence
ıπ /4
(1+ ı) = (2) e
.
(1+ ı) 8 = ( (2) e ıπ /4 ) 8 = 16e 2πı = 16

Uses of de Moivre and complex exponentials
Ex. 2
Solving differential equations
The solution to d 2 z
d 2
θ
z = Ce iθ
z = x + i y
+ z = 0 is simply given by
where C = A + ıB is a complex constant.
y = Im(z) = Im(( A + ıB)(cosθ + ısinθ))
= Asinθ + Bcosθ
c.f.
d 2 y
d 2
θ
+ y ≡ Im( d 2 z
+ z) = 0, v = Acosθ
+ Bsinθ
dθ 2

Uses of de Moivre and complex exponentials
Ex. 3 Summing series
e.g. Show
∞
∑
n=
0
r
n
(1 r)sin
sin(2n
+ θ
1) θ = +
,
2
1 − 2r
cos 2θ
+ r
0 < r < 1
∞
∑ r n sin(2n + 1)θ = Im∑ r n (e ı(2n+1)θ ) = Im(e ıθ
∑ (re 2ıθ ) n )
n=0
n
= Im(e ıθ 1
1− re 2ıθ )
e ıθ (1− re −2ıθ )
= Im(
(1− re 2ıθ )(1− re −2ıθ ) )
sinθ + r sinθ
=
1− 2r cos2θ + r 2
n

Curves in the complex plane
Ex 1
| z |=
1
iθ
z re r
= ⇒ =
1, any
θ
or
(a) 2 + (b) 2 = 1,
z = a + ib
Circle centre (0,0), radius 1
| z − z |= 1 0
Circle centre z 0 , radius 1
( a − a ) + ( b − b ) = 1
2 2
0 0

Curves in the complex plane
Ex 2
| z −ı
z +ı |= 1
| z − i | = | z + i |
Distance from (0,1) = distance from (0,-1)
Real axis
or (a) + ( b − 1) = (a) + ( b + 1)
2 2 2 2
z = a + ib
b
=
0, a arbitrary

Curves in the complex plane
Ex 3
arg( )
z π
z+ 1
=
4
i.e. arg(z) − arg(z + 1) = π 4
Take tan of both sides :
tan( A − B) =
tan A − tan B
1+ tan Atan B
b
a
−
b
1 + .
a
b
a+
1
b
a+
1
b( a + 1) − ba
= 1 =
2
a( a + 1) + b
b = a( a + 1) + b
2
z = a + ib
( a + ) + ( b − ) =
1 2 1 2 1
2 2 2
…but BEWARE…not all of circle satisfies equation…

arg( )
z π
z+ 1
=
4
z
z + 1 =
z
z + 1 . z * +1
z * + 1 = z(z* + 1)
z + 1 2
=
(a + ib)(a + 1− ib)
z + 1 2
= a(a + 1) + b2 + ib
z + 1 2
⇒
⎛
b > 0 since arg
⎝
⎜
z ⎞
z + 1⎠
⎟ positive
(a + 1 2 )2 + (b − 1 2 )2 = 1 2 , b > 0
( b < 0 solution introduced by tangent ambiguity)

Alternative solution
arg( )
z π
z 1 4
+
= i.e. arg(z) − arg(z + 1) = π 4
Solution : portion of circle through (0,0) and (-1,0)
Circle centre (-1/2,1/2) and radius 1/ 2

The lower portion of the circle is given by :
arg(
+
) = −
z 3π
z 1 4