A simple rule for the evolution of cooperation on graphs and social ...
A simple rule for the evolution of cooperation on graphs and social ...
A simple rule for the evolution of cooperation on graphs and social ...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
Vol 441|25 May 2006|doi:10.1038/nature04605<br />
LETTERS<br />
A <str<strong>on</strong>g>simple</str<strong>on</strong>g> <str<strong>on</strong>g>rule</str<strong>on</strong>g> <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g> <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g><br />
<strong>on</strong> <strong>graphs</strong> <strong>and</strong> <strong>social</strong> networks<br />
Hisashi Ohtsuki 1,2 , Christoph Hauert 2 , Erez Lieberman 2,3 & Martin A. Nowak 2<br />
A fundamental aspect <str<strong>on</strong>g>of</str<strong>on</strong>g> all biological systems is <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g>.<br />
Cooperative interacti<strong>on</strong>s are required <str<strong>on</strong>g>for</str<strong>on</strong>g> many levels <str<strong>on</strong>g>of</str<strong>on</strong>g> biological<br />
organizati<strong>on</strong> ranging from single cells to groups <str<strong>on</strong>g>of</str<strong>on</strong>g> animals 1–4 .<br />
Human society is based to a large extent <strong>on</strong> mechanisms that<br />
promote <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> 5–7 . It is well known that in unstructured<br />
populati<strong>on</strong>s, natural selecti<strong>on</strong> favours defectors over cooperators.<br />
There is much current interest, however, in studying <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary<br />
games in structured populati<strong>on</strong>s <strong>and</strong> <strong>on</strong> <strong>graphs</strong> 8–17 . These ef<str<strong>on</strong>g>for</str<strong>on</strong>g>ts<br />
recognize <str<strong>on</strong>g>the</str<strong>on</strong>g> fact that who-meets-whom is not r<strong>and</strong>om, but<br />
determined by spatial relati<strong>on</strong>ships or <strong>social</strong> networks 18–24 . Here<br />
we describe a surprisingly <str<strong>on</strong>g>simple</str<strong>on</strong>g> <str<strong>on</strong>g>rule</str<strong>on</strong>g> that is a good approximati<strong>on</strong><br />
<str<strong>on</strong>g>for</str<strong>on</strong>g> all <strong>graphs</strong> that we have analysed, including cycles,<br />
spatial lattices, r<strong>and</strong>om regular <strong>graphs</strong>, r<strong>and</strong>om <strong>graphs</strong> <strong>and</strong> scalefree<br />
networks 25,26 : natural selecti<strong>on</strong> favours <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g>, if <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
benefit <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> altruistic act, b, divided by <str<strong>on</strong>g>the</str<strong>on</strong>g> cost, c, exceeds <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
average number <str<strong>on</strong>g>of</str<strong>on</strong>g> neighbours, k, which means b/c > k. In this<br />
case, <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> can evolve as a c<strong>on</strong>sequence <str<strong>on</strong>g>of</str<strong>on</strong>g> ‘<strong>social</strong> viscosity’<br />
even in <str<strong>on</strong>g>the</str<strong>on</strong>g> absence <str<strong>on</strong>g>of</str<strong>on</strong>g> reputati<strong>on</strong> effects or strategic complexity.<br />
A cooperator is some<strong>on</strong>e who pays a cost, c, <str<strong>on</strong>g>for</str<strong>on</strong>g> ano<str<strong>on</strong>g>the</str<strong>on</strong>g>r individual<br />
to receive a benefit, b. A defector pays no cost <strong>and</strong> does not distribute<br />
any benefits. In <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary biology, cost <strong>and</strong> benefit are measured<br />
in terms <str<strong>on</strong>g>of</str<strong>on</strong>g> fitness. Reproducti<strong>on</strong> can be genetic or cultural. In <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
latter case, <str<strong>on</strong>g>the</str<strong>on</strong>g> strategy <str<strong>on</strong>g>of</str<strong>on</strong>g> some<strong>on</strong>e who does well is imitated by<br />
o<str<strong>on</strong>g>the</str<strong>on</strong>g>rs. In an unstructured populati<strong>on</strong>, where all individuals are<br />
equally likely to interact with each o<str<strong>on</strong>g>the</str<strong>on</strong>g>r, defectors have a higher<br />
average pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f than unc<strong>on</strong>diti<strong>on</strong>al cooperators. There<str<strong>on</strong>g>for</str<strong>on</strong>g>e, natural<br />
selecti<strong>on</strong> increases <str<strong>on</strong>g>the</str<strong>on</strong>g> relative abundance <str<strong>on</strong>g>of</str<strong>on</strong>g> defectors <strong>and</strong> drives<br />
cooperators to extincti<strong>on</strong>. These <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary dynamics hold <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
deterministic setting <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> replicator equati<strong>on</strong> 27,28 <strong>and</strong> <str<strong>on</strong>g>for</str<strong>on</strong>g> stochastic<br />
game dynamics <str<strong>on</strong>g>of</str<strong>on</strong>g> finite populati<strong>on</strong>s 29 .<br />
In our model, <str<strong>on</strong>g>the</str<strong>on</strong>g> players <str<strong>on</strong>g>of</str<strong>on</strong>g> an <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary game occupy <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
vertices <str<strong>on</strong>g>of</str<strong>on</strong>g> a graph. The edges denote links between individuals in<br />
terms <str<strong>on</strong>g>of</str<strong>on</strong>g> game dynamical interacti<strong>on</strong> <strong>and</strong> biological reproducti<strong>on</strong>.<br />
We assume that <str<strong>on</strong>g>the</str<strong>on</strong>g> graph is fixed <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> durati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary<br />
dynamics. C<strong>on</strong>sider a populati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> N individuals c<strong>on</strong>sisting <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
cooperators <strong>and</strong> defectors. A cooperator helps all individuals to<br />
whom it is c<strong>on</strong>nected. If a cooperator is c<strong>on</strong>nected to k o<str<strong>on</strong>g>the</str<strong>on</strong>g>r<br />
individuals <strong>and</strong> i <str<strong>on</strong>g>of</str<strong>on</strong>g> those are cooperators, <str<strong>on</strong>g>the</str<strong>on</strong>g>n its pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f is<br />
bi 2 ck. A defector does not provide any help, <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g>re<str<strong>on</strong>g>for</str<strong>on</strong>g>e has no<br />
costs, but it can receive <str<strong>on</strong>g>the</str<strong>on</strong>g> benefit from neighbouring cooperators. If<br />
a defector is c<strong>on</strong>nected to j cooperators, <str<strong>on</strong>g>the</str<strong>on</strong>g>n its pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f is bj.<br />
The fitness <str<strong>on</strong>g>of</str<strong>on</strong>g> an individual is given by a c<strong>on</strong>stant term, denoting<br />
<str<strong>on</strong>g>the</str<strong>on</strong>g> baseline fitness, plus <str<strong>on</strong>g>the</str<strong>on</strong>g> pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f that arises from <str<strong>on</strong>g>the</str<strong>on</strong>g> game. Str<strong>on</strong>g<br />
selecti<strong>on</strong> means that <str<strong>on</strong>g>the</str<strong>on</strong>g> pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f is large compared to <str<strong>on</strong>g>the</str<strong>on</strong>g> baseline<br />
fitness; weak selecti<strong>on</strong> means <str<strong>on</strong>g>the</str<strong>on</strong>g> pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f is small compared to <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
baseline fitness. The idea behind weak selecti<strong>on</strong> is that many different<br />
factors c<strong>on</strong>tribute to <str<strong>on</strong>g>the</str<strong>on</strong>g> overall fitness <str<strong>on</strong>g>of</str<strong>on</strong>g> an individual, <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g> game<br />
under c<strong>on</strong>siderati<strong>on</strong> is just <strong>on</strong>e <str<strong>on</strong>g>of</str<strong>on</strong>g> those factors.<br />
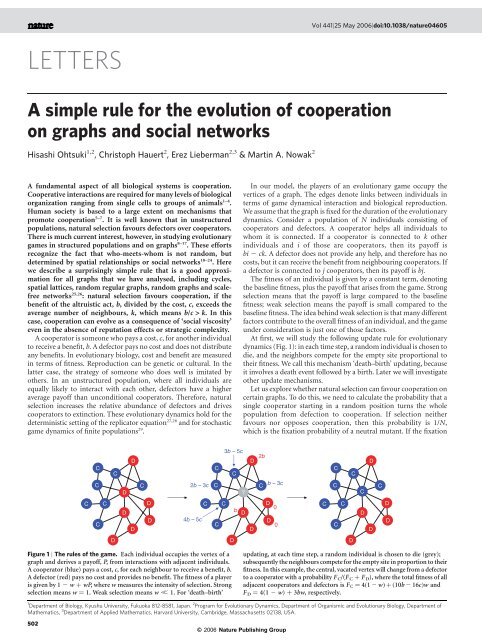
At first, we will study <str<strong>on</strong>g>the</str<strong>on</strong>g> following update <str<strong>on</strong>g>rule</str<strong>on</strong>g> <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary<br />
dynamics (Fig. 1): in each time step, a r<strong>and</strong>om individual is chosen to<br />
die, <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g> neighbors compete <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> empty site proporti<strong>on</strong>al to<br />
<str<strong>on</strong>g>the</str<strong>on</strong>g>ir fitness. We call this mechanism ‘death–birth’ updating, because<br />
it involves a death event followed by a birth. Later we will investigate<br />
o<str<strong>on</strong>g>the</str<strong>on</strong>g>r update mechanisms.<br />
Let us explore whe<str<strong>on</strong>g>the</str<strong>on</strong>g>r natural selecti<strong>on</strong> can favour <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> <strong>on</strong><br />
certain <strong>graphs</strong>. To do this, we need to calculate <str<strong>on</strong>g>the</str<strong>on</strong>g> probability that a<br />
single cooperator starting in a r<strong>and</strong>om positi<strong>on</strong> turns <str<strong>on</strong>g>the</str<strong>on</strong>g> whole<br />
populati<strong>on</strong> from defecti<strong>on</strong> to <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g>. If selecti<strong>on</strong> nei<str<strong>on</strong>g>the</str<strong>on</strong>g>r<br />
favours nor opposes <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g>, <str<strong>on</strong>g>the</str<strong>on</strong>g>n this probability is 1/N,<br />
which is <str<strong>on</strong>g>the</str<strong>on</strong>g> fixati<strong>on</strong> probability <str<strong>on</strong>g>of</str<strong>on</strong>g> a neutral mutant. If <str<strong>on</strong>g>the</str<strong>on</strong>g> fixati<strong>on</strong><br />
Figure 1 | The <str<strong>on</strong>g>rule</str<strong>on</strong>g>s <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> game. Each individual occupies <str<strong>on</strong>g>the</str<strong>on</strong>g> vertex <str<strong>on</strong>g>of</str<strong>on</strong>g> a<br />
graph <strong>and</strong> derives a pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f, P, from interacti<strong>on</strong>s with adjacent individuals.<br />
A cooperator (blue) pays a cost, c, <str<strong>on</strong>g>for</str<strong>on</strong>g> each neighbour to receive a benefit, b.<br />
A defector (red) pays no cost <strong>and</strong> provides no benefit. The fitness <str<strong>on</strong>g>of</str<strong>on</strong>g> a player<br />
is given by 1 2 w þ wP, where w measures <str<strong>on</strong>g>the</str<strong>on</strong>g> intensity <str<strong>on</strong>g>of</str<strong>on</strong>g> selecti<strong>on</strong>. Str<strong>on</strong>g<br />
selecti<strong>on</strong> means w ¼ 1. Weak selecti<strong>on</strong> means w ,, 1. For ‘death–birth’<br />
updating, at each time step, a r<strong>and</strong>om individual is chosen to die (grey);<br />
subsequently <str<strong>on</strong>g>the</str<strong>on</strong>g> neighbours compete <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> empty site in proporti<strong>on</strong> to <str<strong>on</strong>g>the</str<strong>on</strong>g>ir<br />
fitness. In this example, <str<strong>on</strong>g>the</str<strong>on</strong>g> central, vacated vertex will change from a defector<br />
to a cooperator with a probability F C /(F C þ F D ), where <str<strong>on</strong>g>the</str<strong>on</strong>g> total fitness <str<strong>on</strong>g>of</str<strong>on</strong>g> all<br />
adjacent cooperators <strong>and</strong> defectors is F C ¼ 4ð1 2 wÞþð10b 2 16cÞw <strong>and</strong><br />
F D ¼ 4(1 2 w) þ 3bw, respectively.<br />
1 Department <str<strong>on</strong>g>of</str<strong>on</strong>g> Biology, Kyushu University, Fukuoka 812-8581, Japan. 2 Program <str<strong>on</strong>g>for</str<strong>on</strong>g> Evoluti<strong>on</strong>ary Dynamics, Department <str<strong>on</strong>g>of</str<strong>on</strong>g> Organismic <strong>and</strong> Evoluti<strong>on</strong>ary Biology, Department <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
Ma<str<strong>on</strong>g>the</str<strong>on</strong>g>matics, 3 Department <str<strong>on</strong>g>of</str<strong>on</strong>g> Applied Ma<str<strong>on</strong>g>the</str<strong>on</strong>g>matics, Harvard University, Cambridge, Massachusetts 02138, USA.<br />
502<br />
© 2006 Nature Publishing Group
NATURE|Vol 441|25 May 2006<br />
LETTERS<br />
Figure 2 | The <str<strong>on</strong>g>simple</str<strong>on</strong>g> <str<strong>on</strong>g>rule</str<strong>on</strong>g>, b/c > k, is in good agreement with numerical<br />
simulati<strong>on</strong>s. The parameter k denotes <str<strong>on</strong>g>the</str<strong>on</strong>g> degree <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> graph, which is<br />
given by <str<strong>on</strong>g>the</str<strong>on</strong>g> (average) number <str<strong>on</strong>g>of</str<strong>on</strong>g> neighbours per individual. The first row<br />
illustrates <str<strong>on</strong>g>the</str<strong>on</strong>g> type <str<strong>on</strong>g>of</str<strong>on</strong>g> graph <str<strong>on</strong>g>for</str<strong>on</strong>g> k ¼ 2(a) <strong>and</strong> k ¼ 4(b–e). The sec<strong>on</strong>d <strong>and</strong><br />
third rows show simulati<strong>on</strong> data <str<strong>on</strong>g>for</str<strong>on</strong>g> populati<strong>on</strong> sizes N ¼ 100 <strong>and</strong> N ¼ 500.<br />
The fixati<strong>on</strong> probability, r, <str<strong>on</strong>g>of</str<strong>on</strong>g> cooperators is determined by <str<strong>on</strong>g>the</str<strong>on</strong>g> fracti<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
runs where cooperators reached fixati<strong>on</strong> out <str<strong>on</strong>g>of</str<strong>on</strong>g> 10 6 runs under weak<br />
selecti<strong>on</strong>, w ¼ 0.01. Each type <str<strong>on</strong>g>of</str<strong>on</strong>g> graph is simulated <str<strong>on</strong>g>for</str<strong>on</strong>g> different (average)<br />
degrees ranging from k ¼ 2tok ¼ 10. The arrows mark b/c ¼ k. The dotted<br />
horiz<strong>on</strong>tal line indicates <str<strong>on</strong>g>the</str<strong>on</strong>g> fixati<strong>on</strong> probability 1/N <str<strong>on</strong>g>of</str<strong>on</strong>g> neutral <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>.<br />
The data suggest that b/c . k is necessary but not sufficient. The discrepancy<br />
is larger <str<strong>on</strong>g>for</str<strong>on</strong>g> n<strong>on</strong>-regular <strong>graphs</strong> (d, e) with high average degree (k ¼ 10).<br />
This is not surprising given that <str<strong>on</strong>g>the</str<strong>on</strong>g> derivati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> <str<strong>on</strong>g>rule</str<strong>on</strong>g> is <str<strong>on</strong>g>for</str<strong>on</strong>g> regular<br />
<strong>graphs</strong> <strong>and</strong> in <str<strong>on</strong>g>the</str<strong>on</strong>g> limit N . k. Note that <str<strong>on</strong>g>the</str<strong>on</strong>g> larger populati<strong>on</strong> size,<br />
N ¼ 500, gives better agreement. Interactive <strong>on</strong>line tutorials can be found at<br />
http://univie.ac.at/virtuallabs.<br />
probability <str<strong>on</strong>g>of</str<strong>on</strong>g> a single cooperator is greater than 1/N, <str<strong>on</strong>g>the</str<strong>on</strong>g>n selecti<strong>on</strong><br />
favours <str<strong>on</strong>g>the</str<strong>on</strong>g> emergence <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g>. We also calculate <str<strong>on</strong>g>the</str<strong>on</strong>g> fixati<strong>on</strong><br />
probability <str<strong>on</strong>g>of</str<strong>on</strong>g> a single defector in a populati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> cooperators, <strong>and</strong><br />
compare <str<strong>on</strong>g>the</str<strong>on</strong>g> two fixati<strong>on</strong> probabilities.<br />
The traditi<strong>on</strong>al well-mixed populati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary game <str<strong>on</strong>g>the</str<strong>on</strong>g>ory<br />
is represented by <str<strong>on</strong>g>the</str<strong>on</strong>g> complete graph, where all vertices are c<strong>on</strong>nected.<br />
In this special situati<strong>on</strong>, cooperators are always opposed by selecti<strong>on</strong>.<br />
This is <str<strong>on</strong>g>the</str<strong>on</strong>g> fundamental intuiti<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> classical <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary game<br />
<str<strong>on</strong>g>the</str<strong>on</strong>g>ory. But what happens <strong>on</strong> o<str<strong>on</strong>g>the</str<strong>on</strong>g>r <strong>graphs</strong>?<br />
Let us first c<strong>on</strong>sider a cycle. Each individual is linked to two<br />
neighbours. A single cooperator could be wiped out immediately or<br />
take over <strong>on</strong>e <str<strong>on</strong>g>of</str<strong>on</strong>g> its two neighbours. A cluster <str<strong>on</strong>g>of</str<strong>on</strong>g> two cooperators<br />
could exp<strong>and</strong> to three cooperators or revert to a single cooperator. In<br />
any case, <str<strong>on</strong>g>the</str<strong>on</strong>g> lineage starting from <strong>on</strong>e cooperator always <str<strong>on</strong>g>for</str<strong>on</strong>g>ms a<br />
single cluster <str<strong>on</strong>g>of</str<strong>on</strong>g> cooperators, which cannot fragment into pieces. This<br />
fact allows a straight<str<strong>on</strong>g>for</str<strong>on</strong>g>ward calculati<strong>on</strong>. We find that selecti<strong>on</strong><br />
favours <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> if b/c . 2. This result holds <str<strong>on</strong>g>for</str<strong>on</strong>g> weak selecti<strong>on</strong><br />
<strong>and</strong> large populati<strong>on</strong> size.<br />
Next, we study regular <strong>graphs</strong>, where each individual has exactly k<br />
neighbours. Such <strong>graphs</strong> include cycles, spatial lattices <strong>and</strong> r<strong>and</strong>om<br />
regular <strong>graphs</strong>. For all such <strong>graphs</strong>, a direct calculati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> fixati<strong>on</strong><br />
probability is impossible, because a single invader can lead to very<br />
complicated patterns: <str<strong>on</strong>g>the</str<strong>on</strong>g> emerging cluster usually breaks into many<br />
pieces, allowing a large number <str<strong>on</strong>g>of</str<strong>on</strong>g> c<strong>on</strong>ceivable geometric c<strong>on</strong>figurati<strong>on</strong>s.<br />
In general, <str<strong>on</strong>g>the</str<strong>on</strong>g> inherent complexity <str<strong>on</strong>g>of</str<strong>on</strong>g> games <strong>on</strong> <strong>graphs</strong> makes<br />
analytical investigati<strong>on</strong>s almost always impossible.<br />
© 2006 Nature Publishing Group<br />
Never<str<strong>on</strong>g>the</str<strong>on</strong>g>less, we can calculate <str<strong>on</strong>g>the</str<strong>on</strong>g> fixati<strong>on</strong> probability <str<strong>on</strong>g>of</str<strong>on</strong>g> a r<strong>and</strong>omly<br />
placed mutant <str<strong>on</strong>g>for</str<strong>on</strong>g> any two-pers<strong>on</strong>, two-strategy game <strong>on</strong> a<br />
regular graph by using pair approximati<strong>on</strong> <strong>and</strong> diffusi<strong>on</strong> approximati<strong>on</strong><br />
(see Supplementary In<str<strong>on</strong>g>for</str<strong>on</strong>g>mati<strong>on</strong>). In particular, we find<br />
that cooperators have a fixati<strong>on</strong> probability greater than 1/N <strong>and</strong><br />
defectors have a fixati<strong>on</strong> probability less than 1/N, if:<br />
b=c . k<br />
The ratio <str<strong>on</strong>g>of</str<strong>on</strong>g> benefit to cost <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> altruistic act has to exceed <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
degree, k, which is given by <str<strong>on</strong>g>the</str<strong>on</strong>g> number <str<strong>on</strong>g>of</str<strong>on</strong>g> neighbours per individual.<br />
This c<strong>on</strong>diti<strong>on</strong> is derived <str<strong>on</strong>g>for</str<strong>on</strong>g> weak selecti<strong>on</strong> <strong>and</strong> under <str<strong>on</strong>g>the</str<strong>on</strong>g> assumpti<strong>on</strong><br />
that <str<strong>on</strong>g>the</str<strong>on</strong>g> populati<strong>on</strong> size, N, is much larger than <str<strong>on</strong>g>the</str<strong>on</strong>g> degree, k.<br />
We find excellent agreement with numerical simulati<strong>on</strong>s (Fig. 2).<br />
For a given populati<strong>on</strong> size, b/c . k is a necessary c<strong>on</strong>diti<strong>on</strong> <str<strong>on</strong>g>for</str<strong>on</strong>g><br />
selecti<strong>on</strong> to favour cooperators. As <str<strong>on</strong>g>the</str<strong>on</strong>g> populati<strong>on</strong> size increases, <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
discrepancy between b/c . k <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g> numerical simulati<strong>on</strong>s becomes<br />
smaller. Moreover, we find that <str<strong>on</strong>g>the</str<strong>on</strong>g> <str<strong>on</strong>g>rule</str<strong>on</strong>g> also holds <str<strong>on</strong>g>for</str<strong>on</strong>g> r<strong>and</strong>om<br />
<strong>graphs</strong> 25 <strong>and</strong> scale-free networks 26,27 , where individuals differ in <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
number <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g>ir neighbours. Here k denotes <str<strong>on</strong>g>the</str<strong>on</strong>g> average degree <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
graph. Scale-free networks fit slightly less well than r<strong>and</strong>om <strong>graphs</strong>,<br />
presumably because <str<strong>on</strong>g>the</str<strong>on</strong>g>y have a larger variance <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> degree<br />
distributi<strong>on</strong>.<br />
The intuitive justificati<strong>on</strong> <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> b/c . k <str<strong>on</strong>g>rule</str<strong>on</strong>g> is illustrated in Fig. 3.<br />
C<strong>on</strong>sider <strong>on</strong>e cooperator <strong>and</strong> <strong>on</strong>e defector competing <str<strong>on</strong>g>for</str<strong>on</strong>g> an empty<br />
site. The pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> cooperator is P C ¼ bq CjC (k 2 1) 2 ck. The<br />
pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> defector is P D ¼ bq CjD (k 2 1). The c<strong>on</strong>diti<strong>on</strong>al<br />
503
LETTERS NATURE|Vol 441|25 May 2006<br />
Figure 3 | Some intuiti<strong>on</strong> <str<strong>on</strong>g>for</str<strong>on</strong>g> games <strong>on</strong> <strong>graphs</strong>. a, For death–birth updating,<br />
we must c<strong>on</strong>sider a cooperator <strong>and</strong> a defector competing <str<strong>on</strong>g>for</str<strong>on</strong>g> an empty site.<br />
The pair-approximati<strong>on</strong> calculati<strong>on</strong> shows that <str<strong>on</strong>g>for</str<strong>on</strong>g> weak selecti<strong>on</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
cooperator has <strong>on</strong>e more cooperator am<strong>on</strong>g its k 2 1 o<str<strong>on</strong>g>the</str<strong>on</strong>g>r neighbours than<br />
does <str<strong>on</strong>g>the</str<strong>on</strong>g> defector. Hence, <str<strong>on</strong>g>the</str<strong>on</strong>g> cooperator has a higher chance to win <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
empty site if b/c . k. b, For birth–death updating, we must c<strong>on</strong>sider a<br />
cooperator–defector pair competing <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> next reproducti<strong>on</strong> event. Again<br />
<str<strong>on</strong>g>the</str<strong>on</strong>g> cooperator has <strong>on</strong>e more cooperator am<strong>on</strong>g its k 2 1 o<str<strong>on</strong>g>the</str<strong>on</strong>g>r neighbours<br />
than <str<strong>on</strong>g>the</str<strong>on</strong>g> defector, but <str<strong>on</strong>g>the</str<strong>on</strong>g> focal cooperator is also a neighbour <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> defector.<br />
Hence, both competitors are linked to <str<strong>on</strong>g>the</str<strong>on</strong>g> same number <str<strong>on</strong>g>of</str<strong>on</strong>g> cooperators, <strong>and</strong><br />
<str<strong>on</strong>g>the</str<strong>on</strong>g>re<str<strong>on</strong>g>for</str<strong>on</strong>g>e <str<strong>on</strong>g>the</str<strong>on</strong>g> defector has a higher pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f. For birth–death updating,<br />
selecti<strong>on</strong> does not favour <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g>. c, On a cycle (k ¼ 2), <str<strong>on</strong>g>the</str<strong>on</strong>g> situati<strong>on</strong> is<br />
<str<strong>on</strong>g>simple</str<strong>on</strong>g>. A direct calculati<strong>on</strong>, <str<strong>on</strong>g>for</str<strong>on</strong>g> weak selecti<strong>on</strong> <strong>and</strong> large populati<strong>on</strong> size,<br />
leads to <str<strong>on</strong>g>the</str<strong>on</strong>g> following results. For birth–death updating, <str<strong>on</strong>g>the</str<strong>on</strong>g> boundary<br />
between a cluster <str<strong>on</strong>g>of</str<strong>on</strong>g> cooperators <strong>and</strong> defectors tends to move in favour <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
defectors. For death–birth updating, <str<strong>on</strong>g>the</str<strong>on</strong>g> cooperator cluster exp<strong>and</strong>s if<br />
b/c . 2. For imitati<strong>on</strong> updating, <str<strong>on</strong>g>the</str<strong>on</strong>g> cooperator cluster exp<strong>and</strong>s if b/c . 4.<br />
probability to find a cooperator next to a cooperator is q CjC <strong>and</strong><br />
to find a cooperator next to a defector is q CjD . The cooperator pays<br />
cost c <str<strong>on</strong>g>for</str<strong>on</strong>g> all <str<strong>on</strong>g>of</str<strong>on</strong>g> its k neighbours <strong>and</strong> receives benefit b from each<br />
cooperator am<strong>on</strong>g its k 2 1 neighbours, excluding <str<strong>on</strong>g>the</str<strong>on</strong>g> c<strong>on</strong>tested site.<br />
The defector pays no cost, but receives benefit b from each cooperator<br />
am<strong>on</strong>g its k 2 1 neighbours, also excluding <str<strong>on</strong>g>the</str<strong>on</strong>g> c<strong>on</strong>tested site. The<br />
pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f that comes from <str<strong>on</strong>g>the</str<strong>on</strong>g> c<strong>on</strong>tested site is excluded, because it<br />
c<strong>on</strong>tributes equally to <str<strong>on</strong>g>the</str<strong>on</strong>g> cooperator <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g> defector <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g>re<str<strong>on</strong>g>for</str<strong>on</strong>g>e<br />
cancels out. If P C . P D , <str<strong>on</strong>g>the</str<strong>on</strong>g>n selecti<strong>on</strong> favours <str<strong>on</strong>g>the</str<strong>on</strong>g> cooperator.<br />
Pair-approximati<strong>on</strong> shows that (k 2 1)(q CjC 2 q CjD ) ¼ 1 <str<strong>on</strong>g>for</str<strong>on</strong>g> weak<br />
selecti<strong>on</strong>. Thus, <str<strong>on</strong>g>the</str<strong>on</strong>g> cooperator has <strong>on</strong> average <strong>on</strong>e more cooperator<br />
neighbour than <str<strong>on</strong>g>the</str<strong>on</strong>g> defector. There<str<strong>on</strong>g>for</str<strong>on</strong>g>e, we obtain P C 2 P D ¼ b 2 ck,<br />
which leads to <str<strong>on</strong>g>the</str<strong>on</strong>g> b/c . k <str<strong>on</strong>g>rule</str<strong>on</strong>g>.<br />
We have also explored o<str<strong>on</strong>g>the</str<strong>on</strong>g>r update mechanisms. Suppose at each<br />
time step a r<strong>and</strong>om individual is chosen to update its strategy; it will<br />
stay with its own strategy or imitate <strong>on</strong>e <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> neighbours proporti<strong>on</strong>al<br />
to fitness. For this ‘imitati<strong>on</strong>’ updating, we find that<br />
cooperators are favoured if b/c . k þ 2. This result can be obtained<br />
with an exact calculati<strong>on</strong> <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> cycle <strong>and</strong> with pair approximati<strong>on</strong><br />
<str<strong>on</strong>g>for</str<strong>on</strong>g> regular <strong>graphs</strong>. Again, <str<strong>on</strong>g>the</str<strong>on</strong>g>re is good agreement with numerical<br />
simulati<strong>on</strong>s (Supplementary Fig. 4). Ma<str<strong>on</strong>g>the</str<strong>on</strong>g>matically, imitati<strong>on</strong><br />
updating can be obtained from our earlier death–birth updating by<br />
adding loops to every vertex. There<str<strong>on</strong>g>for</str<strong>on</strong>g>e, each individual is also its<br />
own neighbour. Let us define <str<strong>on</strong>g>the</str<strong>on</strong>g> c<strong>on</strong>nectivity, k, <str<strong>on</strong>g>of</str<strong>on</strong>g> a vertex as <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
total number <str<strong>on</strong>g>of</str<strong>on</strong>g> links c<strong>on</strong>nected to that vertex, noting that a loop is<br />
c<strong>on</strong>nected twice. Then <str<strong>on</strong>g>the</str<strong>on</strong>g> <str<strong>on</strong>g>simple</str<strong>on</strong>g> <str<strong>on</strong>g>rule</str<strong>on</strong>g> b/c . k holds both <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
imitati<strong>on</strong> <strong>and</strong> death–birth updating.<br />
There are also update <str<strong>on</strong>g>rule</str<strong>on</strong>g>s <str<strong>on</strong>g>for</str<strong>on</strong>g> which selecti<strong>on</strong> can never favour<br />
cooperators. For example, let us c<strong>on</strong>sider ‘birth–death’ updating: at<br />
each time step an individual is selected <str<strong>on</strong>g>for</str<strong>on</strong>g> reproducti<strong>on</strong> proporti<strong>on</strong>al<br />
to fitness, <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g> <str<strong>on</strong>g>of</str<strong>on</strong>g>fspring replaces a r<strong>and</strong>omly chosen<br />
neighbour. In this case, selecti<strong>on</strong> always favours defectors, because<br />
<strong>on</strong>ly <str<strong>on</strong>g>the</str<strong>on</strong>g> pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f <str<strong>on</strong>g>of</str<strong>on</strong>g> individuals right at <str<strong>on</strong>g>the</str<strong>on</strong>g> boundary between<br />
cooperators <strong>and</strong> defectors matters, <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g>re cooperators are always<br />
at a disadvantage (Fig. 3b). In <str<strong>on</strong>g>the</str<strong>on</strong>g> two o<str<strong>on</strong>g>the</str<strong>on</strong>g>r models, <str<strong>on</strong>g>the</str<strong>on</strong>g> pay<str<strong>on</strong>g>of</str<strong>on</strong>g>fs <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
individuals that are <strong>on</strong>e place removed from <str<strong>on</strong>g>the</str<strong>on</strong>g> boundary also play a<br />
role, which gives <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> a chance to survive.<br />
Using a different model, van Baalen <strong>and</strong> R<strong>and</strong> 11 have derived a<br />
c<strong>on</strong>diti<strong>on</strong> <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> initial invasi<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> cooperators. In <str<strong>on</strong>g>the</str<strong>on</strong>g>ir model, <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
vertices <str<strong>on</strong>g>of</str<strong>on</strong>g> a spatial lattice (or a graph) are ei<str<strong>on</strong>g>the</str<strong>on</strong>g>r empty or occupied<br />
by cooperators or defectors. There are birth, death <strong>and</strong> migrati<strong>on</strong><br />
events. Implicitly, <str<strong>on</strong>g>the</str<strong>on</strong>g>y have shown that without migrati<strong>on</strong> a few<br />
cooperators can successfully invade a populati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> defectors if<br />
b/c . k 2 /(k 2 1). The difference between this result <strong>and</strong> ours is<br />
not surprising. The invasi<strong>on</strong> c<strong>on</strong>diti<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> ref. 11 examines whe<str<strong>on</strong>g>the</str<strong>on</strong>g>r<br />
rare cooperators are able to increase in abundance, whereas our<br />
fixati<strong>on</strong> probability includes <str<strong>on</strong>g>the</str<strong>on</strong>g> whole <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary trajectory<br />
including <str<strong>on</strong>g>the</str<strong>on</strong>g> initial invasi<strong>on</strong> <strong>and</strong> propagati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> cooperators as<br />
well as <str<strong>on</strong>g>the</str<strong>on</strong>g> final extincti<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> defectors. For a comparis<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> invasi<strong>on</strong><br />
<strong>and</strong> fixati<strong>on</strong> criteria see Wild <strong>and</strong> Taylor 30 .<br />
504<br />
Thus, we have shown that <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary dynamics <strong>on</strong> <strong>graphs</strong> can<br />
favour <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> over defecti<strong>on</strong> if <str<strong>on</strong>g>the</str<strong>on</strong>g> benefit to cost ratio, b/c, <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
<str<strong>on</strong>g>the</str<strong>on</strong>g> altruistic act exceeds <str<strong>on</strong>g>the</str<strong>on</strong>g> average c<strong>on</strong>nectivity, k. The fewer<br />
c<strong>on</strong>necti<strong>on</strong>s <str<strong>on</strong>g>the</str<strong>on</strong>g>re are, <str<strong>on</strong>g>the</str<strong>on</strong>g> easier it is <str<strong>on</strong>g>for</str<strong>on</strong>g> natural selecti<strong>on</strong> to promote<br />
<str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g>. In our present analysis, all c<strong>on</strong>necti<strong>on</strong>s are equally<br />
str<strong>on</strong>g. A next step will be to explore <strong>graphs</strong> with weighted edges. In<br />
<strong>social</strong> networks, people might have a substantial number <str<strong>on</strong>g>of</str<strong>on</strong>g> c<strong>on</strong>necti<strong>on</strong>s,<br />
but <strong>on</strong>ly very few <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g>m are str<strong>on</strong>g. Hence, <str<strong>on</strong>g>the</str<strong>on</strong>g> ‘effective’<br />
average degree, k, <str<strong>on</strong>g>of</str<strong>on</strong>g> many relevant networks could be small, <str<strong>on</strong>g>the</str<strong>on</strong>g>reby<br />
making selecti<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> <strong>on</strong> <strong>graphs</strong> a powerful opti<strong>on</strong>.<br />
Our study is <str<strong>on</strong>g>the</str<strong>on</strong>g>oretically motivated, but has implicati<strong>on</strong>s <str<strong>on</strong>g>for</str<strong>on</strong>g><br />
empirical research. For example, <strong>on</strong>e can envisage an experiment<br />
where people are asked to play a n<strong>on</strong>-repeated Pris<strong>on</strong>er’s Dilemma<br />
within a given network. Certain network structures should promote<br />
cooperative behaviour more than o<str<strong>on</strong>g>the</str<strong>on</strong>g>rs. In particular, more<br />
<str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> should emerge if c<strong>on</strong>nectivity is low. Moreover, in<br />
certain animal species <str<strong>on</strong>g>the</str<strong>on</strong>g>re exist complicated <strong>social</strong> networks.<br />
Observati<strong>on</strong>al studies could reveal how network structure affects<br />
<str<strong>on</strong>g>the</str<strong>on</strong>g> level <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g>; higher c<strong>on</strong>nectivity should reduce<br />
<str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g>. In this paper, as a logical first step, we have studied<br />
<str<strong>on</strong>g>the</str<strong>on</strong>g> <str<strong>on</strong>g>simple</str<strong>on</strong>g>st possible interacti<strong>on</strong> between unc<strong>on</strong>diti<strong>on</strong>al cooperators<br />
<strong>and</strong> defectors, but in an extended approach, both in terms <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g>ory<br />
<strong>and</strong> experiment, it will be interesting to see which strategies <str<strong>on</strong>g>of</str<strong>on</strong>g> direct<br />
or indirect reciprocity evolve <strong>on</strong> particular networks.<br />
Finally, we note <str<strong>on</strong>g>the</str<strong>on</strong>g> beautiful similarity <str<strong>on</strong>g>of</str<strong>on</strong>g> our finding with<br />
Hamilt<strong>on</strong>’s <str<strong>on</strong>g>rule</str<strong>on</strong>g> 1 , which states that kin selecti<strong>on</strong> can favour<br />
<str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> provided b/c . 1/r, where r is <str<strong>on</strong>g>the</str<strong>on</strong>g> coefficient <str<strong>on</strong>g>of</str<strong>on</strong>g> genetic<br />
relatedness between individuals. The similarity makes sense. In our<br />
framework, <str<strong>on</strong>g>the</str<strong>on</strong>g> average degree <str<strong>on</strong>g>of</str<strong>on</strong>g> a graph is an inverse measure <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
<strong>social</strong> relatedness (or <strong>social</strong> viscosity). The fewer friends I have <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
more str<strong>on</strong>gly my fate is bound to <str<strong>on</strong>g>the</str<strong>on</strong>g>irs.<br />
© 2006 Nature Publishing Group<br />
Received 7 December 2005; accepted 26 January 2006.<br />
1. Hamilt<strong>on</strong>, W. D. The genetical <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g> <str<strong>on</strong>g>of</str<strong>on</strong>g> <strong>social</strong> behaviour. J. Theor. Biol. 7,<br />
1–-16 (1964).<br />
2. Trivers, R. The <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g> <str<strong>on</strong>g>of</str<strong>on</strong>g> reciprocal altruism. Q. Rev. Biol. 46, 35–-57<br />
(1971).<br />
3. Axelrod, R. & Hamilt<strong>on</strong>, W. D. The <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g> <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g>. Science 211,<br />
1390–-1396 (1981).<br />
4. Wils<strong>on</strong>, E. O. Sociobiology (Harvard Univ. Press, Cambridge, Massachusetts,<br />
1975).<br />
5. Wedekind, C. & Milinski, M. Cooperati<strong>on</strong> through image scoring in humans.<br />
Science 288, 850–-852 (2000).<br />
6. Fehr, E. & Fischbacher, U. The nature <str<strong>on</strong>g>of</str<strong>on</strong>g> human altruism. Nature 425, 785–-791<br />
(2003).<br />
7. Nowak, M. A. & Sigmund, K. Evoluti<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> indirect reciprocity. Nature 437,<br />
1291–-1298 (2005).<br />
8. Nowak, M. A. & May, R. M. Evoluti<strong>on</strong>ary games <strong>and</strong> spatial chaos. Nature 359,<br />
826–-829 (1992).<br />
9. Killingback, T. & Doebeli, M. Spatial <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary game <str<strong>on</strong>g>the</str<strong>on</strong>g>ory: Hawks <strong>and</strong><br />
Doves revisited. Proc. R. Soc. L<strong>on</strong>d. B 263, 1135–-1144 (1996).<br />
10. Nakamaru, M., Matsuda, H. & Iwasa, Y. The <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g> <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> in a<br />
lattice-structured populati<strong>on</strong>. J. Theor. Biol. 184, 65–-81 (1997).
NATURE|Vol 441|25 May 2006<br />
LETTERS<br />
11. van Baalen, M. & R<strong>and</strong>, D. A. The unit <str<strong>on</strong>g>of</str<strong>on</strong>g> selecti<strong>on</strong> in viscous populati<strong>on</strong>s <strong>and</strong><br />
<str<strong>on</strong>g>the</str<strong>on</strong>g> <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g> <str<strong>on</strong>g>of</str<strong>on</strong>g> altruism. J. Theor. Biol. 193, 631–-648 (1998).<br />
12. Mitteldorf, J. & Wils<strong>on</strong>, D. S. Populati<strong>on</strong> viscosity <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g> <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g> <str<strong>on</strong>g>of</str<strong>on</strong>g> altruism.<br />
J. Theor. Biol. 204, 481–-496 (2000).<br />
13. Hauert, C., De M<strong>on</strong>te, S., H<str<strong>on</strong>g>of</str<strong>on</strong>g>bauer, J. & Sigmund, K. Volunteering as red queen<br />
mechanism <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> in public goods games. Science 296, 1129–-1132<br />
(2002).<br />
14. Le Galliard, J., Ferriere, R. & Dieckman, U. The adaptive dynamics <str<strong>on</strong>g>of</str<strong>on</strong>g> altruism in<br />
spatially heterogeneous populati<strong>on</strong>s. Evoluti<strong>on</strong> 57, 1–-17 (2003).<br />
15. Hauert, C. & Doebeli, M. Spatial structure <str<strong>on</strong>g>of</str<strong>on</strong>g>ten inhibits <str<strong>on</strong>g>the</str<strong>on</strong>g> <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g> <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
<str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> in <str<strong>on</strong>g>the</str<strong>on</strong>g> snowdrift game. Nature 428, 643–-646 (2004).<br />
16. Ifti, M., Killingback, T. & Doebeli, M. Effects <str<strong>on</strong>g>of</str<strong>on</strong>g> neighbourhood size <strong>and</strong><br />
c<strong>on</strong>nectivity <strong>on</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g> spatial c<strong>on</strong>tinuous pris<strong>on</strong>er’s dilemma. J. Theor. Biol. 231,<br />
97–-106 (2004).<br />
17. Santos, F. C. & Pacheco, J. M. Scale-free networks provide a unifying<br />
framework <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> emergence <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g>. Phys. Rev. Lett. 95, 098104<br />
(2005).<br />
18. Levin, S. A. & Paine, R. T. Disturbance, patch <str<strong>on</strong>g>for</str<strong>on</strong>g>mati<strong>on</strong>, <strong>and</strong> community<br />
structure. Proc. Natl Acad. Sci. USA 71, 2744–-2747 (1974).<br />
19. Durrett, R. & Levin, S. A. The importance <str<strong>on</strong>g>of</str<strong>on</strong>g> being discrete (<strong>and</strong> spatial). Theor.<br />
Popul. Biol. 46, 363–-394 (1994).<br />
20. Hassell, M. P., Comins, H. N. & May, R. M. Species coexistence <strong>and</strong><br />
self-organizing spatial dynamics. Nature 370, 290–-292 (1994).<br />
21. Skyrms, B. & Pemantle, R. A dynamic model <str<strong>on</strong>g>of</str<strong>on</strong>g> <strong>social</strong> network <str<strong>on</strong>g>for</str<strong>on</strong>g>mati<strong>on</strong>. Proc.<br />
Natl Acad. Sci. USA 97, 9340–-9346 (2000).<br />
22. Abrams<strong>on</strong>, G. & Kuperman, M. Social games in a <strong>social</strong> network. Phys. Rev. E<br />
63, 030901 (2001).<br />
23. Szabó, G. & Vukov, J. Cooperati<strong>on</strong> <str<strong>on</strong>g>for</str<strong>on</strong>g> volunteering <strong>and</strong> partially r<strong>and</strong>om<br />
partnership. Phys. Rev. E 69, 036107 (2004).<br />
24. Lieberman, E., Hauert, C. & Nowak, M. A. Evoluti<strong>on</strong>ary dynamics <strong>on</strong> <strong>graphs</strong>.<br />
Nature 433, 312–-316 (2005).<br />
25. Watts, D. J. & Strogatz, S. H. Collective dynamics <str<strong>on</strong>g>of</str<strong>on</strong>g> ‘small-world’ networks.<br />
Nature 393, 440–-442 (1998).<br />
26. Barabasi, A. & Albert, R. Emergence <str<strong>on</strong>g>of</str<strong>on</strong>g> scaling in r<strong>and</strong>om networks. Science<br />
286, 509–-512 (1999).<br />
27. Taylor, P. D. & J<strong>on</strong>ker, L. Evoluti<strong>on</strong>ary stable strategies <strong>and</strong> game dynamics.<br />
Math. Biosci. 40, 145–-156 (1978).<br />
28. H<str<strong>on</strong>g>of</str<strong>on</strong>g>bauer, J. & Sigmund, K. Evoluti<strong>on</strong>ary Games <strong>and</strong> Populati<strong>on</strong> Dynamics<br />
(Cambridge Univ. Press, Cambridge, UK, 1998).<br />
29. Nowak, M. A., Sasaki, A., Taylor, C. & Fudenberg, D. Emergence <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
<str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> <strong>and</strong> <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary stability in finite populati<strong>on</strong>s. Nature 428,<br />
646–-650 (2004).<br />
30. Wild, G. & Taylor, P. D. Fitness <strong>and</strong> <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary stability in game<br />
<str<strong>on</strong>g>the</str<strong>on</strong>g>oretic models <str<strong>on</strong>g>of</str<strong>on</strong>g> finite populati<strong>on</strong>s. Proc. R. Soc. L<strong>on</strong>d. B 271, 2345–-2349<br />
(2004).<br />
Supplementary In<str<strong>on</strong>g>for</str<strong>on</strong>g>mati<strong>on</strong> is linked to <str<strong>on</strong>g>the</str<strong>on</strong>g> <strong>on</strong>line versi<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> paper at<br />
www.nature.com/nature.<br />
Acknowledgements Support from <str<strong>on</strong>g>the</str<strong>on</strong>g> John Templet<strong>on</strong> Foundati<strong>on</strong>, JSPS,<br />
NDSEG <strong>and</strong> Harvard-MIT HST is gratefully acknowledged. The Program <str<strong>on</strong>g>for</str<strong>on</strong>g><br />
Evoluti<strong>on</strong>ary Dynamics at Harvard University is sp<strong>on</strong>sored by Jeffrey Epstein.<br />
Author In<str<strong>on</strong>g>for</str<strong>on</strong>g>mati<strong>on</strong> Reprints <strong>and</strong> permissi<strong>on</strong>s in<str<strong>on</strong>g>for</str<strong>on</strong>g>mati<strong>on</strong> is available at<br />
npg.nature.com/reprints<strong>and</strong>permissi<strong>on</strong>s. The authors declare no competing<br />
financial interests. Corresp<strong>on</strong>dence <strong>and</strong> requests <str<strong>on</strong>g>for</str<strong>on</strong>g> materials should be<br />
addressed to M.A.N. (martin_nowak@harvard.edu).<br />
© 2006 Nature Publishing Group<br />
505
A <str<strong>on</strong>g>simple</str<strong>on</strong>g> <str<strong>on</strong>g>rule</str<strong>on</strong>g> <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g> <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> <strong>on</strong><br />
<strong>graphs</strong> <strong>and</strong> <strong>social</strong> networks<br />
Hisashi Ohtsuki, Christoph Hauert, Erez Lieberman, Martin A. Nowak<br />
February 10, 2006<br />
Supplementary In<str<strong>on</strong>g>for</str<strong>on</strong>g>mati<strong>on</strong><br />
C<strong>on</strong>sider a game between two strategies, A <strong>and</strong> B, with <str<strong>on</strong>g>the</str<strong>on</strong>g> general pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f matrix<br />
A B<br />
⎛ ⎞<br />
A a b<br />
⎝ ⎠. (1)<br />
B c d<br />
A populati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> fixed size N is distributed over <str<strong>on</strong>g>the</str<strong>on</strong>g> vertices <str<strong>on</strong>g>of</str<strong>on</strong>g> a graph. All vertices <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> graph are<br />
occupied by individuals who use ei<str<strong>on</strong>g>the</str<strong>on</strong>g>r strategy A or B. The pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f <str<strong>on</strong>g>of</str<strong>on</strong>g> each individual is <str<strong>on</strong>g>the</str<strong>on</strong>g> sum over<br />
all interacti<strong>on</strong>s with its neighbors. Each vertex is c<strong>on</strong>nected to k o<str<strong>on</strong>g>the</str<strong>on</strong>g>r vertices; this number denotes <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
degree <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> graph.<br />
We study three different update <str<strong>on</strong>g>rule</str<strong>on</strong>g>s. First, we c<strong>on</strong>sider ‘death-birth’ (DB) updating: in each time<br />
step a r<strong>and</strong>om individual is chosen to die; subsequently <str<strong>on</strong>g>the</str<strong>on</strong>g> neighbors compete <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> empty site proporti<strong>on</strong>al<br />
to <str<strong>on</strong>g>the</str<strong>on</strong>g>ir fitness. Sec<strong>on</strong>d, we c<strong>on</strong>sider imitati<strong>on</strong> (IM) updating: in each time step a r<strong>and</strong>om individual<br />
is chosen to evaluate its strategy; it will ei<str<strong>on</strong>g>the</str<strong>on</strong>g>r stay with its own strategy or imitate a neighbor’s strategy<br />
proporti<strong>on</strong>al to fitness. Third, we c<strong>on</strong>sider ‘birth-death’ (BD) updating: in each time step an individual<br />
is chosen <str<strong>on</strong>g>for</str<strong>on</strong>g> reproducti<strong>on</strong> proporti<strong>on</strong>al to fitness; <str<strong>on</strong>g>the</str<strong>on</strong>g> <str<strong>on</strong>g>of</str<strong>on</strong>g>fspring replaces a r<strong>and</strong>om neighbor. In an unstructured<br />
populati<strong>on</strong>, <str<strong>on</strong>g>the</str<strong>on</strong>g>se three update mechanism generate almost equivalent <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary dynamics,<br />
but <str<strong>on</strong>g>for</str<strong>on</strong>g> games <strong>on</strong> <strong>graphs</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g>y lead to very different outcomes.<br />
Using a combinati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> pair approximati<strong>on</strong> 1 <strong>and</strong> diffusi<strong>on</strong> approximati<strong>on</strong> 2 , we derive <str<strong>on</strong>g>the</str<strong>on</strong>g> fixati<strong>on</strong><br />
probability, ρ A , which represents <str<strong>on</strong>g>the</str<strong>on</strong>g> probability that a single A player starting in a r<strong>and</strong>om positi<strong>on</strong> <strong>on</strong><br />
<str<strong>on</strong>g>the</str<strong>on</strong>g> graph (with N − 1 many B players occupying <str<strong>on</strong>g>the</str<strong>on</strong>g> remaining positi<strong>on</strong>s) generates a lineage <str<strong>on</strong>g>of</str<strong>on</strong>g> A<br />
1
players that takes over <str<strong>on</strong>g>the</str<strong>on</strong>g> entire populati<strong>on</strong>. If ρ A > 1/N, <str<strong>on</strong>g>the</str<strong>on</strong>g>n selecti<strong>on</strong> favors <str<strong>on</strong>g>the</str<strong>on</strong>g> fixati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> A. We<br />
can also compare <str<strong>on</strong>g>the</str<strong>on</strong>g> two fixati<strong>on</strong> probabilities, ρ A <strong>and</strong> ρ B .<br />
Having derived <str<strong>on</strong>g>the</str<strong>on</strong>g> c<strong>on</strong>diti<strong>on</strong>s <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> general game, we will <str<strong>on</strong>g>the</str<strong>on</strong>g>n examine <str<strong>on</strong>g>the</str<strong>on</strong>g> fixati<strong>on</strong> probabilities,<br />
ρ C <strong>and</strong> ρ D , <str<strong>on</strong>g>for</str<strong>on</strong>g> cooperators, C, <strong>and</strong> defectors, D, in a Pris<strong>on</strong>er’s Dilemma given by <str<strong>on</strong>g>the</str<strong>on</strong>g> pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f matrix<br />
C D<br />
⎛ ⎞<br />
C b − c −c<br />
⎝ ⎠.<br />
D b 0<br />
The parameters b <strong>and</strong> c denote <str<strong>on</strong>g>the</str<strong>on</strong>g> benefit <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> recipient <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g> cost <str<strong>on</strong>g>for</str<strong>on</strong>g> d<strong>on</strong>or <str<strong>on</strong>g>of</str<strong>on</strong>g> an altruistic act.<br />
For DB updating, we find that ρ C > 1/N > ρ D if b/c > k. For IM updating, we find that<br />
ρ C > 1/N > ρ D if b/c > k + 2, where k is <str<strong>on</strong>g>the</str<strong>on</strong>g> degree <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> graph. For BD updating, we obtain that<br />
ρ D > 1/N > ρ C always holds, <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g>re<str<strong>on</strong>g>for</str<strong>on</strong>g>e selecti<strong>on</strong> cannot favor <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> in this case.<br />
These results hold <str<strong>on</strong>g>for</str<strong>on</strong>g> large populati<strong>on</strong> size, N >> k, <strong>and</strong> weak selecti<strong>on</strong>. Moreover, pair approximati<strong>on</strong><br />
is <str<strong>on</strong>g>for</str<strong>on</strong>g>mulated <str<strong>on</strong>g>for</str<strong>on</strong>g> Be<str<strong>on</strong>g>the</str<strong>on</strong>g> lattices (or Cayley trees), which are regular <strong>graphs</strong> without any loops.<br />
There<str<strong>on</strong>g>for</str<strong>on</strong>g>e, some discrepancy with numerical simulati<strong>on</strong>s <strong>on</strong> <strong>graphs</strong> with loops is expected.<br />
1 ‘Death-birth’ (DB) updating<br />
Let p A <strong>and</strong> p B denote <str<strong>on</strong>g>the</str<strong>on</strong>g> frequencies <str<strong>on</strong>g>of</str<strong>on</strong>g> A <strong>and</strong> B in <str<strong>on</strong>g>the</str<strong>on</strong>g> populati<strong>on</strong>. Let p AA , p AB , p BA <strong>and</strong> p BB denote<br />
<str<strong>on</strong>g>the</str<strong>on</strong>g> frequencies <str<strong>on</strong>g>of</str<strong>on</strong>g> AA, AB, BA <strong>and</strong> BB pairs. Pair approximati<strong>on</strong> means that <str<strong>on</strong>g>the</str<strong>on</strong>g> frequencies <str<strong>on</strong>g>of</str<strong>on</strong>g> larger<br />
clusters are derived from <str<strong>on</strong>g>the</str<strong>on</strong>g> frequencies <str<strong>on</strong>g>of</str<strong>on</strong>g> pair. Let q X|Y denote <str<strong>on</strong>g>the</str<strong>on</strong>g> c<strong>on</strong>diti<strong>on</strong>al probability to find an<br />
X-player given that <str<strong>on</strong>g>the</str<strong>on</strong>g> adjacent node is occupied by a Y -player. Here, both X <strong>and</strong> Y st<strong>and</strong> <str<strong>on</strong>g>for</str<strong>on</strong>g> A or B.<br />
The identities<br />
p A + p B = 1<br />
q A|X + q B|X = 1<br />
(2)<br />
p XY = q X|Y · p Y<br />
p AB = p BA<br />
imply that <str<strong>on</strong>g>the</str<strong>on</strong>g> whole system can be described by <strong>on</strong>ly two variables, p A <strong>and</strong> q A|A , in pair approximati<strong>on</strong>.<br />
Each player derives a pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f from interacti<strong>on</strong> with all its neighbors. At each time step, a r<strong>and</strong>om<br />
player is chosen to die. The neighbors compete <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> empty site proporti<strong>on</strong>al to <str<strong>on</strong>g>the</str<strong>on</strong>g>ir fitness. First we<br />
calculate <str<strong>on</strong>g>the</str<strong>on</strong>g> probabilities that <str<strong>on</strong>g>the</str<strong>on</strong>g> variables p A <strong>and</strong> p AA change during <strong>on</strong>e time step.<br />
2
1.1 Updating a B-player<br />
A B player is eliminated with probability p B . Its k neighbors compete <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> vacancy. Let k A <strong>and</strong> k B<br />
denote <str<strong>on</strong>g>the</str<strong>on</strong>g> numbers <str<strong>on</strong>g>of</str<strong>on</strong>g> A <strong>and</strong> B players am<strong>on</strong>g <str<strong>on</strong>g>the</str<strong>on</strong>g>se k neighbors. We have k A + k B = k. The frequency<br />
<str<strong>on</strong>g>of</str<strong>on</strong>g> such a c<strong>on</strong>figurati<strong>on</strong> is (k!/k A !k B !) q A|B<br />
k A<br />
q B|B<br />
k B<br />
. The fitness <str<strong>on</strong>g>of</str<strong>on</strong>g> each A-player is<br />
The fitness <str<strong>on</strong>g>of</str<strong>on</strong>g> each B-player is<br />
[<br />
]<br />
f A = (1 − w) + w (k − 1)q A|A · a + {(k − 1)q B|A + 1} · b . (3)<br />
[<br />
]<br />
f B = (1 − w) + w (k − 1)q A|B · c + {(k − 1)q B|B + 1} · d . (4)<br />
The parameter w represents <str<strong>on</strong>g>the</str<strong>on</strong>g> intensity <str<strong>on</strong>g>of</str<strong>on</strong>g> selecti<strong>on</strong>. If w = 1 <str<strong>on</strong>g>the</str<strong>on</strong>g>n <str<strong>on</strong>g>the</str<strong>on</strong>g> fitness is identical to pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f;<br />
this is <str<strong>on</strong>g>the</str<strong>on</strong>g> case <str<strong>on</strong>g>of</str<strong>on</strong>g> str<strong>on</strong>g selecti<strong>on</strong>. If w
The probability that <strong>on</strong>e <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> B-players replaces <str<strong>on</strong>g>the</str<strong>on</strong>g> vacancy is given by<br />
k B g B<br />
k A g A + k B g B<br />
.<br />
The vacancy is replaced by a B-player <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g>re<str<strong>on</strong>g>for</str<strong>on</strong>g>e p A decreases by 1/N with probability<br />
(<br />
Prob ∆p A = − 1 ) ∑<br />
= p A<br />
N<br />
k A +k B =k<br />
k!<br />
k A !k B ! q A|A k A k<br />
q B<br />
k B g B<br />
B|A . (9)<br />
k A g A + k B g B<br />
Regarding pairs, <str<strong>on</strong>g>the</str<strong>on</strong>g> number <str<strong>on</strong>g>of</str<strong>on</strong>g> AA-pairs decreases by k A <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g>re<str<strong>on</strong>g>for</str<strong>on</strong>g>e p AA decreases by k A /(kN/2)<br />
with probability<br />
(<br />
Prob ∆p AA = − 2k )<br />
A k!<br />
= p A<br />
kN k A !k B ! q A|A k A k<br />
q B<br />
k B g B<br />
B|A . (10)<br />
k A g A + k B g B<br />
1.3 Diffusi<strong>on</strong> approximati<strong>on</strong><br />
Let us now suppose that <strong>on</strong>e replacement event takes place in <strong>on</strong>e unit <str<strong>on</strong>g>of</str<strong>on</strong>g> time. The time derivatives <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
p A <strong>and</strong> p AA are given by<br />
<strong>and</strong><br />
ṗ AA =<br />
ṗ A = 1 N · Prob (<br />
∆p A = 1 N<br />
k∑<br />
k A =0<br />
We have used <str<strong>on</strong>g>the</str<strong>on</strong>g> notati<strong>on</strong><br />
From Eq.(12) we have<br />
) (<br />
+ − 1 ) (<br />
· Prob ∆p A = − 1 )<br />
N<br />
N<br />
= w · k − 1<br />
N p AB(I a a + I b b − I c c − I d d) + O(w 2 )<br />
2k<br />
(<br />
A<br />
kN · Prob<br />
∆p A = 2k A<br />
kN<br />
)<br />
+<br />
= 2 [<br />
]<br />
kN p AB 1 + (k − 1)(q A|B − q A|A )<br />
k∑ (<br />
k A =0<br />
− 2k A<br />
kN<br />
+ O(w).<br />
) (<br />
· Prob<br />
I a = k − 1<br />
k<br />
q A|A(q A|A + q B|B ) + 1 k q A|A,<br />
I b = k − 1<br />
k<br />
q B|A(q A|A + q B|B ) + 1 k q B|B,<br />
I c = k − 1<br />
k<br />
q A|B(q A|A + q B|B ) + 1 k q A|A,<br />
I d = k − 1<br />
k<br />
q B|B(q A|A + q B|B ) + 1 k q B|B.<br />
˙q A|A = d ( )<br />
pAA<br />
dt p A<br />
= 2 p<br />
[<br />
]<br />
AB<br />
1 + (k − 1)(q<br />
kN p A|B − q A|A ) + O(w).<br />
A<br />
∆p A = − 2k A<br />
kN<br />
)<br />
(11)<br />
(12)<br />
(13)<br />
(14)<br />
4
Remember that <str<strong>on</strong>g>the</str<strong>on</strong>g> system is described by p A <strong>and</strong> q A|A . Rewriting <str<strong>on</strong>g>the</str<strong>on</strong>g> r.h.s’s <str<strong>on</strong>g>of</str<strong>on</strong>g> Eq.(11) <strong>and</strong> Eq.(14)<br />
as functi<strong>on</strong>s <str<strong>on</strong>g>of</str<strong>on</strong>g> p A <strong>and</strong> q A|A yields <str<strong>on</strong>g>the</str<strong>on</strong>g> closed dynamical system:<br />
ṗ A = w · F 1 (p A , q A|A ) + O(w 2 ),<br />
˙q A|A = F 2 (p A , q A|A ) + O(w).<br />
(15)<br />
For weak selecti<strong>on</strong>, w k.<br />
Instead <str<strong>on</strong>g>of</str<strong>on</strong>g> studying a diffusi<strong>on</strong> process with respect to two r<strong>and</strong>om variables, p A <strong>and</strong> q A|A , we now<br />
assume, that <str<strong>on</strong>g>the</str<strong>on</strong>g> relati<strong>on</strong>ship given by Eq.(16) always holds. Hence, we study a <strong>on</strong>e dimensi<strong>on</strong>al diffusi<strong>on</strong><br />
process <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> r<strong>and</strong>om variable p A .<br />
Here<br />
Within <str<strong>on</strong>g>the</str<strong>on</strong>g> short time interval, ∆t, we have<br />
(<br />
)<br />
k − 2<br />
E[∆p A ] ≃ w ·<br />
k(k − 1)N p A(1 − p A )(αp A + β)∆t ≡ m(p A )∆t ,<br />
Var[∆p A ] ≃ 2<br />
( )<br />
k − 2<br />
N 2 k − 1 p A(1 − p A )∆t ≡ v(p A )∆t .<br />
(18)<br />
α = (k + 1)(k − 2)(a − b − c + d),<br />
β = (k + 1)a + (k 2 − k − 1)b − c − (k 2 − 1)d.<br />
(19)<br />
The fixati<strong>on</strong> probability, φ A (y) <str<strong>on</strong>g>of</str<strong>on</strong>g> strategy A with initial frequency p A (t = 0) = y, satisfies <str<strong>on</strong>g>the</str<strong>on</strong>g> following<br />
differential equati<strong>on</strong>:<br />
0 = m(y) dφ A(y)<br />
dy<br />
+ v(y) d 2 φ A (y)<br />
2 dy 2 . (20)<br />
5
Since w is very small, we have<br />
as an approximate soluti<strong>on</strong>.<br />
φ A (y) = y + w · N<br />
6k y(1 − y)[ (α + 3β) + αy ] (21)<br />
1.4 Fixati<strong>on</strong> probabilities<br />
The fixati<strong>on</strong> probability <str<strong>on</strong>g>of</str<strong>on</strong>g> a single A player in a populati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> N − 1 B players is given by ρ A =<br />
φ A (1/N). For large N, we have ρ A > 1/N if <strong>and</strong> <strong>on</strong>ly if α + 3β > 0, which is equivalent to<br />
(k 2 + 2k + 1)a + (2k 2 − 2k − 1)b > (k 2 − k + 1)c + (2k 2 + k − 1)d. (22)<br />
Similarly, ρ B > 1/N is equivalent to<br />
(k 2 + 2k + 1)d + (2k 2 − 2k − 1)c > (k 2 − k + 1)b + (2k 2 + k − 1)a. (23)<br />
For k = 2, both <str<strong>on</strong>g>the</str<strong>on</strong>g> expectati<strong>on</strong> <strong>and</strong> variance in Eq.(18) are zero. There<str<strong>on</strong>g>for</str<strong>on</strong>g>e, <str<strong>on</strong>g>the</str<strong>on</strong>g> above calculati<strong>on</strong> <strong>on</strong>ly<br />
makes sense <str<strong>on</strong>g>for</str<strong>on</strong>g> k ≥ 3. A separate exact calculati<strong>on</strong> <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> cycle shows, however, that inequalities (22)<br />
<strong>and</strong> (23) also hold <str<strong>on</strong>g>for</str<strong>on</strong>g> k = 2.<br />
From Eq.(21), we can also calculate <str<strong>on</strong>g>the</str<strong>on</strong>g> ratio <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> fixati<strong>on</strong> probabilities,<br />
ρ A<br />
= 1 + w · N − 1 {(k + 1)a + (k − 1)b − (k − 1)c − (k + 1)d}. (24)<br />
ρ B 2<br />
If k ≫ 1, <str<strong>on</strong>g>the</str<strong>on</strong>g>n inequality (22) leads to a + 2b > c + 2d, which is <str<strong>on</strong>g>the</str<strong>on</strong>g> 1/3 <str<strong>on</strong>g>rule</str<strong>on</strong>g> 3 . This <str<strong>on</strong>g>rule</str<strong>on</strong>g> works as<br />
follows. C<strong>on</strong>sider a game between two strategies, A <strong>and</strong> B, which are best replies to <str<strong>on</strong>g>the</str<strong>on</strong>g>mselves: a > c<br />
<strong>and</strong> d > b. In this case, <str<strong>on</strong>g>the</str<strong>on</strong>g> replicator equati<strong>on</strong> has an unstable equilibrium at x ∗ = (d−b)/(a−b−c+d)<br />
denoting <str<strong>on</strong>g>the</str<strong>on</strong>g> frequency <str<strong>on</strong>g>of</str<strong>on</strong>g> A. If x ∗ < 1/3, <str<strong>on</strong>g>the</str<strong>on</strong>g>n in an unstructured populati<strong>on</strong> (described by a complete<br />
graph) <str<strong>on</strong>g>the</str<strong>on</strong>g> fixati<strong>on</strong> probability ρ A will be greater than 1/N <str<strong>on</strong>g>for</str<strong>on</strong>g> weak selecti<strong>on</strong> <strong>and</strong> sufficiently large<br />
populati<strong>on</strong> size, N. The calculati<strong>on</strong> shown here has extended <str<strong>on</strong>g>the</str<strong>on</strong>g> 1/3 <str<strong>on</strong>g>rule</str<strong>on</strong>g> to any <strong>graphs</strong> with sufficiently<br />
large N.<br />
6
1.5 The <str<strong>on</strong>g>rule</str<strong>on</strong>g> b/c > k<br />
Let us now c<strong>on</strong>sider a game between cooperators, C, <strong>and</strong> defectors, D. The benefit <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> is b<br />
<strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g> cost is c. The pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f matrix takes <str<strong>on</strong>g>the</str<strong>on</strong>g> <str<strong>on</strong>g>for</str<strong>on</strong>g>m<br />
A<br />
⎛<br />
B<br />
⎞<br />
A a<br />
⎝<br />
b<br />
⎠ →<br />
B c d<br />
C D<br />
⎛ ⎞<br />
C b − c −c<br />
⎝ ⎠. (25)<br />
D b 0<br />
Substituting those pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f values into inequalities (22) <strong>and</strong> (23) leads to <str<strong>on</strong>g>the</str<strong>on</strong>g> c<strong>on</strong>clusi<strong>on</strong> that if b/c > k<br />
<str<strong>on</strong>g>the</str<strong>on</strong>g>n ρ C > 1/N > ρ D . Vice versa, we have if b/c < k <str<strong>on</strong>g>the</str<strong>on</strong>g>n ρ C < 1/N < ρ D . There<str<strong>on</strong>g>for</str<strong>on</strong>g>e, <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> is<br />
favored (<str<strong>on</strong>g>for</str<strong>on</strong>g> weak selecti<strong>on</strong> <strong>and</strong> large populati<strong>on</strong> size) if <strong>and</strong> <strong>on</strong>ly if<br />
b/c > k. (26)<br />
2 Imitati<strong>on</strong> (IM) updating<br />
Let us now study a different update <str<strong>on</strong>g>rule</str<strong>on</strong>g>. In any <strong>on</strong>e time step, a r<strong>and</strong>om individual is chosen to compare<br />
its pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f with those <str<strong>on</strong>g>of</str<strong>on</strong>g> its neighbors. The individual stays with its own strategy or imitates a neighbor’s<br />
strategy proporti<strong>on</strong>al to <str<strong>on</strong>g>the</str<strong>on</strong>g> pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f. In c<strong>on</strong>trast to ‘death-birth’ (DB) updating, here <str<strong>on</strong>g>the</str<strong>on</strong>g> pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
individual that is being updated also matters. We expect that this effect will introduce an advantage <str<strong>on</strong>g>for</str<strong>on</strong>g><br />
defectors, because defectors at <str<strong>on</strong>g>the</str<strong>on</strong>g> boundary <str<strong>on</strong>g>of</str<strong>on</strong>g> a cluster have a higher pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f than cooperators at <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
boundary <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g>re<str<strong>on</strong>g>for</str<strong>on</strong>g>e defectors are less likely to change <str<strong>on</strong>g>the</str<strong>on</strong>g>ir strategy.<br />
The fitness <str<strong>on</strong>g>of</str<strong>on</strong>g> a B-player with k A many A-players <strong>and</strong> k B many B-players in its neighborhood is<br />
given by<br />
The probability that <str<strong>on</strong>g>the</str<strong>on</strong>g> B player will adopt strategy A is given by<br />
f 0 = 1 − w + w(k A c + k B d). (27)<br />
k A f A<br />
k A f A + k B f B + f 0<br />
.<br />
The fitness <str<strong>on</strong>g>of</str<strong>on</strong>g> an A-player with k A many A-players <strong>and</strong> k B many B-players in its neighborhood is<br />
given by<br />
g 0 = 1 − w + w(k A a + k B b). (28)<br />
7
The probability that <str<strong>on</strong>g>the</str<strong>on</strong>g> A-player will adopt strategy B is given by<br />
k B g B<br />
k A g A + k B g B + g 0<br />
.<br />
Taking <str<strong>on</strong>g>the</str<strong>on</strong>g>se two modificati<strong>on</strong>s into account, a similar calculati<strong>on</strong> as in Secti<strong>on</strong> 1 leads to <str<strong>on</strong>g>the</str<strong>on</strong>g> following<br />
result: ρ A > 1/N if<br />
(k 2 + 4k + 3)a + (2k 2 + 2k − 3)b > (k 2 + k + 3)c + (2k 2 + 5k − 3)d. (29)<br />
Similarly, ρ B > 1/N if<br />
(k 2 + 4k + 3)d + (2k 2 + 2k − 3)c > (k 2 + k + 3)b + (2k 2 + 5k − 3)a. (30)<br />
Returning to <str<strong>on</strong>g>the</str<strong>on</strong>g> Pris<strong>on</strong>er’s Dilemma, given by pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f matrix (25), we find that ρ C > 1/N > ρ D if<br />
b/c > k + 2. (31)<br />
If instead b/c < k + 2 <str<strong>on</strong>g>the</str<strong>on</strong>g>n ρ D > 1/N > ρ C .<br />
3 ‘Birth-death’ (BD) updating<br />
Finally, we c<strong>on</strong>sider <str<strong>on</strong>g>the</str<strong>on</strong>g> following update <str<strong>on</strong>g>rule</str<strong>on</strong>g>: in each time step an individual is selected <str<strong>on</strong>g>for</str<strong>on</strong>g> reproducti<strong>on</strong><br />
proporti<strong>on</strong>al to fitness. The <str<strong>on</strong>g>of</str<strong>on</strong>g>fspring replaces a r<strong>and</strong>omly chosen neighbor.<br />
In order to derive <str<strong>on</strong>g>the</str<strong>on</strong>g> transiti<strong>on</strong> probabilities <str<strong>on</strong>g>of</str<strong>on</strong>g> p A <strong>and</strong> p AA we need to specify not <strong>on</strong>ly who reproduces<br />
but also <str<strong>on</strong>g>the</str<strong>on</strong>g> local c<strong>on</strong>figurati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> reproducing individual. The probability that an A-player<br />
who has k A many A-neighbors <strong>and</strong> k B many B-neighbors is selected <str<strong>on</strong>g>for</str<strong>on</strong>g> reproducti<strong>on</strong> is proporti<strong>on</strong>al to<br />
[<br />
] [<br />
]<br />
k!<br />
p A<br />
k A !k B ! q A|A k A k<br />
q B B|A · 1 − w + w(k A a + k B b) . (32)<br />
The first term is <str<strong>on</strong>g>the</str<strong>on</strong>g> frequency <str<strong>on</strong>g>of</str<strong>on</strong>g> such a c<strong>on</strong>figurati<strong>on</strong>. The sec<strong>on</strong>d term denotes <str<strong>on</strong>g>the</str<strong>on</strong>g> fitness <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> A-<br />
player. If <strong>on</strong>e <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> B-neighbors is replaced, <str<strong>on</strong>g>the</str<strong>on</strong>g>n <str<strong>on</strong>g>the</str<strong>on</strong>g> number <str<strong>on</strong>g>of</str<strong>on</strong>g> A-players increases by <strong>on</strong>e <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
number <str<strong>on</strong>g>of</str<strong>on</strong>g> AA-pairs increases by 1 + (k − 1)q A|B . Similarly, <str<strong>on</strong>g>the</str<strong>on</strong>g> probability that a B-player who has<br />
k A many A-neighbors <strong>and</strong> k B many B-neighbors is selected <str<strong>on</strong>g>for</str<strong>on</strong>g> reproducti<strong>on</strong> is proporti<strong>on</strong>al to<br />
[<br />
] [<br />
]<br />
k!<br />
p B<br />
k A !k B ! q A|B k A k<br />
q B B|B · 1 − w + w(k A c + k B d) . (33)<br />
8
If <strong>on</strong>e <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> A-neighbors is replaced, <str<strong>on</strong>g>the</str<strong>on</strong>g>n <str<strong>on</strong>g>the</str<strong>on</strong>g> number <str<strong>on</strong>g>of</str<strong>on</strong>g> A-players decreases by <strong>on</strong>e <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g> number <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
AA-pairs by (k − 1)q A|A .<br />
A similar calculati<strong>on</strong> as in Secti<strong>on</strong> 1 leads to <str<strong>on</strong>g>the</str<strong>on</strong>g> following result: ρ A > 1/N, if<br />
(k + 1)a + (2k − 1)b > (k + 1)c + (2k − 1)d. (34)<br />
Moreover, ρ B > 1/N, if<br />
(k + 1)d + (2k − 1)c > (k + 1)b + (2k − 1)a. (35)<br />
Applying inequalities (34) <strong>and</strong> (35) to <str<strong>on</strong>g>the</str<strong>on</strong>g> Pris<strong>on</strong>er’s Dilemma pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f matrix (25), we find that ρ D ><br />
1/N > ρ C always holds <str<strong>on</strong>g>for</str<strong>on</strong>g> any choice <str<strong>on</strong>g>of</str<strong>on</strong>g> cost <strong>and</strong> benefit, b > c > 0. Thus, selecti<strong>on</strong> never favors<br />
cooperators <str<strong>on</strong>g>for</str<strong>on</strong>g> BD updating.<br />
4 Computer simulati<strong>on</strong>s<br />
The different network structures are generated with ei<str<strong>on</strong>g>the</str<strong>on</strong>g>r N = 100 or N = 500 nodes <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g>n initialized<br />
with all defectors except <str<strong>on</strong>g>for</str<strong>on</strong>g> a single cooperator placed in a r<strong>and</strong>om locati<strong>on</strong>. At each time step, a<br />
r<strong>and</strong>omly chosen individual is updated according to two different <str<strong>on</strong>g>rule</str<strong>on</strong>g>s.<br />
In Figure 2 (main paper), we simulate death-birth (DB) updating. A r<strong>and</strong>om individual is chosen<br />
to die. The neighbors compete <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> empty site proporti<strong>on</strong>al to <str<strong>on</strong>g>the</str<strong>on</strong>g>ir pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f. In Figure 4 (<strong>on</strong>line), we<br />
simulate imitati<strong>on</strong> (IM) updating. A r<strong>and</strong>om individual is chosen to update its strategy. It will keep its<br />
current strategy or imitate a neighbor’s strategy proporti<strong>on</strong>al to pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f. For IM updating, <str<strong>on</strong>g>the</str<strong>on</strong>g> pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
<str<strong>on</strong>g>the</str<strong>on</strong>g> focal individual also plays a role.<br />
For each simulati<strong>on</strong> run, <str<strong>on</strong>g>the</str<strong>on</strong>g> respective update steps are repeated until ei<str<strong>on</strong>g>the</str<strong>on</strong>g>r cooperators have vanished<br />
or reached fixati<strong>on</strong>. The fixati<strong>on</strong> probability ρ C <str<strong>on</strong>g>of</str<strong>on</strong>g> cooperators is determined by <str<strong>on</strong>g>the</str<strong>on</strong>g> fracti<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
runs where cooperators reached fixati<strong>on</strong> out <str<strong>on</strong>g>of</str<strong>on</strong>g> 10 6 runs. The network structure was re-generated every<br />
10 3 runs to prevent any spurious results based <strong>on</strong> <strong>on</strong>e particular realizati<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> a specific network type.<br />
Cycles:<br />
On cycles, individuals interact with <str<strong>on</strong>g>the</str<strong>on</strong>g>ir k/2 nearest neighbors <strong>on</strong> ei<str<strong>on</strong>g>the</str<strong>on</strong>g>r side. C<strong>on</strong>sequently,<br />
odd degrees are not possible.<br />
Lattices: The three regular lattices in two dimensi<strong>on</strong>s are c<strong>on</strong>sidered: triangular, hexag<strong>on</strong>al <strong>and</strong> square.<br />
On square lattices we c<strong>on</strong>sider <str<strong>on</strong>g>the</str<strong>on</strong>g> st<strong>and</strong>ard neighborhoods, v<strong>on</strong> Neumann (k = 4) <strong>and</strong> Moore (k = 8).<br />
9
a cycle b lattice c r<strong>and</strong>om regular<br />
graph<br />
d r<strong>and</strong>om graph<br />
e scale-free network<br />
0.014<br />
0.014<br />
0.014<br />
0.014<br />
0.014<br />
fixati<strong>on</strong> probability ρ<br />
0.012<br />
0.01<br />
0.008<br />
0.012<br />
0.01<br />
0.008<br />
0.012<br />
0.01<br />
0.008<br />
0.012<br />
0.01<br />
0.008<br />
0.012<br />
0.01<br />
0.008<br />
0.006<br />
0.006<br />
0.006<br />
0.006<br />
0.006<br />
20<br />
2.5 5 7.5 10 12.5 15 17.5 20 2.5 5 7.5 10 12.5 15 17.5 20 2.5 5 7.5 10 12.5 15 17.5 20 2.5 5 7.5 10 12.5 15 17.5 2.5 5 7.5 10 12.5 15 17.5 20<br />
benefit-to-cost ratio b/c benefit-to-cost ratio b/c benefit-to-cost ratio b/c benefit-to-cost ratio b/c benefit-to-cost ratio b/c<br />
degree <str<strong>on</strong>g>of</str<strong>on</strong>g> nodes: k = 2 k = 3 k = 4 k = 6 k = 8 k = 10<br />
Figure 4: Fixati<strong>on</strong> probability ρ <str<strong>on</strong>g>of</str<strong>on</strong>g> a single cooperator in <str<strong>on</strong>g>the</str<strong>on</strong>g> imitati<strong>on</strong> process as a functi<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> benefitto-cost<br />
ratio b/c under weak selecti<strong>on</strong> (w = 0.01) <str<strong>on</strong>g>for</str<strong>on</strong>g> populati<strong>on</strong>s <str<strong>on</strong>g>of</str<strong>on</strong>g> N = 100 individuals <strong>on</strong> various types<br />
<str<strong>on</strong>g>of</str<strong>on</strong>g> <strong>graphs</strong> with different average numbers <str<strong>on</strong>g>of</str<strong>on</strong>g> neighbors, k. The top row shows <str<strong>on</strong>g>the</str<strong>on</strong>g> structure <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> graph<br />
<str<strong>on</strong>g>for</str<strong>on</strong>g> k = 2 (a) <strong>and</strong> (<strong>on</strong> average) k = 4 (b-e). The bottom row depicts simulati<strong>on</strong> data <str<strong>on</strong>g>for</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> fixati<strong>on</strong><br />
probability, ρ, <str<strong>on</strong>g>of</str<strong>on</strong>g> cooperators as determined by <str<strong>on</strong>g>the</str<strong>on</strong>g> fracti<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> runs where cooperators reached fixati<strong>on</strong><br />
out <str<strong>on</strong>g>of</str<strong>on</strong>g> 10 6 runs. In every time step, a focal site is r<strong>and</strong>omly selected <strong>and</strong> adopts a neighboring strategy<br />
with a probability proporti<strong>on</strong>al to <str<strong>on</strong>g>the</str<strong>on</strong>g> neighbors’ pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f or keeps its strategy proporti<strong>on</strong>al to <str<strong>on</strong>g>the</str<strong>on</strong>g> focal<br />
individual’s pay<str<strong>on</strong>g>of</str<strong>on</strong>g>f. The arrows mark b/c = k + 2 <strong>and</strong> <str<strong>on</strong>g>the</str<strong>on</strong>g> dotted line indicates <str<strong>on</strong>g>the</str<strong>on</strong>g> fixati<strong>on</strong> probability 1/N<br />
under neutral <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>.<br />
R<strong>and</strong>om-regular-<strong>graphs</strong>:<br />
For r<strong>and</strong>om regular <strong>graphs</strong> (RRG), <str<strong>on</strong>g>the</str<strong>on</strong>g> links between nodes are r<strong>and</strong>omly<br />
drawn under <str<strong>on</strong>g>the</str<strong>on</strong>g> c<strong>on</strong>straint that every node ends up with an equal number <str<strong>on</strong>g>of</str<strong>on</strong>g> links, k. Locally a RRG is<br />
similar to a tree (or Be<str<strong>on</strong>g>the</str<strong>on</strong>g> lattice) because <str<strong>on</strong>g>the</str<strong>on</strong>g> average loop size increases with N (Ref 4). Note that <str<strong>on</strong>g>the</str<strong>on</strong>g><br />
results from pair-approximati<strong>on</strong> are exact <str<strong>on</strong>g>for</str<strong>on</strong>g> Be<str<strong>on</strong>g>the</str<strong>on</strong>g> lattices but because <str<strong>on</strong>g>of</str<strong>on</strong>g> boundary problems <str<strong>on</strong>g>the</str<strong>on</strong>g>y are<br />
unsuitable <str<strong>on</strong>g>for</str<strong>on</strong>g> simulati<strong>on</strong>s <strong>and</strong> RRG’s serve as suitable substitutes. In order to ensure c<strong>on</strong>nectedness <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
<str<strong>on</strong>g>the</str<strong>on</strong>g> network, every node is first linked to a r<strong>and</strong>om node <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> already c<strong>on</strong>nected <strong>on</strong>es.<br />
R<strong>and</strong>om-<strong>graphs</strong>:<br />
R<strong>and</strong>om <strong>graphs</strong> (RG) are generated in much <str<strong>on</strong>g>the</str<strong>on</strong>g> same way as RRG, but relaxing<br />
<str<strong>on</strong>g>the</str<strong>on</strong>g> c<strong>on</strong>straint that every node has <str<strong>on</strong>g>the</str<strong>on</strong>g> same number <str<strong>on</strong>g>of</str<strong>on</strong>g> links to having k links <strong>on</strong> average. As <str<strong>on</strong>g>for</str<strong>on</strong>g> RRG,<br />
we first need to make sure that <str<strong>on</strong>g>the</str<strong>on</strong>g> graph is c<strong>on</strong>nected. In a sec<strong>on</strong>d step two r<strong>and</strong>omly drawn nodes are<br />
10
linked. The sec<strong>on</strong>d step is repeated until <str<strong>on</strong>g>the</str<strong>on</strong>g> desired average c<strong>on</strong>nectivity is reached.<br />
Scale-free networks: Scale-free networks are generated according to <str<strong>on</strong>g>the</str<strong>on</strong>g> method <str<strong>on</strong>g>of</str<strong>on</strong>g> preferential attachment<br />
5 . This leads to a degree distributi<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> P (k) = 2m 2 k −3 with k ≥ m <strong>and</strong> an average c<strong>on</strong>nectivity<br />
<str<strong>on</strong>g>of</str<strong>on</strong>g> 〈k〉 = 2m (see Ref 6).<br />
For interactive <strong>on</strong>line tutorials illustrating <str<strong>on</strong>g>the</str<strong>on</strong>g> dynamics <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> <strong>and</strong> defecti<strong>on</strong> <strong>on</strong> <strong>graphs</strong> <strong>and</strong><br />
<strong>social</strong> networks see Ref. 7.<br />
References<br />
[1] Matsuda, H., Ogita, N., Sasaki, A. & Satō, K. Statistical mechanics <str<strong>on</strong>g>of</str<strong>on</strong>g> populati<strong>on</strong>: The lattice Lotka-<br />
Volterra model. Prog. Theor. Phys. 88, 1035-1049 (1992).<br />
[2] Crow, J. F. & Kimura, M. Introducti<strong>on</strong> to Populati<strong>on</strong> Genetics Theory (Harper & Row, New York,<br />
1970).<br />
[3] Nowak, M. A., Sasaki, A., Taylor, C. & Fudenberg, D. Emergence <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>cooperati<strong>on</strong></str<strong>on</strong>g> <strong>and</strong> <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary<br />
stability in finite populati<strong>on</strong>s. Nature 428, 646-650 (2004).<br />
[4] Bollobás, B. R<strong>and</strong>om Graphs (Academic Press, New York, 1995).<br />
[5] Albert, R. & Barabási, A.-L. Statistical mechanics <str<strong>on</strong>g>of</str<strong>on</strong>g> complex networks. Rev. Mod. Phys. 74, 47-98<br />
(2002).<br />
[6] Klemm, K. & Eguíluz, V. M. Growing scale-free networks with small-world behavior. Phys. Rev. E<br />
65, 057102 (2002).<br />
[7] Hauert, C. VirtualLabs: Interactive tutorials <strong>on</strong> <str<strong>on</strong>g>evoluti<strong>on</strong></str<strong>on</strong>g>ary dynamics,<br />
http://www.univie.ac.at/virtuallabs (2005).<br />
11