On some properties of a differential operator on the polydisk

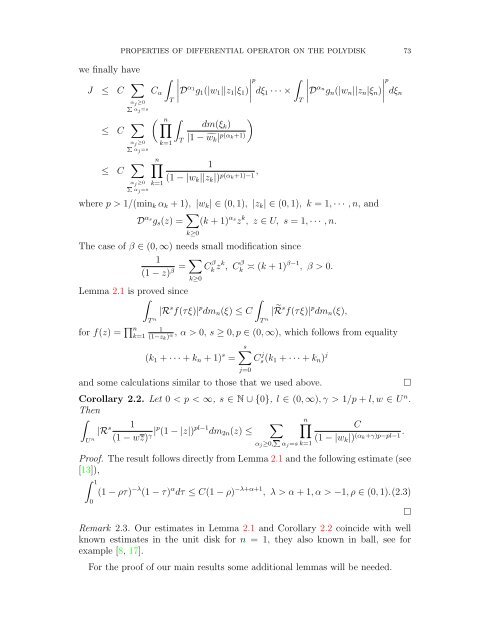
we finally have
J ≤ C ∑ α j ≥0
P αj =s
≤
≤
PROPERTIES OF DIFFERENTIAL OPERATOR ON THE POLYDISK 73
C ∑ α j ≥0
P αj =s
C ∑ α j ≥0
P αj =s
C α
∫
T
(
∏ n ∫
n∏
k=1
k=1
p ∫
∣ Dα 1
g 1 (|w 1 ||z 1 |ξ 1 )
∣ dξ 1 · · · ×
T
)
dm(ξ k )
|1 − ˜w k | p(α k+1)
1
(1 − |w k ||z k |) p(α k+1)−1 ,
T
p
∣ Dαn g n (|w n ||z n |ξ n )
∣ dξ n
where p > 1/(min k α k + 1), |w k | ∈ (0, 1), |z k | ∈ (0, 1), k = 1, · · · , n, and
D αs g s (z) = ∑ k≥0(k + 1) αs z k , z ∈ U, s = 1, · · · , n.
The case <strong>of</strong> β ∈ (0, ∞) needs small modification since
1
(1 − z) = ∑ C β β k zk , C β k ≍ (k + 1)β−1 , β > 0.
k≥0
Lemma 2.1 is proved since
∫
∫
|R s f(τξ)| p dm n (ξ) ≤ C | ˜R s f(τξ)| p dm n (ξ),
T n T n
for f(z) = ∏ n 1
k=1 (1−z k
, α > 0, s ≥ 0, p ∈ (0, ∞), which follows from equality
) α s∑
(k 1 + · · · + k n + 1) s = Cs(k j 1 + · · · + k n ) j
and <strong>some</strong> calculations similar to those that we used above.
j=0
Corollary 2.2. Let 0 < p < ∞, s ∈ N ∪ {0}, l ∈ (0, ∞), γ > 1/p + l, w ∈ U n .
Then
∫
|R s 1
∑ n∏
U (1 − wz) n γ |p (1 − |z|) pl−1 C
dm 2n (z) ≤
(1 − |w k |) .
(α k+γ)p−pl−1
α j ≥0, P α j =s k=1
Pro<strong>of</strong>. The result follows directly from Lemma 2.1 and the following estimate (see
[13]),
∫ 1
0
(1 − ρτ) −λ (1 − τ) α dτ ≤ C(1 − ρ) −λ+α+1 , λ > α + 1, α > −1, ρ ∈ (0, 1).(2.3)
Remark 2.3. Our estimates in Lemma 2.1 and Corollary 2.2 coincide with well
known estimates in the unit disk for n = 1, they also known in ball, see for
example [8, 17].
For the pro<strong>of</strong> <strong>of</strong> our main results <strong>some</strong> additional lemmas will be needed.
□
□