Inter-universal Teichmuller Theory I: Construction of Hodge Theaters

More documents

Recommendations

Info

88 SHINICHI MOCHIZUKI Note that the “desired geometry” in question will also be subject to other requirements. For instance, in [IUTchIII] [cf. also [IUTchII], §4], we shall make essential use of the global arithmetic — i.e., the ring structure and absolute Galois groups — of number fields. As observed above in Remark 4.3.1, (ii), these global arithmetic structures are not compatible with the “arithmetic local-analytic sections” constituted by the prime-strips. In particular, this state of affairs imposes the further requirement that the “geometry” in question be compatible with globalization, i.e., that it give rise to the global arithmetic of the number fields in question in a fashion that is independent of the various local geometries that appear in the “arithmetic local-analytic sections” constituted by the prime-strips, but nevertheless admits localization 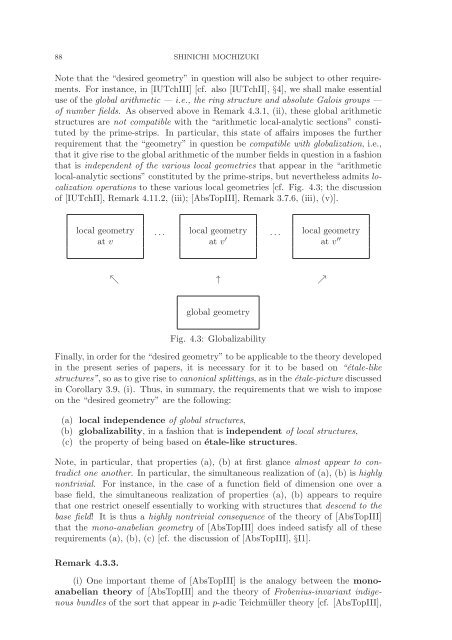operations to these various local geometries [cf. Fig. 4.3; the discussion of [IUTchII], Remark 4.11.2, (iii); [AbsTopIII], Remark 3.7.6, (iii), (v)]. local geometry at v ... local geometry ... local geometry at v ′ at v ′′ ↖ ↑ ↗ global geometry Fig. 4.3: Globalizability Finally, in order for the “desired geometry” to be applicable to the theory developed in the present series of papers, it is necessary for it to be based on “étale-like structures”, soastogiverisetocanonical splittings, asintheétale-picture discussed in Corollary 3.9, (i). Thus, in summary, the requirements that we wish to impose on the “desired geometry” are the following: (a) local independence of global structures, (b) globalizability, in a fashion that is independent of local structures, (c) the property of being based on étale-like structures. Note, in particular, that properties (a), (b) at first glance almost appear to contradict one another. In particular, the simultaneous realization of (a), (b) is highly nontrivial. For instance, in the case of a function field of dimension one over a base field, the simultaneous realization of properties (a), (b) appears to require that one restrict oneself essentially to working with structures that descend to the base field! It is thus a highly nontrivial consequence of the theory of [AbsTopIII] that the mono-anabelian geometry of [AbsTopIII] does indeed satisfy all of these requirements (a), (b), (c) [cf. the discussion of [AbsTopIII], §I1]. Remark 4.3.3. (i) One important theme of [AbsTopIII] is the analogy between the monoanabelian theory of [AbsTopIII] and the theory of Frobenius-invariant indigenous bundles of the sort that appear in p-adic Teichmüller theory [cf. [AbsTopIII],
INTER-UNIVERSAL TEICHMÜLLER THEORY I 89 §I5]. In fact, [although this point of view is not mentioned in [AbsTopIII]] one may “compose” this analogy with the analogy between the p-adic and complex theories discussed in [pOrd], Introduction; [pTeich], Introduction, §0, and consider the analogy between the mono-anabelian theory of [AbsTopIII] and the classical geometry of the upper half-plane H. In addition to being more elementary than the p-adic theory, this analogy with the classical geometry of the upper half-plane H also has the virtue that since it revolves around the canonical Kähler metric — i.e., the Poincaré metric— on the upper half-plane, it renders more transparent the relationship between the theory of the present series of papers and classical Arakelov theory [which also revolves, to a substantial extent, around Kähler metrics at the archimedean primes]. (ii) The essential content of the mono-anabelian theory of [AbsTopIII] may be summarized by the diagram Π k × log −→ k Π (∗) —wherek is a finite extension of Q p ; k is an algebraic closure of k; Π is the arithmetic fundamental group of a hyperbolic orbicurve over k; log is the p-adic logarithm [cf. [AbsTopIII], §I1]. On the other hand, if (E, ∇ E ) denotes the “tautological indigenous bundle” on H [i.e., the first de Rham cohomology of the tautological elliptic curve over H], then one has a natural Hodge filtration 0 → ω →E→τ → 0 [where ω, τ def = ω −1 are holomorphic line bundles on H], together with a natural complex conjugation operation ι E : E→E. The composite ω ↩→ E ι E −→ E ↠ τ then determines an Hermitian metric |−| ω on ω. For any trivializing section f of ω, the(1, 1)-form def κ H = 1 2πi ∂∂ log(|f| ω) is the canonical Kähler metric [i.e., Poincaré metric] on H. Then one can already readily identify various formal similarities between κ H and the diagram (∗) reviewed above: Indeed, at a purely formal [but by no means coincidental!] level, the “log” that appears in the definition of κ H is reminiscent of the “log-Frobenius operation” log. At a less formal level, the “Galois group” Π is reminiscent — cf. the point of view that “Galois groups are arithmetic tangent bundles”, a point of view that underlies the theory of the arithmetic Kodaira-Spencer morphism discussed in [HASurI]! — of ∂. If one thinks of complex conjugation as a sort of “archimedean Frobenius” [cf. [pTeich], Introduction, §0], then ∂ is reminiscent of the “Galois group” Π operating on the opposite side [cf. ι E ] of the log-Frobenius operation log. The Hodge filtration of E corresponds to the ring structures of the copies of k on either side of log [cf. the discussion of [AbsTopIII], Remark 3.7.2]. Finally, perhaps most importantly from the point of view of the theory of the present series of papers:
Page 1 and 2:
INTER-UNIVERSAL TEICHMÜLLER THEORY
Page 3 and 4:
Page 5 and 6:
Page 7 and 8:
Page 9 and 10:
Page 11 and 12:
Page 13 and 14:
Page 15 and 16:
associated to † F ⊩ mod and ‡
Page 17 and 18:
Page 19 and 20:
Page 21 and 22:
Page 23 and 24:
Page 25 and 26:
Page 27 and 28:
Page 29 and 30:
Page 31 and 32:
Page 33 and 34:
Page 35 and 36:
Page 37 and 38: INTER-UNIVERSAL TEICHMÜLLER THEORY
Page 81 and 82: completely determined by † D. INT
Page 87: INTER-UNIVERSAL TEICHMÜLLER THEORY
Page 97 and 98: conjugation by which maps φ NF IN
Page 129 and 130: (v) Write INTER-UNIVERSAL TEICHMÜL
Page 139 and 140:
Page 141 and 142:
Page 143 and 144:
Page 145 and 146:
Page 147 and 148:
Page 149 and 150:
Page 151 and 152:
Page 153 and 154:
Page 155 and 156:
Page 157:
show all

Inter-universal Teichmuller Theory I: Construction of Hodge Theaters

Create successful ePaper yourself

Delete template?

Save as template?