Inter-universal Teichmuller Theory I: Construction of Hodge Theaters

86 SHINICHI MOCHIZUKI
“arithmetic collections of local analytic sections” of Spec(K) → Spec(F )
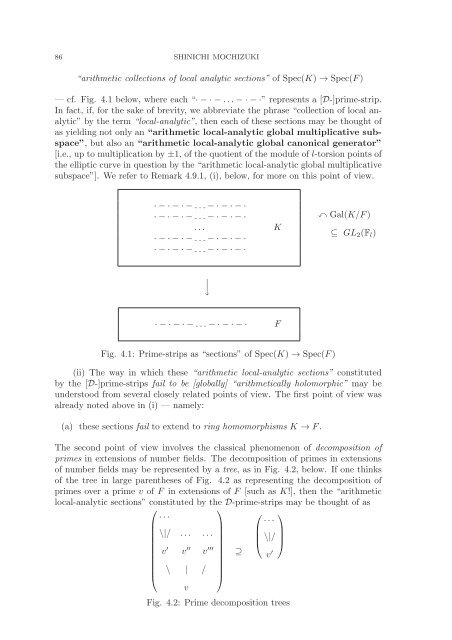
— cf. Fig. 4.1 below, where each “·−·−...−·−·” represents a [D-]prime-strip.
In fact, if, for the sake of brevity, we abbreviate the phrase “collection of local analytic”
by the term “local-analytic”, then each of these sections may be thought of
as yielding not only an “arithmetic local-analytic global multiplicative subspace”,
but also an “arithmetic local-analytic global canonical generator”
[i.e., up to multiplication by ±1, of the quotient of the module of l-torsion points of
the elliptic curve in question by the “arithmetic local-analytic global multiplicative
subspace”]. We refer to Remark 4.9.1, (i), below, for more on this point of view.
·−·−·−...−·−·−·
·−·−·−...−·−·−·
... K
·−·−·−...−·−·−·
·−·−·−...−·−·−·
Gal(K/F)
⊆ GL 2 (F l )
⏐
↓
·−·−·−...−·−·−·
F
Fig. 4.1: Prime-strips as “sections” of Spec(K) → Spec(F )
(ii) The way in which these “arithmetic local-analytic sections” constituted
by the [D-]prime-strips fail to be [globally] “arithmetically holomorphic” may be
understood from several closely related points of view. The first point of view was
already noted above in (i) — namely:
(a) these sections fail to extend to ring homomorphisms K → F .
The second point of view involves the classical phenomenon of decomposition of
primes in extensions of number fields. The decomposition of primes in extensions
of number fields may be represented by a tree, as in Fig. 4.2, below. If one thinks
of the tree in large parentheses of Fig. 4.2 as representing the decomposition of
primes over a prime v of F in extensions of F [such as K!], then the “arithmetic
local-analytic sections” constituted by the D-prime-strips may be thought of as
⎛
⎞
...
⎛ ⎞
...
\|/ ... ...
⎜
⎝ \|/ ⎟
⎠
v ′ v ′′ v ′′′
⊇
v ′ ⎜
⎝
\ | / ⎟
⎠
v
Fig. 4.2: Prime decomposition trees