Inter-universal Teichmuller Theory I: Construction of Hodge Theaters
Inter-universal Teichmuller Theory I: Construction of Hodge Theaters Inter-universal Teichmuller Theory I: Construction of Hodge Theaters
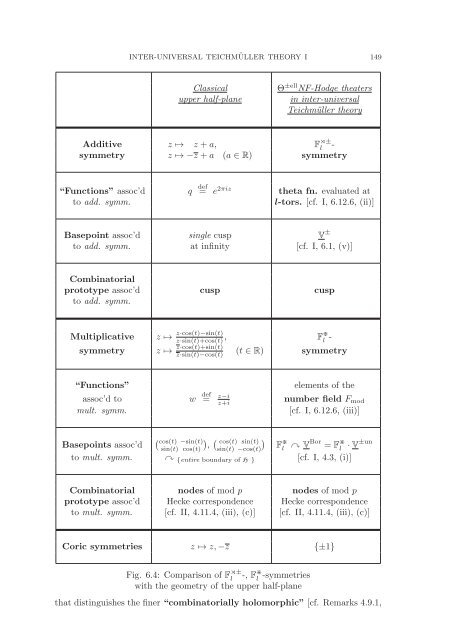
148 SHINICHI MOCHIZUKI the F l -symmetry is reminiscent of the fact that the multiplicative symmetries of the upper half-plane recalled above act transitively on the entire boundary of the upper half-plane. That is to say, taken as a whole, the geometry and coordinate naturally associated to this multiplicative symmetry of the upper half-plane may be thought of, at the level of “combinatorial prototypes”, as the geometric apparatus associated to a node, i.e., of the sort that occurs in the reduction modulo p of a Hecke correspondence [cf. the discussion of [IUTchII], Remark 4.11.4, (iii), (c); [NodNon], Introduction]. Finally, we note that, just as in the case of the F ⋊± l -, F l -symmetries discussed in the present paper, the only “coric” symmetries, i.e., symmetries common to both the additive and multiplicative symmetries of the upper half-plane recalled above, are the symmetries “{±1}” [i.e., the symmetries z ↦→ z,−z in the case of the upper half-plane]. The observations of the above discussion are summarized in Fig. 6.4 below. Remark 6.12.4. (i) Just as in the case of the F l -symmetry of Proposition 4.9, (i), the F⋊± l - symmetry of Proposition 6.8, (i), will eventually be applied, in the theory of the present series of papers [cf. theory of [IUTchII], [IUTchIII]], to establish an explicit network of comparison isomorphisms relating various objects — such as log-volumes — associated to the non-labeled prime-strips that are permuted by this symmetry [cf. the discussion of Remark 4.9.1, (i)]. Moreover, just as in the case of the F l -symmetry studied in §4 [cf. the discussion of Remark 4.9.2], one important property of this “network of comparison isomorphisms” is that it operates without “label crushing” [cf. Remark 4.9.2, (i)] — i.e., without disturbing the bijective relationship between the set of indices of the symmetrized collection of prime-strips and the set of labels ∈ T → ∼ F l under consideration. Finally, just as in the situation studied in §4, this crucial synchronization of labels is essentially a consequence of the single connected component — or, at a more abstract level, the single basepoint — of the global object [i.e., “ † D ⊚± ” in the present §6; “ † D ⊚ ”in§4] that appears in the [D-Θ ±ell -orD-ΘNF-] Hodge theater under consideration [cf. Remark 4.9.2, (ii)]. (ii) At a more concrete level, the “synchronization of labels” discussed in (i) is realized by means of the crucial bijections † ζ : LabCusp( † D ⊚ ) ∼ → J; † ζ ± : LabCusp ± ( † D ⊚± ) ∼ → T of Propositions 4.7, (iii); 6.5, (iii). Here, we pause to observe that it is precisely the existence of these bijections relating index sets of capsules of D-prime-strips to sets of global [±-]label classes of cusps
INTER-UNIVERSAL TEICHMÜLLER THEORY I 149 Classical upper half-plane Θ ±ell NF-Hodge theaters in inter-universal Teichmüller theory Additive z ↦→ z + a, F ⋊± l - symmetry z ↦→ −z + a (a ∈ R) symmetry “Functions” assoc’d to add. symm. q def = e 2πiz theta fn. evaluated at l-tors. [cf. I, 6.12.6, (ii)] Basepoint assoc’d single cusp V ± to add. symm. at infinity [cf. I, 6.1, (v)] Combinatorial prototype assoc’d cusp cusp to add. symm. Multiplicative symmetry z ↦→ z·cos(t)−sin(t) z·sin(t)+cos(t) , F l - z ↦→ z·cos(t)+sin(t) z·sin(t)−cos(t) (t ∈ R) symmetry “Functions” assoc’d to mult. symm. w def = z−i z+i elements of the number field F mod [cf. I, 6.12.6, (iii)] Basepoints assoc’d ( cos(t) −sin(t) ) ( sin(t) cos(t) , cos(t) sin(t) ) sin(t) −cos(t) F l V Bor = F l · V ±un to mult. symm. {entire boundary of H } [cf. I, 4.3, (i)] Combinatorial nodes of mod p nodes of mod p prototype assoc’d Hecke correspondence Hecke correspondence to mult. symm. [cf. II, 4.11.4, (iii), (c)] [cf. II, 4.11.4, (iii), (c)] Coric symmetries z ↦→ z,−z {±1} Fig. 6.4: Comparison of F ⋊± l -, F l -symmetries with the geometry of the upper half-plane that distinguishes the finer “combinatorially holomorphic” [cf. Remarks 4.9.1,
- Page 97 and 98: conjugation by which maps φ NF IN
- Page 99 and 100: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 101 and 102: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 103 and 104: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 105 and 106: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 107 and 108: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 109 and 110: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 111 and 112: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 113 and 114: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 115 and 116: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 117 and 118: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 119 and 120: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 121 and 122: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 123 and 124: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 125 and 126: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 127 and 128: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 129 and 130: (v) Write INTER-UNIVERSAL TEICHMÜL
- Page 131 and 132: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 133 and 134: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 135 and 136: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 137 and 138: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 139 and 140: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 141 and 142: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 143 and 144: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 145 and 146: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 147: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 151 and 152: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 153 and 154: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 155 and 156: INTER-UNIVERSAL TEICHMÜLLER THEORY
- Page 157: INTER-UNIVERSAL TEICHMÜLLER THEORY
INTER-UNIVERSAL TEICHMÜLLER THEORY I 149<br />
Classical<br />
upper half-plane<br />
Θ ±ell NF-<strong>Hodge</strong> theaters<br />
in inter-<strong>universal</strong><br />
Teichmüller theory<br />
Additive z ↦→ z + a, F ⋊±<br />
l<br />
-<br />
symmetry z ↦→ −z + a (a ∈ R) symmetry<br />
“Functions” assoc’d<br />
to add. symm.<br />
q def<br />
= e 2πiz theta fn. evaluated at<br />
l-tors. [cf. I, 6.12.6, (ii)]<br />
Basepoint assoc’d single cusp V ±<br />
to add. symm. at infinity [cf. I, 6.1, (v)]<br />
Combinatorial<br />
prototype assoc’d cusp cusp<br />
to add. symm.<br />
Multiplicative<br />
symmetry<br />
z ↦→ z·cos(t)−sin(t)<br />
z·sin(t)+cos(t) , F l -<br />
z ↦→ z·cos(t)+sin(t)<br />
z·sin(t)−cos(t)<br />
(t ∈ R) symmetry<br />
“Functions”<br />
assoc’d to<br />
mult. symm.<br />
w def<br />
= z−i<br />
z+i<br />
elements <strong>of</strong> the<br />
number field F mod<br />
[cf. I, 6.12.6, (iii)]<br />
Basepoints assoc’d<br />
( cos(t) −sin(t)<br />
) (<br />
sin(t) cos(t) , cos(t) sin(t)<br />
)<br />
sin(t) −cos(t)<br />
F l<br />
V Bor = F l<br />
· V ±un<br />
to mult. symm. {entire boundary <strong>of</strong> H } [cf. I, 4.3, (i)]<br />
Combinatorial nodes <strong>of</strong> mod p nodes <strong>of</strong> mod p<br />
prototype assoc’d Hecke correspondence Hecke correspondence<br />
to mult. symm. [cf. II, 4.11.4, (iii), (c)] [cf. II, 4.11.4, (iii), (c)]<br />
Coric symmetries z ↦→ z,−z {±1}<br />
Fig. 6.4: Comparison <strong>of</strong> F ⋊±<br />
l<br />
-, F l -symmetries<br />
with the geometry <strong>of</strong> the upper half-plane<br />
that distinguishes the finer “combinatorially holomorphic” [cf. Remarks 4.9.1,