Inter-universal Teichmuller Theory I: Construction of Hodge Theaters

INTER-UNIVERSAL TEICHMÜLLER THEORY I 139
whose output data admits a F ⋊±
l
-symmetry — i.e., more precisely, a symmetry
given by the action of a finite group that is equipped with a natural outer isomorphism
to F ⋊±
l
— which acts doubly transitively [i.e., transitively with stabilizers
of order two] on the index set [i.e., “T ”] of the underlying capsule of D-prime-strips
[i.e., “ † D T ”] of this output data.
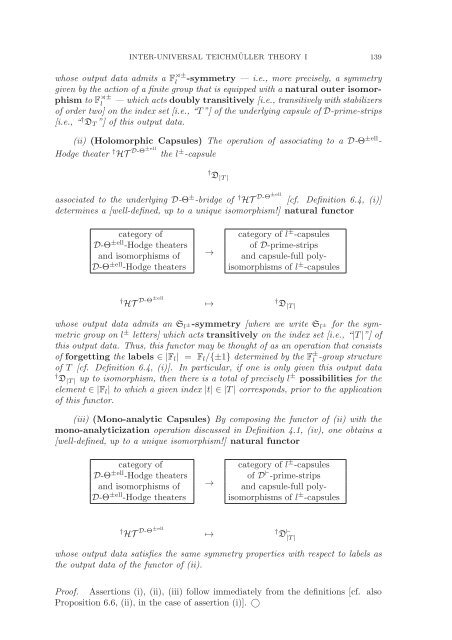
(ii) (Holomorphic Capsules) The operation of associating to a D-Θ ±ell -
Hodge theater † HT D-Θ±ell
the l ± -capsule
† D |T |
associated to the underlying D-Θ ± -bridge of † HT D-Θ±ell
[cf. Definition 6.4, (i)]
determines a [well-defined, up to a unique isomorphism!] natural functor
category of
D-Θ ±ell -Hodge theaters
and isomorphisms of
D-Θ ±ell -Hodge theaters
→
category of l ± -capsules
of D-prime-strips
and capsule-full polyisomorphisms
of l ± -capsules
† HT D-Θ±ell ↦→ † D |T |
whose output data admits an S l ±-symmetry [where we write S l
± for the symmetric
group on l ± letters] which acts transitively on the index set [i.e., “|T |”] of
this output data. Thus, this functor may be thought of as an operation that consists
of forgetting the labels ∈|F l | = F l /{±1} determined by the F ± l
-group structure
of T [cf. Definition 6.4, (i)]. In particular, if one is only given this output data
† D |T | up to isomorphism, then there is a total of precisely l ± possibilities for the
element ∈|F l | to which a given index |t| ∈|T | corresponds, prior to the application
of this functor.
(iii) (Mono-analytic Capsules) By composing the functor of (ii) with the
mono-analyticization operation discussed in Definition 4.1, (iv), one obtains a
[well-defined, up to a unique isomorphism!] natural functor
category of
D-Θ ±ell -Hodge theaters
and isomorphisms of
D-Θ ±ell -Hodge theaters
→
category of l ± -capsules
of D ⊢ -prime-strips
and capsule-full polyisomorphisms
of l ± -capsules
† HT D-Θ±ell ↦→ † D ⊢ |T |
whose output data satisfies the same symmetry properties with respect to labels as
the output data of the functor of (ii).
Proof. Assertions (i), (ii), (iii) follow immediately from the definitions [cf. also
Proposition 6.6, (ii), in the case of assertion (i)]. ○