Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
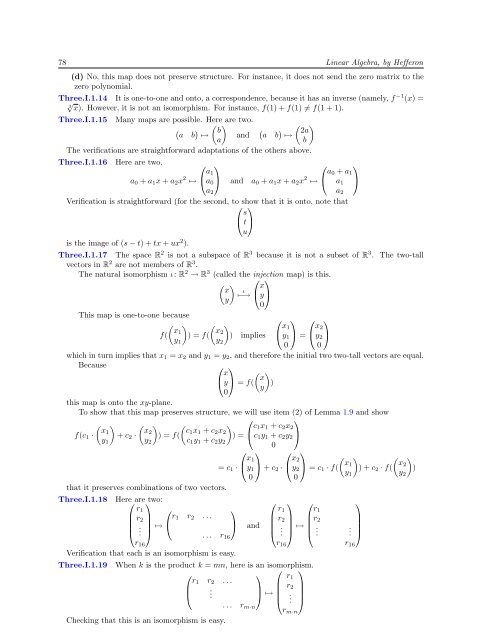
78 <strong>Linear</strong> <strong>Algebra</strong>, by Hefferon<br />
(d) No, this map does not preserve structure. For instance, it does not send the zero matrix to the<br />
zero polynomial.<br />
Three.I.1.14 It is one-to-one and onto, a correspondence, because it has an inverse (namely, f −1 (x) =<br />
3√ x). However, it is not an isomorphism. For instance, f(1) + f(1) ≠ f(1 + 1).<br />
Three.I.1.15 Many maps are possible. Here<br />
( )<br />
are two.<br />
( )<br />
( ) b ( ) 2a a b ↦→ and a b ↦→<br />
a<br />
b<br />
The verifications are straightforward adaptations of the others above.<br />
Three.I.1.16 Here are two. ⎛<br />
a 0 + a 1 x + a 2 x 2 ↦→ ⎝ a ⎞<br />
⎛<br />
1<br />
a 0<br />
⎠ and a 0 + a 1 x + a 2 x 2 ↦→ ⎝ a ⎞<br />
0 + a 1<br />
a 1<br />
⎠<br />
a 2 a 2<br />
Verification is straightforward (for the second, to show that it is onto, note that<br />
⎛ ⎞<br />
s<br />
⎝t⎠<br />
u<br />
is the image of (s − t) + tx + ux 2 ).<br />
Three.I.1.17 The space R 2 is not a subspace of R 3 because it is not a subset of R 3 . The two-tall<br />
vectors in R 2 are not members of R 3 .<br />
The natural isomorphism ι: R 2 → R 3 (called the injection map) is this.<br />
⎛<br />
(<br />
x ι<br />
↦−→ ⎝<br />
y) x ⎞<br />
y⎠<br />
0<br />
This map is one-to-one because<br />
⎛<br />
( ) ( )<br />
x1 x2<br />
f( ) = f( ) implies ⎝ x ⎞ ⎛<br />
1<br />
y<br />
y 1 y 1<br />
⎠ = ⎝ x ⎞<br />
2<br />
y 2<br />
⎠<br />
2<br />
0 0<br />
which in turn implies that x 1 = x 2 and y 1 = y 2 , and therefore the initial two two-tall vectors are equal.<br />
Because<br />
⎛<br />
⎝ x ⎞<br />
(<br />
y⎠ x<br />
= f( )<br />
y)<br />
0<br />
this map is onto the xy-plane.<br />
To show that this map preserves structure, we will use item (2) of Lemma 1.9 and show<br />
⎛<br />
( ) ( ) ( )<br />
x1 x2 c1 x<br />
f(c 1 · + c<br />
y 2 · ) = f( 1 + c 2 x 2<br />
) = ⎝ c ⎞<br />
1x 1 + c 2 x 2<br />
c<br />
1 y 2 c 1 y 1 + c 2 y 1 y 1 + c 2 y 2<br />
⎠<br />
2<br />
0<br />
⎛<br />
= c 1 · ⎝ x ⎞ ⎛<br />
1<br />
y 1<br />
⎠ + c 2 · ⎝ x ⎞<br />
) ( )<br />
2<br />
y 2<br />
⎠ x1<br />
x2<br />
= c 1 · f((<br />
) + c<br />
y 2 · f( )<br />
0<br />
0<br />
1 y 2<br />
that it preserves combinations of two vectors.<br />
Three.I.1.18 Here ⎛are⎞two:<br />
r 1 ⎛<br />
r 2<br />
⎜ ⎟<br />
⎝ . ⎠ ↦→ ⎝ r ⎞<br />
1 r 2 . . .<br />
⎠<br />
. . . r 16<br />
r 16<br />
Verification that each is an isomorphism is easy.<br />
and<br />
⎛ ⎞ ⎛<br />
r 1<br />
r 2<br />
⎜ ⎟<br />
⎝ . ⎠ ↦→ ⎜<br />
⎝ .<br />
r 16<br />
Three.I.1.19 When k is the product k = mn, here is an isomorphism.<br />
⎛ ⎞<br />
⎛<br />
⎞ r<br />
r 1 r 2 . . .<br />
1<br />
⎜<br />
⎝<br />
⎟<br />
r 2<br />
.<br />
⎠ ↦→ ⎜ ⎟<br />
⎝ . ⎠<br />
. . . r m·n<br />
r m·n<br />
Checking that this is an isomorphism is easy.<br />
r 1<br />
r 2<br />
⎞<br />
⎟<br />
. ⎠<br />
r 16