Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
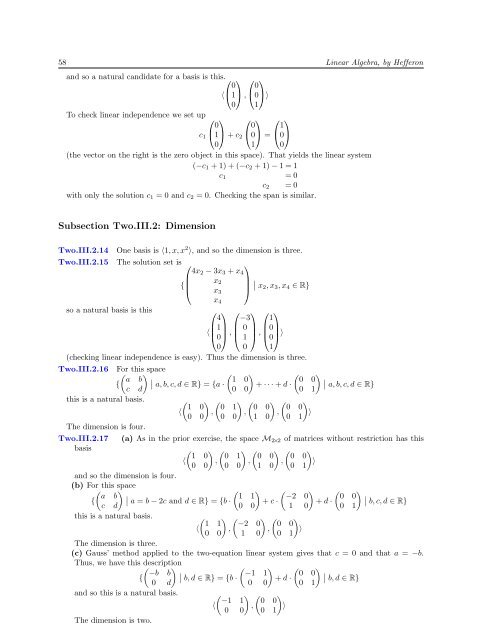
58 <strong>Linear</strong> <strong>Algebra</strong>, by Hefferon<br />
and so a natural candidate for a basis is this. ⎛ ⎞ ⎛ ⎞<br />
〈 ⎝ 0 1⎠ , ⎝ 0 0⎠〉<br />
0 1<br />
To check linear independence we set up<br />
⎛<br />
c 1<br />
⎝ 0 ⎞ ⎛<br />
1⎠ + c 2<br />
⎝ 0 ⎞ ⎛<br />
0⎠ = ⎝ 1 ⎞<br />
0⎠<br />
0 1 0<br />
(the vector on the right is the zero object in this space). That yields the linear system<br />
(−c 1 + 1) + (−c 2 + 1) − 1 = 1<br />
c 1 = 0<br />
c 2 = 0<br />
with only the solution c 1 = 0 and c 2 = 0. Checking the span is similar.<br />
Subsection Two.III.2: Dimension<br />
Two.III.2.14<br />
One basis is 〈1, x, x 2 〉, and so the dimension is three.<br />
Two.III.2.15 The solution set is ⎛<br />
⎞<br />
4x 2 − 3x 3 + x 4<br />
{ ⎜ x 2<br />
⎟ ∣<br />
⎝ x 3<br />
⎠ x 2 , x 3 , x 4 ∈ R}<br />
x 4<br />
so a natural basis is this<br />
⎛ ⎞ ⎛ ⎞ ⎛ ⎞<br />
4 −3 1<br />
〈 ⎜1<br />
⎟<br />
⎝0⎠ , ⎜ 0<br />
⎟<br />
⎝ 1 ⎠ , ⎜0<br />
⎟<br />
⎝0⎠ 〉<br />
0 0 1<br />
(checking linear independence is easy). Thus the dimension is three.<br />
Two.III.2.16 For<br />
(<br />
this<br />
)<br />
space<br />
( )<br />
a b ∣∣ 1 0<br />
{ a, b, c, d ∈ R} = {a · + · · · + d ·<br />
c d<br />
0 0<br />
this is a natural basis. ( ) ( ) ( )<br />
1 0 0 1 0 0<br />
〈 , , ,<br />
0 0 0 0 1 0<br />
The dimension is four.<br />
( )<br />
0 0 ∣∣<br />
a, b, c, d ∈ R}<br />
0 1<br />
( )<br />
0 0<br />
〉<br />
0 1<br />
Two.III.2.17 (a) As in the prior exercise, the space M 2×2 of matrices without restriction has this<br />
basis<br />
( ) ( ) ( ) ( )<br />
1 0 0 1 0 0 0 0<br />
〈 , , , 〉<br />
0 0 0 0 1 0 0 1<br />
and so the dimension is four.<br />
(b) For this space<br />
( ) ( ) ( ) ( )<br />
a b ∣∣ 1 1 −2 0 0 0 ∣∣<br />
{ a = b − 2c and d ∈ R} = {b · + c · + d · b, c, d ∈ R}<br />
c d<br />
0 0 1 0 0 1<br />
this is a natural basis.<br />
( ) ( ) ( )<br />
1 1 −2 0 0 0<br />
〈 , , 〉<br />
0 0 1 0 0 1<br />
The dimension is three.<br />
(c) Gauss’ method applied to the two-equation linear system gives that c = 0 and that a = −b.<br />
Thus, we have this<br />
(<br />
description<br />
) ( ) ( ) −b b ∣∣ −1 1 0 0 ∣∣<br />
{<br />
b, d ∈ R} = {b · + d · b, d ∈ R}<br />
0 d<br />
0 0 0 1<br />
and so this is a natural basis. ( ) ( )<br />
−1 1 0 0<br />
〈 , 〉<br />
0 0 0 1<br />
The dimension is two.