Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
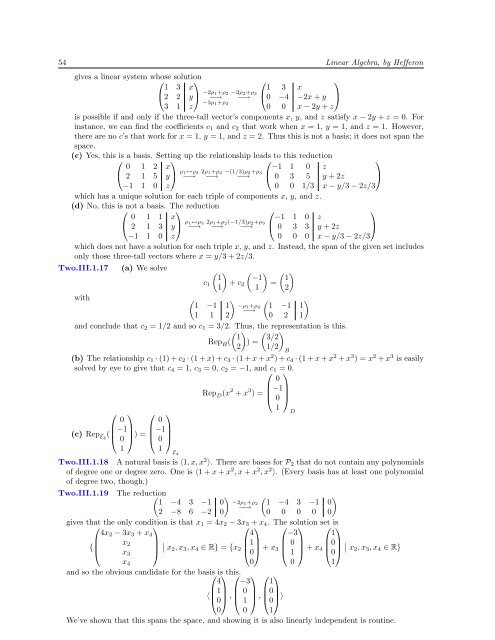
54 <strong>Linear</strong> <strong>Algebra</strong>, by Hefferon<br />
gives a linear system whose<br />
⎛<br />
solution<br />
⎝ 1 3 x<br />
⎞<br />
⎛<br />
2 2 y⎠ −2ρ1+ρ2 −2ρ 2+ρ 3<br />
−→ −→ ⎝ 1 3 x<br />
⎞<br />
0 −4 −2x + y ⎠<br />
−3ρ<br />
3 1 z<br />
1 +ρ 3<br />
0 0 x − 2y + z<br />
is possible if and only if the three-tall vector’s components x, y, and z satisfy x − 2y + z = 0. For<br />
instance, we can find the coefficients c 1 and c 2 that work when x = 1, y = 1, and z = 1. However,<br />
there are no c’s that work for x = 1, y = 1, and z = 2. Thus this is not a basis; it does not span the<br />
space.<br />
(c) Yes, this is a basis. Setting up the relationship leads to this reduction<br />
⎛<br />
⎝ 0 1 2 x<br />
⎞<br />
⎛<br />
⎞<br />
−1 1 0 z<br />
2 1 5 y⎠ ρ1↔ρ3<br />
−→ 2ρ1+ρ2<br />
−→<br />
−(1/3)ρ2+ρ3<br />
−→ ⎝ 0 3 5 y + 2z ⎠<br />
−1 1 0 z<br />
0 0 1/3 x − y/3 − 2z/3<br />
which has a unique solution for each triple of components x, y, and z.<br />
(d) No, this is not a basis. The reduction<br />
⎛<br />
⎝ 0 1 1 x<br />
⎞<br />
⎛<br />
2 1 3 y⎠ ρ 1↔ρ 3 2ρ 1 +ρ 2 (−1/3)ρ 2 +ρ 3<br />
−→ −→ −→ ⎝ −1 1 0 z<br />
⎞<br />
0 3 3 y + 2z ⎠<br />
−1 1 0 z<br />
0 0 0 x − y/3 − 2z/3<br />
which does not have a solution for each triple x, y, and z. Instead, the span of the given set includes<br />
only those three-tall vectors where x = y/3 + 2z/3.<br />
Two.III.1.17<br />
(a) We solve<br />
)<br />
( ) (<br />
−1 1<br />
+ c 2 =<br />
1 2)<br />
(<br />
1<br />
c 1<br />
1<br />
with ( ) ( )<br />
1 −1 1 −ρ 1+ρ 2 1 −1 1<br />
−→<br />
1 1 2 0 2 1<br />
and conclude that c 2 = 1/2 and so c 1 = 3/2. Thus, the representation is this.<br />
( ( )<br />
1 3/2<br />
Rep B ( ) =<br />
2)<br />
1/2<br />
B<br />
(b) The relationship c 1 · (1) + c 2 · (1 + x) + c 3 · (1 + x + x 2 ) + c 4 · (1 + x + x 2 + x 3 ) = x 2 + x 3 is easily<br />
solved by eye to give that c 4 = 1, c 3 = 0, c 2 = −1, and c 1 = 0.<br />
⎛ ⎞ ⎛ ⎞<br />
0 0<br />
(c) Rep E4<br />
( ⎜−1<br />
⎟<br />
⎝ 0 ⎠ ) = ⎜−1<br />
⎟<br />
⎝ 0 ⎠<br />
1 1<br />
E 4<br />
⎛ ⎞<br />
0<br />
Rep D (x 2 + x 3 ) = ⎜−1<br />
⎟<br />
⎝ 0 ⎠<br />
1<br />
Two.III.1.18 A natural basis is 〈1, x, x 2 〉. There are bases for P 2 that do not contain any polynomials<br />
of degree one or degree zero. One is 〈1 + x + x 2 , x + x 2 , x 2 〉. (Every basis has at least one polynomial<br />
of degree two, though.)<br />
Two.III.1.19 The reduction ( ) ( )<br />
1 −4 3 −1 0 −2ρ 1 +ρ 2 1 −4 3 −1 0<br />
−→<br />
2 −8 6 −2 0<br />
0 0 0 0 0<br />
gives that<br />
⎛<br />
the only condition<br />
⎞<br />
is that x 1 = 4x 2 − 3x<br />
⎛ 3 +<br />
⎞<br />
x 4 . The<br />
⎛<br />
solution<br />
⎞<br />
set<br />
⎛<br />
is<br />
⎞<br />
4x 2 − 3x 3 + x 4<br />
4 −3 1<br />
{ ⎜ x 2<br />
⎟ ∣<br />
⎝ x 3<br />
⎠ x 2 , x 3 , x 4 ∈ R} = {x 2<br />
⎜1<br />
⎟<br />
⎝0⎠ + x ⎜ 0<br />
⎟<br />
3 ⎝ 1 ⎠ + x ⎜0<br />
⎟ ∣<br />
4 ⎝0⎠<br />
x 2 , x 3 , x 4 ∈ R}<br />
x 4<br />
0 0 1<br />
and so the obvious candidate for the basis ⎛is⎞<br />
this. ⎛ ⎞ ⎛ ⎞<br />
4 −3 1<br />
〈 ⎜1<br />
⎟<br />
⎝0⎠ , ⎜ 0<br />
⎟<br />
⎝ 1 ⎠ , ⎜0<br />
⎟<br />
⎝0⎠ 〉<br />
0 0 1<br />
We’ve shown that this spans the space, and showing it is also linearly independent is routine.<br />
D