Linear Algebra Exercises-n-Answers.pdf

More documents

Recommendations

Info

202 Linear Algebra, by Hefferon (c) The minimal polynomial m(t) sends every vector in the domain to zero and so it is not one-to-one (except in a trivial space, which we ignore). By the first item of this question, since the composition m(t) is not one-to-one, at least one of the components t − λ i is not one-to-one. By the second item, t − λ i has a nontrivial nullspace. Because (t − λ i )(⃗v) = ⃗0 holds if and only if t(⃗v) = λ i · ⃗v, the prior sentence gives that λ i is an eigenvalue (recall that the definition of eigenvalue requires that the relationship hold for at least one nonzero ⃗v). Five.IV.1.30 This is false. The natural example of a non-diagonalizable transformation works here. Consider the transformation of C 2 represented with respect to the standard basis by this matrix. ( ) 0 1 N = 0 0 The characteristic polynomial is c(x) = x 2 . Thus the minimal polynomial is either m 1 (x) = x or m 2 (x) = x 2 . The first is not right since N − 0 · I is not the zero matrix, thus in this example the minimal polynomial has degree equal to the dimension of the underlying space, and, as mentioned, we know this matrix is not diagonalizable because it is nilpotent. Five.IV.1.31 Let A and B be similar A = P BP −1 . From the facts that A n = (P BP −1 ) n = (P BP −1 )(P BP −1 ) · · · (P BP −1 ) = P B(P −1 P )B(P −1 P ) · · · (P −1 P )BP −1 = P B n P −1 and c · A = c · (P BP −1 ) = P (c · B)P −1 follows the required fact that for any polynomial function f we have f(A) = P f(B) P −1 . For instance, if f(x) = x 2 + 2x + 3 then A 2 + 2A + 3I = (P BP −1 ) 2 + 2 · P BP −1 + 3 · I = (P BP −1 )(P BP −1 ) + P (2B)P −1 + 3 · P P −1 = P (B 2 + 2B + 3I)P −1 shows that f(A) is similar to f(B). (a) Taking f to be a linear polynomial we have that A − xI is similar to B − xI. Similar matrices have equal determinants (since |A| = |P BP −1 | = |P | · |B| · |P −1 | = 1 · |B| · 1 = |B|). Thus the characteristic polynomials are equal. (b) As P and P −1 are invertible, f(A) is the zero matrix when, and only when, f(B) is the zero matrix. (c) They cannot be similar since they don’t have the same characteristic polynomial. The characteristic polynomial of the first one is x 2 − 4x − 3 while the characteristic polynomial of the second is x 2 − 5x + 5. Five.IV.1.32 Suppose that m(x) = x n + m n−1 x n−1 + · · · + m 1 x + m 0 is minimal for T . (a) For the ‘if’ argument, because T n + · · · + m 1 T + m 0 I is the zero matrix we have that I = (T n +· · ·+m 1 T )/(−m 0 ) = T ·(T n−1 +· · ·+m 1 I)/(−m 0 ) and so the matrix (−1/m 0 )·(T n−1 +· · ·+m 1 I) is the inverse of T . For ‘only if’, suppose that m 0 = 0 (we put the n = 1 case aside but it is easy) so that T n + · · · + m 1 T = (T n−1 + · · · + m 1 I)T is the zero matrix. Note that T n−1 + · · · + m 1 I is not the zero matrix because the degree of the minimal polynomial is n. If T −1 exists then multiplying both (T n−1 + · · · + m 1 I)T and the zero matrix from the right by T −1 gives a contradiction. (b) If T is not invertible then the constant term in its minimal polynomial is zero. Thus, T n + · · · + m 1 T = (T n−1 + · · · + m 1 I)T = T (T n−1 + · · · + m 1 I) is the zero matrix. Five.IV.1.33 (a) For the inductive step, assume that Lemma 1.7 is true for polynomials of degree i, . . . , k − 1 and consider a polynomial f(x) of degree k. Factor f(x) = k(x − λ 1 ) q1 · · · (x − λ l ) q l and let k(x − λ 1 ) q 1−1 · · · (x − λ l ) q l be c n−1 x n−1 + · · · + c 1 x + c 0 . Substitute: k(t − λ 1 ) q1 ◦ · · · ◦ (t − λ l ) q l (⃗v) = (t − λ 1 ) ◦ (t − λ 1 ) q1 ◦ · · · ◦ (t − λ l ) q l (⃗v) = (t − λ 1 ) (c n−1 t n−1 (⃗v) + · · · + c 0 ⃗v) = f(t)(⃗v) (the second equality follows from the inductive hypothesis and the third from the linearity of t). (b) One example is to consider the squaring map s: R → R given by s(x) = x 2 . It is nonlinear. The action defined by the polynomial f(t) = t 2 − 1 changes s to f(s) = s 2 − 1, which is this map. x s2 −1 ↦−→ s ◦ s(x) − 1 = x 4 − 1 Observe that this map differs from the map (s − 1) ◦ (s + 1); for instance, the first map takes x = 5 to 624 while the second one takes x = 5 to 675.
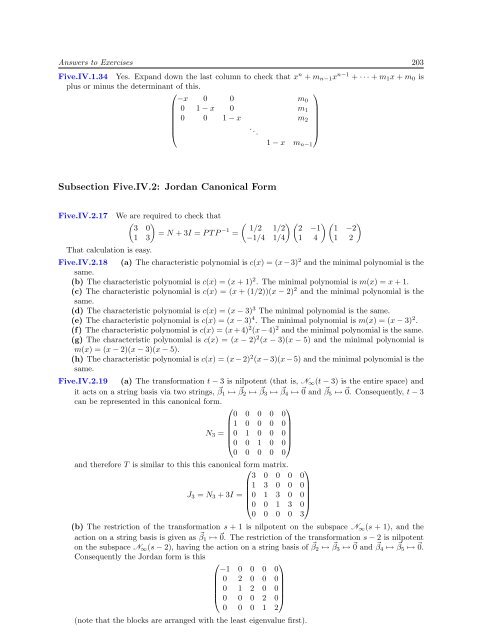
Answers to Exercises 203 Five.IV.1.34 Yes. Expand down the last column to check that x n + m n−1 x n−1 + · · · + m 1 x + m 0 is plus or minus the determinant of this. ⎛ ⎞ −x 0 0 m 0 0 1 − x 0 m 1 0 0 1 − x m 2 ⎜ ⎝ . .. ⎟ ⎠ 1 − x m n−1 Subsection Five.IV.2: Jordan Canonical Form Five.IV.2.17 That calculation is easy. We are required to check that ( ) 3 0 = N + 3I = P T P −1 = 1 3 ( 1/2 1/2 −1/4 1/4 ) ( 2 −1 1 4 ) ( 1 −2 1 2 Five.IV.2.18 (a) The characteristic polynomial is c(x) = (x−3) 2 and the minimal polynomial is the same. (b) The characteristic polynomial is c(x) = (x + 1) 2 . The minimal polynomial is m(x) = x + 1. (c) The characteristic polynomial is c(x) = (x + (1/2))(x − 2) 2 and the minimal polynomial is the same. (d) The characteristic polynomial is c(x) = (x − 3) 3 The minimal polynomial is the same. (e) The characteristic polynomial is c(x) = (x − 3) 4 . The minimal polynomial is m(x) = (x − 3) 2 . (f) The characteristic polynomial is c(x) = (x + 4) 2 (x − 4) 2 and the minimal polynomial is the same. (g) The characteristic polynomial is c(x) = (x − 2) 2 (x − 3)(x − 5) and the minimal polynomial is m(x) = (x − 2)(x − 3)(x − 5). (h) The characteristic polynomial is c(x) = (x − 2) 2 (x − 3)(x − 5) and the minimal polynomial is the same. Five.IV.2.19 (a) The transformation t − 3 is nilpotent (that is, N ∞ (t − 3) is the entire space) and it acts on a string basis via two strings, β ⃗ 1 ↦→ β ⃗ 2 ↦→ β ⃗ 3 ↦→ β ⃗ 4 ↦→ ⃗0 and β ⃗ 5 ↦→ ⃗0. Consequently, t − 3 can be represented in this canonical form. ⎛ ⎞ 0 0 0 0 0 1 0 0 0 0 N 3 = ⎜0 1 0 0 0 ⎟ ⎝0 0 1 0 0⎠ 0 0 0 0 0 and therefore T is similar to this this canonical form matrix. ⎛ ⎞ 3 0 0 0 0 1 3 0 0 0 J 3 = N 3 + 3I = ⎜0 1 3 0 0 ⎟ ⎝0 0 1 3 0⎠ 0 0 0 0 3 (b) The restriction of the transformation s + 1 is nilpotent on the subspace N ∞ (s + 1), and the action on a string basis is given as β ⃗ 1 ↦→ ⃗0. The restriction of the transformation s − 2 is nilpotent on the subspace N ∞ (s − 2), having the action on a string basis of β ⃗ 2 ↦→ β ⃗ 3 ↦→ ⃗0 and β ⃗ 4 ↦→ β ⃗ 5 ↦→ ⃗0. Consequently the Jordan form is this ⎛ ⎞ −1 0 0 0 0 0 2 0 0 0 ⎜ 0 1 2 0 0 ⎟ ⎝ 0 0 0 2 0⎠ 0 0 0 1 2 (note that the blocks are arranged with the least eigenvalue first). )
Page 1 and 2:
Answers to Exercises Linear Algebra
Page 3:
These are answers to the exercises
Page 6 and 7:
4 Linear Algebra, by Hefferon Topic
Page 8 and 9:
6 Linear Algebra, by Hefferon One.I
Page 10 and 11:
8 Linear Algebra, by Hefferon (this
Page 12 and 13:
10 Linear Algebra, by Hefferon from
Page 14 and 15:
12 Linear Algebra, by Hefferon (d)
Page 16 and 17:
14 Linear Algebra, by Hefferon One.
Page 18 and 19:
16 Linear Algebra, by Hefferon so t
Page 20 and 21:
18 Linear Algebra, by Hefferon ⎛
Page 22 and 23:
20 Linear Algebra, by Hefferon inst
Page 24 and 25:
Page 26 and 27:
24 Linear Algebra, by Hefferon and
Page 28 and 29:
Page 30 and 31:
28 Linear Algebra, by Hefferon (a)
Page 32 and 33:
30 Linear Algebra, by Hefferon The
Page 34 and 35:
32 Linear Algebra, by Hefferon > A:
Page 36 and 37:
34 Linear Algebra, by Hefferon Topi
Page 38 and 39:
36 Linear Algebra, by Hefferon 65 5
Page 40 and 41:
38 Linear Algebra, by Hefferon Two.
Page 42 and 43:
40 Linear Algebra, by Hefferon oute
Page 44 and 45:
42 Linear Algebra, by Hefferon (e)
Page 46 and 47:
44 Linear Algebra, by Hefferon Asso
Page 48 and 49:
46 Linear Algebra, by Hefferon of B
Page 50 and 51:
48 Linear Algebra, by Hefferon (c)
Page 52 and 53:
50 Linear Algebra, by Hefferon Poss
Page 54 and 55:
52 Linear Algebra, by Hefferon Repe
Page 56 and 57:
54 Linear Algebra, by Hefferon give
Page 58 and 59:
56 Linear Algebra, by Hefferon (b)
Page 60 and 61:
Page 62 and 63:
Page 64 and 65:
Page 66 and 67:
Page 68 and 69:
66 Linear Algebra, by Hefferon But
Page 70 and 71:
Page 72 and 73:
70 Linear Algebra, by Hefferon 2 Fo
Page 74 and 75:
72 Linear Algebra, by Hefferon -a T
Page 76 and 77:
74 Linear Algebra, by Hefferon 3 (a
Page 78 and 79:
Page 80 and 81:
Page 82 and 83:
80 Linear Algebra, by Hefferon Thre
Page 84 and 85:
82 Linear Algebra, by Hefferon has
Page 86 and 87:
Page 88 and 89:
Page 90 and 91:
Page 92 and 93:
90 Linear Algebra, by Hefferon ∣
Page 94 and 95:
Page 96 and 97:
Page 98 and 99:
96 Linear Algebra, by Hefferon and,
Page 100 and 101:
98 Linear Algebra, by Hefferon The
Page 102 and 103:
Page 104 and 105:
102 Linear Algebra, by Hefferon mea
Page 106 and 107:
Page 108 and 109:
106 Linear Algebra, by Hefferon Thr
Page 110 and 111:
108 Linear Algebra, by Hefferon (e)
Page 112 and 113:
Page 114 and 115:
112 Linear Algebra, by Hefferon to
Page 116 and 117:
Page 118 and 119:
Page 120 and 121:
118 Linear Algebra, by Hefferon As
Page 122 and 123:
Page 124 and 125:
122 Linear Algebra, by Hefferon we
Page 126 and 127:
Page 128 and 129:
126 Linear Algebra, by Hefferon As
Page 130 and 131:
Page 132 and 133:
130 Linear Algebra, by Hefferon the
Page 134 and 135:
Page 136 and 137:
and that (⃗v − (c1 · ⃗κ 1 +
Page 138 and 139:
Page 140 and 141:
138 Linear Algebra, by Hefferon the
Page 142 and 143:
Page 144 and 145:
Page 146 and 147:
144 Linear Algebra, by Hefferon Pro
Page 148 and 149:
146 Linear Algebra, by Hefferon 5 T
Page 150 and 151:
148 Linear Algebra, by Hefferon Top
Page 152 and 153:
150 Linear Algebra, by Hefferon 4 R
Page 154 and 155: 152 Linear Algebra, by Hefferon > 0
Page 156 and 157: 154 Linear Algebra, by Hefferon 0.5
Page 158 and 159: 156 Linear Algebra, by Hefferon n =
Page 160 and 161: 158 Linear Algebra, by Hefferon Top
Page 162 and 163: 160 Linear Algebra, by Hefferon and
Page 164 and 165: 162 Linear Algebra, by Hefferon ∣
Page 166 and 167: 164 Linear Algebra, by Hefferon Fou
Page 170 and 171: 168 Linear Algebra, by Hefferon The
Page 174 and 175: 172 Linear Algebra, by Hefferon ∣
Page 176 and 177: 174 Linear Algebra, by Hefferon whe
Page 178 and 179: 176 Linear Algebra, by Hefferon 0.2
Page 180 and 181: 178 Linear Algebra, by Hefferon Als
Page 182 and 183: 180 Linear Algebra, by Hefferon Fiv
Page 184 and 185: 182 Linear Algebra, by Hefferon Res
Page 186 and 187: 184 Linear Algebra, by Hefferon The
Page 188 and 189: 186 Linear Algebra, by Hefferon (as
Page 192 and 193: 190 Linear Algebra, by Hefferon to
Page 196 and 197: 194 Linear Algebra, by Hefferon and
Page 198 and 199: 196 Linear Algebra, by Hefferon tha
Page 200 and 201: 198 Linear Algebra, by Hefferon Aga
Page 202 and 203: 200 Linear Algebra, by Hefferon Bec
Page 208 and 209: 206 Linear Algebra, by Hefferon For
show all

Linear Algebra Exercises-n-Answers.pdf

Create successful ePaper yourself

Delete template?

Save as template?