Linear Algebra Exercises-n-Answers.pdf

Answers to Exercises 199
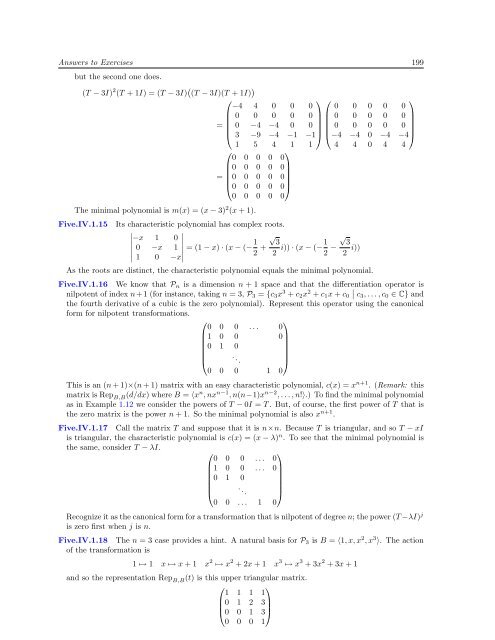
but the second one does.
(T − 3I) 2 (T + 1I) = (T − 3I) ( (T − 3I)(T + 1I) )
⎛
⎞ ⎛
⎞
−4 4 0 0 0 0 0 0 0 0
0 0 0 0 0
0 0 0 0 0
=
⎜ 0 −4 −4 0 0
⎟ ⎜ 0 0 0 0 0
⎟
⎝ 3 −9 −4 −1 −1⎠
⎝−4 −4 0 −4 −4⎠
1 5 4 1 1 4 4 0 4 4
⎛
⎞
0 0 0 0 0
0 0 0 0 0
=
⎜0 0 0 0 0
⎟
⎝0 0 0 0 0⎠
0 0 0 0 0
The minimal polynomial is m(x) = (x − 3) 2 (x + 1).
Five.IV.1.15
Its characteristic polynomial has complex roots.
−x 1 0
√ √ 0 −x 1
3
3
∣ 1 0 −x∣ = (1 − x) · (x − (−1 2 + 2 i)) · (x − (−1 2 − 2 i))
As the roots are distinct, the characteristic polynomial equals the minimal polynomial.
Five.IV.1.16 We know that P n is a dimension n + 1 space and that the differentiation ∣ operator is
nilpotent of index n+1 (for instance, taking n = 3, P 3 = {c 3 x 3 + c 2 x 2 + c 1 x + c 0 c 3 , . . . , c 0 ∈ C} and
the fourth derivative of a cubic is the zero polynomial). Represent this operator using the canonical
form for nilpotent transformations.
⎛
⎞
0 0 0 . . . 0
1 0 0 0
0 1 0
⎜
⎝
. ..
⎟
⎠
0 0 0 1 0
This is an (n + 1)×(n + 1) matrix with an easy characteristic polynomial, c(x) = x n+1 . (Remark: this
matrix is Rep B,B (d/dx) where B = 〈x n , nx n−1 , n(n−1)x n−2 , . . . , n!〉.) To find the minimal polynomial
as in Example 1.12 we consider the powers of T − 0I = T . But, of course, the first power of T that is
the zero matrix is the power n + 1. So the minimal polynomial is also x n+1 .
Five.IV.1.17 Call the matrix T and suppose that it is n×n. Because T is triangular, and so T − xI
is triangular, the characteristic polynomial is c(x) = (x − λ) n . To see that the minimal polynomial is
the same, consider T − λI.
⎛
⎞
0 0 0 . . . 0
1 0 0 . . . 0
0 1 0
⎜
⎝
. ..
⎟
⎠
0 0 . . . 1 0
Recognize it as the canonical form for a transformation that is nilpotent of degree n; the power (T −λI) j
is zero first when j is n.
Five.IV.1.18 The n = 3 case provides a hint. A natural basis for P 3 is B = 〈1, x, x 2 , x 3 〉. The action
of the transformation is
1 ↦→ 1 x ↦→ x + 1 x 2 ↦→ x 2 + 2x + 1 x 3 ↦→ x 3 + 3x 2 + 3x + 1
and so the representation Rep B,B (t) is this upper triangular matrix.
⎛ ⎞
1 1 1 1
⎜0 1 2 3
⎟
⎝0 0 1 3⎠
0 0 0 1