Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf Linear Algebra Exercises-n-Answers.pdf
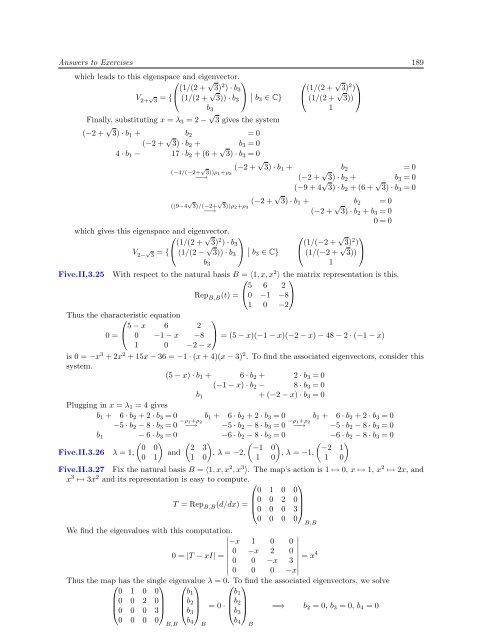
188 Linear Algebra, by Hefferon Five.II.3.24 (a) The characteristic equation is 3 − x −2 0 0 = −2 3 − x 0 ∣ 0 0 5 − x∣ − 11x 2 + 35x − 25 = (x − 1)(x − 5) 2 and so the eigenvalues are λ 1 = 1 and also the repeated eigenvalue λ 2 = 5. To find eigenvectors, consider this system. (3 − x) · b 1 − 2 · b 2 = 0 −2 · b 1 + (3 − x) · b 2 = 0 (5 − x) · b 3 = 0 For λ 1 = 1 we get 2 · b 1 − 2 · b 2 = 0 −2 · b 1 + 2 · b 2 = 0 4 · b 3 = 0 leading to this eigenspace and eigenvector. ⎛ ⎞ ⎛ ⎞ b 2 { ⎝b 2 ⎠ ∣ b 2 ∈ C} ⎝ 1 1⎠ 0 0 For λ 2 = 1 the system is −2 · b 1 − 2 · b 2 = 0 −2 · b 1 − 2 · b 2 = 0 0 · b 3 = 0 leading to this. ⎛ { ⎝ −b ⎞ ⎛ 2 b 2 ⎠ + ⎝ 0 ⎞ ⎛ 0 ⎠ ∣ b 2 , b 3 ∈ C} ⎝ −1 ⎞ ⎛ 1 ⎠ , ⎝ 0 ⎞ 0⎠ 0 b 3 0 1 (b) The characteristic equation is −x 1 0 0 = 0 −x 1 ∣ 4 −17 8 − x∣ = −x3 + 8x 2 − 17x + 4 = −1 · (x − 4)(x 2 − 4x + 1) and the eigenvalues are λ 1 = 4 and (by using the quadratic equation) λ 2 = 2 + √ 3 and λ 3 = 2 − √ 3. To find eigenvectors, consider this system. −x · b 1 + b 2 = 0 −x · b 2 + b 3 = 0 4 · b 1 − 17 · b 2 + (8 − x) · b 3 = 0 Substituting x = λ 1 = 4 gives the system −4 · b 1 + b 2 = 0 −4 · b 2 + b 3 = 0 4 · b 1 − 17 · b 2 + 4 · b 3 = 0 −4 · b 1 + b 2 = 0 ρ 1+ρ 3 −→ −4 · b 2 + b 3 = 0 −16 · b 2 + 4 · b 3 = 0 leading to this eigenspace and eigenvector. ⎛ V 4 = { ⎝ (1/16) · b ⎞ 3 (1/4) · b 3 ⎠ ∣ b 2 ∈ C} Substituting x = λ 2 = 2 + √ 3 gives the system (−2 − √ 3) · b 1 + b 2 = 0 (−2 − √ 3) · b 2 + b 3 = 0 4 · b 1 − 17 · b 2 + (6 − √ 3) · b 3 = 0 b 3 −4 · b 1 + b 2 = 0 −4ρ 2+ρ 3 −→ −4 · b 2 + b 3 = 0 0 = 0 ⎛ ⎞ ⎝ 1 4 ⎠ 16 (−4/(−2− √ 3))ρ 1 +ρ 3 −→ (−2 − √ 3) · b 1 + b 2 = 0 (−2 − √ 3) · b 2 + b 3 = 0 + (−9 − 4 √ 3) · b 2 + (6 − √ 3) · b 3 = 0 (the middle coefficient in the third equation equals the number (−4/(−2− √ 3))−17; find a common denominator of −2 − √ 3 and then rationalize the denominator by multiplying the top and bottom of the frsction by −2 + √ 3) ((9+4 √ 3)/(−2− √ (−2 − √ 3) · b 1 + b 2 = 0 3))ρ 2+ρ 3 −→ (−2 − √ 3) · b 2 + b 3 = 0 0 = 0
Answers to Exercises 189 which leads to this eigenspace and eigenvector. ⎛ V √ 2+ 3 = { ⎝ (1/(2 + √ ⎞ 3) 2 ) · b 3 (1/(2 + √ 3)) · b 3 ⎠ ∣ b 3 ∈ C} b 3 Finally, substituting x = λ 3 = 2 − √ 3 gives the system (−2 + √ 3) · b 1 + b 2 = 0 (−2 + √ 3) · b 2 + b 3 = 0 4 · b 1 − 17 · b 2 + (6 + √ 3) · b 3 = 0 ⎛ ⎝ (1/(2 + √ ⎞ 3) 2 ) (1/(2 + √ 3)) ⎠ 1 (−4/(−2+ √ 3))ρ 1 +ρ 3 −→ (−2 + √ 3) · b 1 + b 2 = 0 (−2 + √ 3) · b 2 + b 3 = 0 (−9 + 4 √ 3) · b 2 + (6 + √ 3) · b 3 = 0 ((9−4 √ 3)/(−2+ √ (−2 + √ 3) · b 1 + b 2 = 0 3))ρ 2+ρ 3 −→ (−2 + √ 3) · b 2 + b 3 = 0 0 = 0 which gives this eigenspace and eigenvector. ⎛ ⎞ ⎛ (1/(−2 + √ ⎞ 3) 2 ) (1/(2 + √ 3) 2 ) · b 3 V √ 2− 3 = { ⎝ (1/(2 − √ 3)) · b 3 ⎠ ∣ b 3 ∈ C} b 3 ⎝ (1/(−2 + √ 3)) 1 Five.II.3.25 With respect to the natural basis B = 〈1, x, x 2 〉 the matrix representation is this. ⎛ ⎞ Rep B,B (t) = ⎝ 5 6 2 0 −1 −8⎠ 1 0 −2 Thus the characteristic ⎛ equation 0 = ⎝ 5 − x 6 2 ⎞ 0 −1 − x −8 ⎠ = (5 − x)(−1 − x)(−2 − x) − 48 − 2 · (−1 − x) 1 0 −2 − x is 0 = −x 3 + 2x 2 + 15x − 36 = −1 · (x + 4)(x − 3) 2 . To find the associated eigenvectors, consider this system. (5 − x) · b 1 + 6 · b 2 + 2 · b 3 = 0 (−1 − x) · b 2 − 8 · b 3 = 0 b 1 + (−2 − x) · b 3 = 0 Plugging in x = λ 1 = 4 gives b 1 + 6 · b 2 + 2 · b 3 = 0 b 1 + 6 · b 2 + 2 · b 3 = 0 b 1 + 6 · b 2 + 2 · b 3 = 0 −ρ 1 +ρ 2 −ρ 1 +ρ 2 −5 · b 2 − 8 · b 3 = 0 −→ −5 · b 2 − 8 · b 3 = 0 −→ −5 · b 2 − 8 · b 3 = 0 b 1 − 6 · b 3 = 0 −6 · b 2 − 8 · b 3 = 0 −6 · b 2 − 8 · b 3 = 0 ( ) ( ) ( ) ( ) 0 0 2 3 −1 0 −2 1 Five.II.3.26 λ = 1, and , λ = −2, , λ = −1, 0 1 1 0 1 0 1 0 Five.II.3.27 Fix the natural basis B = 〈1, x, x 2 , x 3 〉. The map’s action is 1 ↦→ 0, x ↦→ 1, x 2 ↦→ 2x, and x 3 ↦→ 3x 2 and its representation is easy to compute. ⎛ ⎞ 0 1 0 0 T = Rep B,B (d/dx) = ⎜0 0 2 0 ⎟ ⎝0 0 0 3⎠ 0 0 0 0 We find the eigenvalues with this computation. −x 1 0 0 0 = |T − xI| = 0 −x 2 0 0 0 −x 3 = x 4 ∣ 0 0 0 −x∣ Thus the map ⎛ has the single ⎞ eigenvalue ⎛ ⎞ λ = 0. ⎛ To ⎞ find the associated eigenvectors, we solve 0 1 0 0 b 1 b 1 ⎜0 0 2 0 ⎟ ⎜b 2 ⎟ ⎝0 0 0 3⎠ ⎝b 3 ⎠ = 0 · ⎜b 2 ⎟ ⎝b 3 ⎠ =⇒ b 2 = 0, b 3 = 0, b 4 = 0 0 0 0 0 b 4 b 4 B,B B B B,B ⎠
- Page 140 and 141: 138 Linear Algebra, by Hefferon the
- Page 142 and 143: 140 Linear Algebra, by Hefferon and
- Page 144 and 145: 142 Linear Algebra, by Hefferon and
- Page 146 and 147: 144 Linear Algebra, by Hefferon Pro
- Page 148 and 149: 146 Linear Algebra, by Hefferon 5 T
- Page 150 and 151: 148 Linear Algebra, by Hefferon Top
- Page 152 and 153: 150 Linear Algebra, by Hefferon 4 R
- Page 154 and 155: 152 Linear Algebra, by Hefferon > 0
- Page 156 and 157: 154 Linear Algebra, by Hefferon 0.5
- Page 158 and 159: 156 Linear Algebra, by Hefferon n =
- Page 160 and 161: 158 Linear Algebra, by Hefferon Top
- Page 162 and 163: 160 Linear Algebra, by Hefferon and
- Page 164 and 165: 162 Linear Algebra, by Hefferon ∣
- Page 166 and 167: 164 Linear Algebra, by Hefferon Fou
- Page 168 and 169: 166 Linear Algebra, by Hefferon Fou
- Page 170 and 171: 168 Linear Algebra, by Hefferon The
- Page 172 and 173: 170 Linear Algebra, by Hefferon Fou
- Page 174 and 175: 172 Linear Algebra, by Hefferon ∣
- Page 176 and 177: 174 Linear Algebra, by Hefferon whe
- Page 178 and 179: 176 Linear Algebra, by Hefferon 0.2
- Page 180 and 181: 178 Linear Algebra, by Hefferon Als
- Page 182 and 183: 180 Linear Algebra, by Hefferon Fiv
- Page 184 and 185: 182 Linear Algebra, by Hefferon Res
- Page 186 and 187: 184 Linear Algebra, by Hefferon The
- Page 188 and 189: 186 Linear Algebra, by Hefferon (as
- Page 192 and 193: 190 Linear Algebra, by Hefferon to
- Page 194 and 195: 192 Linear Algebra, by Hefferon Fiv
- Page 196 and 197: 194 Linear Algebra, by Hefferon and
- Page 198 and 199: 196 Linear Algebra, by Hefferon tha
- Page 200 and 201: 198 Linear Algebra, by Hefferon Aga
- Page 202 and 203: 200 Linear Algebra, by Hefferon Bec
- Page 204 and 205: 202 Linear Algebra, by Hefferon (c)
- Page 206 and 207: 204 Linear Algebra, by Hefferon Fiv
- Page 208 and 209: 206 Linear Algebra, by Hefferon For
- Page 210 and 211: 208 Linear Algebra, by Hefferon Fiv
- Page 212 and 213: 210 Linear Algebra, by Hefferon Fiv
<strong>Answers</strong> to <strong>Exercises</strong> 189<br />
which leads to this eigenspace and eigenvector.<br />
⎛<br />
V √<br />
2+ 3<br />
= { ⎝ (1/(2 + √ ⎞<br />
3) 2 ) · b 3<br />
(1/(2 + √ 3)) · b 3<br />
⎠ ∣ b 3 ∈ C}<br />
b 3<br />
Finally, substituting x = λ 3 = 2 − √ 3 gives the system<br />
(−2 + √ 3) · b 1 + b 2 = 0<br />
(−2 + √ 3) · b 2 + b 3 = 0<br />
4 · b 1 − 17 · b 2 + (6 + √ 3) · b 3 = 0<br />
⎛<br />
⎝ (1/(2 + √ ⎞<br />
3) 2 )<br />
(1/(2 + √ 3)) ⎠<br />
1<br />
(−4/(−2+ √ 3))ρ 1 +ρ 3<br />
−→<br />
(−2 + √ 3) · b 1 + b 2 = 0<br />
(−2 + √ 3) · b 2 + b 3 = 0<br />
(−9 + 4 √ 3) · b 2 + (6 + √ 3) · b 3 = 0<br />
((9−4 √ 3)/(−2+ √ (−2 + √ 3) · b 1 + b 2 = 0<br />
3))ρ 2+ρ 3<br />
−→ (−2 + √ 3) · b 2 + b 3 = 0<br />
0 = 0<br />
which gives this eigenspace and eigenvector.<br />
⎛<br />
⎞<br />
⎛<br />
(1/(−2 + √ ⎞<br />
3) 2 )<br />
(1/(2 + √ 3) 2 ) · b 3<br />
V √<br />
2− 3<br />
= { ⎝ (1/(2 − √ 3)) · b 3<br />
⎠ ∣ b 3 ∈ C}<br />
b 3<br />
⎝<br />
(1/(−2 + √ 3))<br />
1<br />
Five.II.3.25 With respect to the natural basis B = 〈1, x, x 2 〉 the matrix representation is this.<br />
⎛<br />
⎞<br />
Rep B,B (t) = ⎝ 5 6 2<br />
0 −1 −8⎠<br />
1 0 −2<br />
Thus the characteristic<br />
⎛<br />
equation<br />
0 = ⎝ 5 − x 6 2 ⎞<br />
0 −1 − x −8 ⎠ = (5 − x)(−1 − x)(−2 − x) − 48 − 2 · (−1 − x)<br />
1 0 −2 − x<br />
is 0 = −x 3 + 2x 2 + 15x − 36 = −1 · (x + 4)(x − 3) 2 . To find the associated eigenvectors, consider this<br />
system.<br />
(5 − x) · b 1 + 6 · b 2 + 2 · b 3 = 0<br />
(−1 − x) · b 2 − 8 · b 3 = 0<br />
b 1 + (−2 − x) · b 3 = 0<br />
Plugging in x = λ 1 = 4 gives<br />
b 1 + 6 · b 2 + 2 · b 3 = 0 b 1 + 6 · b 2 + 2 · b 3 = 0 b 1 + 6 · b 2 + 2 · b 3 = 0<br />
−ρ 1 +ρ 2<br />
−ρ 1 +ρ 2<br />
−5 · b 2 − 8 · b 3 = 0 −→ −5 · b 2 − 8 · b 3 = 0 −→ −5 · b 2 − 8 · b 3 = 0<br />
b 1 − 6 · b 3 = 0<br />
−6 · b 2 − 8 · b 3 = 0<br />
−6 · b 2 − 8 · b 3 = 0<br />
( ) ( ) ( ) ( )<br />
0 0 2 3<br />
−1 0<br />
−2 1<br />
Five.II.3.26 λ = 1, and , λ = −2, , λ = −1,<br />
0 1 1 0<br />
1 0<br />
1 0<br />
Five.II.3.27 Fix the natural basis B = 〈1, x, x 2 , x 3 〉. The map’s action is 1 ↦→ 0, x ↦→ 1, x 2 ↦→ 2x, and<br />
x 3 ↦→ 3x 2 and its representation is easy to compute.<br />
⎛ ⎞<br />
0 1 0 0<br />
T = Rep B,B (d/dx) = ⎜0 0 2 0<br />
⎟<br />
⎝0 0 0 3⎠<br />
0 0 0 0<br />
We find the eigenvalues with this computation.<br />
−x 1 0 0<br />
0 = |T − xI| =<br />
0 −x 2 0<br />
0 0 −x 3<br />
= x 4<br />
∣ 0 0 0 −x∣<br />
Thus the map<br />
⎛<br />
has the single<br />
⎞<br />
eigenvalue<br />
⎛ ⎞<br />
λ = 0.<br />
⎛<br />
To<br />
⎞<br />
find the associated eigenvectors, we solve<br />
0 1 0 0 b 1<br />
b 1<br />
⎜0 0 2 0<br />
⎟ ⎜b 2<br />
⎟<br />
⎝0 0 0 3⎠<br />
⎝b 3<br />
⎠ = 0 · ⎜b 2<br />
⎟<br />
⎝b 3<br />
⎠ =⇒ b 2 = 0, b 3 = 0, b 4 = 0<br />
0 0 0 0 b 4 b 4<br />
B,B<br />
B<br />
B<br />
B,B<br />
⎠