Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
184 <strong>Linear</strong> <strong>Algebra</strong>, by Hefferon<br />
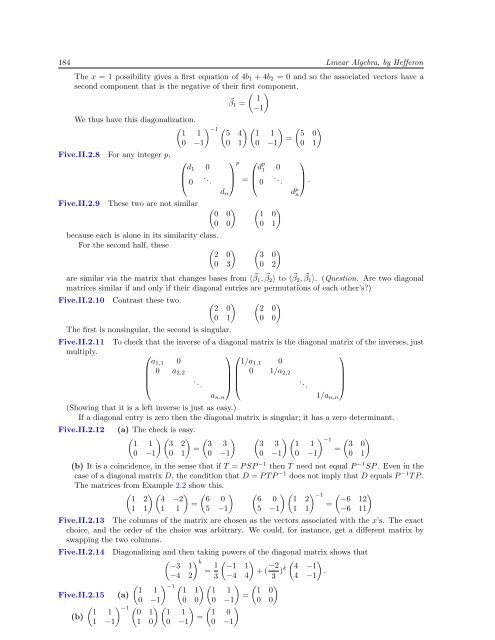
The x = 1 possibility gives a first equation of 4b 1 + 4b 2 = 0 and so the associated vectors have a<br />
second component that is the negative of their first component.<br />
(<br />
1 ⃗β 1 =<br />
−1)<br />
We thus have this diagonalization.<br />
( ) −1 ( ) ( )<br />
1 1 5 4 1 1<br />
=<br />
0 −1 0 1 0 −1<br />
Five.II.2.8 For any integer p,<br />
⎛<br />
⎞<br />
d 1 0<br />
⎜<br />
⎝<br />
.<br />
0 ..<br />
⎟<br />
⎠<br />
d n<br />
p<br />
( )<br />
5 0<br />
0 1<br />
⎛<br />
d p ⎞<br />
1 0<br />
⎜<br />
= ⎝<br />
.<br />
0 ..<br />
⎟<br />
⎠ .<br />
d p n<br />
Five.II.2.9<br />
These two are not similar<br />
(<br />
0<br />
)<br />
0<br />
(<br />
1<br />
)<br />
0<br />
0 0 0 1<br />
because each is alone in its similarity class.<br />
For the second half, these ( ) ( )<br />
2 0 3 0<br />
0 3 0 2<br />
are similar via the matrix that changes bases from 〈 ⃗ β 1 , ⃗ β 2 〉 to 〈 ⃗ β 2 , ⃗ β 1 〉. (Question. Are two diagonal<br />
matrices similar if and only if their diagonal entries are permutations of each other’s?)<br />
Five.II.2.10 Contrast these two. (<br />
2<br />
)<br />
0<br />
(<br />
2<br />
)<br />
0<br />
0 1 0 0<br />
The first is nonsingular, the second is singular.<br />
Five.II.2.11<br />
multiply.<br />
To check that the inverse of a diagonal matrix is the diagonal matrix of the inverses, just<br />
⎛<br />
⎞ ⎛<br />
⎞<br />
a 1,1 0<br />
1/a 1,1 0<br />
0 a 2,2 0 1/a 2,2 ⎜<br />
⎝<br />
. ..<br />
⎟ ⎜<br />
⎠ ⎝<br />
. ..<br />
⎟<br />
⎠<br />
a n,n 1/a n,n<br />
(Showing that it is a left inverse is just as easy.)<br />
If a diagonal entry is zero then the diagonal matrix is singular; it has a zero determinant.<br />
Five.II.2.12<br />
(a) The check is easy.<br />
( ) ( )<br />
1 1 3 2<br />
=<br />
0 −1 0 1<br />
( ) ( ) ( ) −1<br />
3 3 3 3 1 1<br />
=<br />
0 −1 0 −1 0 −1<br />
( )<br />
3 0<br />
0 1<br />
(b) It is a coincidence, in the sense that if T = P SP −1 then T need not equal P −1 SP . Even in the<br />
case of a diagonal matrix D, the condition that D = P T P −1 does not imply that D equals P −1 T P .<br />
The matrices from Example 2.2 show this.<br />
(<br />
1 2<br />
1 1<br />
) ( )<br />
4 −2<br />
=<br />
1 1<br />
(<br />
6 0<br />
5 −1<br />
) ( ) ( ) −1<br />
6 0 1 2<br />
=<br />
5 −1 1 1<br />
(<br />
−6<br />
)<br />
12<br />
−6 11<br />
Five.II.2.13 The columns of the matrix are chosen as the vectors associated with the x’s. The exact<br />
choice, and the order of the choice was arbitrary. We could, for instance, get a different matrix by<br />
swapping the two columns.<br />
Five.II.2.14 Diagonalizing and then taking powers of the diagonal matrix shows that<br />
( k<br />
−3 1<br />
=<br />
−4 2) 1 ( )<br />
−1 1<br />
+ ( −2 ( )<br />
4 −1<br />
3 −4 4 3 )k .<br />
4 −1<br />
( ) −1 ( ) ( ) ( )<br />
1 1 1 1 1 1 1 0<br />
Five.II.2.15 (a)<br />
=<br />
0 −1 0 0 0 −1 0 0<br />
( ) −1 ( ) ( ) ( )<br />
1 1 0 1 1 1 1 0<br />
(b)<br />
=<br />
1 −1 1 0 0 −1 0 −1